Description: Define plus infinity.
Note that the definition is arbitrary, requiring
only that 
be a set not in  and
different from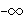 
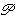(df-mnf 8207). We use    to
make it independent of the
construction of , and Cantor's Theorem will show that it is
different from any member of and therefore . See pnfnre 8211
and mnfnre 8212, and we'll also be able to prove  .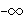
A simpler possibility is to define as a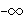nd
as
  , but that approach requires the Axiom of
Regularity to show
that and
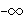are different
from each other and from all
members of .
(Contributed by NM, 13-Oct-2005.)
(New usage is discouraged.) |
