Proof of Theorem phiexg
| Step | Hyp | Ref
| Expression |
|---|
| 1 | | dfphi2 4570 |
. 2
 Phi Phi      Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k       k k   ∼ Nn ∼ Nn  k k      k k  |
| 2 | | addcexlem 4383 |
. . . . . . 7
  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c 
  |
| 3 | | 1cex 4143 |
. . . . . . . . 9
 1c
1c 
 |
| 4 | 3 | pw1ex 4304 |
. . . . . . . 8
  1
1c 1
1c 
 |
| 5 | 4 | pw1ex 4304 |
. . . . . . 7
  1 1  1
1c 1
1c 
 |
| 6 | 2, 5 | imakex 4301 |
. . . . . 6
   Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c 
 |
| 7 | 6 | imagekex 4313 |
. . . . 5
 Imagek
Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c 
 |
| 8 | | nncex 4397 |
. . . . . 6
 Nn Nn   |
| 9 | | vvex 4110 |
. . . . . 6
    |
| 10 | 8, 9 | xpkex 4290 |
. . . . 5
  Nn Nn  k k  
  |
| 11 | 7, 10 | inex 4106 |
. . . 4
  Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k      |
| 12 | | idkex 4315 |
. . . . 5
  k k
  |
| 13 | 8 | complex 4105 |
. . . . . 6
 ∼ Nn ∼ Nn   |
| 14 | 13, 9 | xpkex 4290 |
. . . . 5
  ∼ Nn ∼ Nn  k k  
  |
| 15 | 12, 14 | inex 4106 |
. . . 4
   k k   ∼ Nn ∼ Nn  k k   
  |
| 16 | 11, 15 | unex 4107 |
. . 3
   Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k       k k   ∼ Nn ∼ Nn  k k       |
| 17 | | imakexg 4300 |
. . 3
     Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k       k k   ∼ Nn ∼ Nn  k k          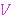      Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k       k k   ∼ Nn ∼ Nn  k k      k k  
  |
| 18 | 16, 17 | mpan 651 |
. 2
    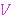     Imagek Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c   Nn Nn  k k       k k   ∼ Nn ∼ Nn  k k      k k  
  |
| 19 | 1, 18 | syl5eqel 2437 |
1
    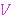  Phi
Phi     |