| Step | Hyp | Ref
| Expression |
|---|
| 1 | | dffun2 5120 |
. . . 4
   Swap Swap           Swap Swap    Swap Swap
    
   |
| 2 | | opeq 4620 |
. . . . . . . 8
    Proj1 Proj1   Proj2 Proj2   |
| 3 | 2 | breq2i 4648 |
. . . . . . 7
   Swap Swap    Swap Swap
 Proj1 Proj1   Proj2 Proj2    |
| 4 | | vex 2863 |
. . . . . . . . 9
    |
| 5 | 4 | proj1ex 4594 |
. . . . . . . 8
 Proj1 Proj1    |
| 6 | 4 | proj2ex 4595 |
. . . . . . . 8
 Proj2 Proj2    |
| 7 | 5, 6 | brswap2 4861 |
. . . . . . 7
   Swap Swap  Proj1 Proj1   Proj2 Proj2       Proj2 Proj2   Proj1 Proj1    |
| 8 | 3, 7 | bitri 240 |
. . . . . 6
   Swap Swap      Proj2 Proj2   Proj1 Proj1    |
| 9 | | opeq 4620 |
. . . . . . . 8
    Proj1 Proj1   Proj2 Proj2   |
| 10 | 9 | breq2i 4648 |
. . . . . . 7
   Swap Swap    Swap Swap
 Proj1 Proj1   Proj2 Proj2    |
| 11 | | vex 2863 |
. . . . . . . . 9
    |
| 12 | 11 | proj1ex 4594 |
. . . . . . . 8
 Proj1 Proj1    |
| 13 | 11 | proj2ex 4595 |
. . . . . . . 8
 Proj2 Proj2    |
| 14 | 12, 13 | brswap2 4861 |
. . . . . . 7
   Swap Swap  Proj1 Proj1   Proj2 Proj2       Proj2 Proj2   Proj1 Proj1    |
| 15 | 10, 14 | bitri 240 |
. . . . . 6
   Swap Swap      Proj2 Proj2   Proj1 Proj1    |
| 16 | | eqtr2 2371 |
. . . . . . 7
      Proj2 Proj2   Proj1 Proj1       Proj2 Proj2   Proj1 Proj1      Proj2 Proj2   Proj1 Proj1     Proj2 Proj2
  Proj1
Proj1    |
| 17 | | ancom 437 |
. . . . . . . 8
   Proj2 Proj2   Proj2 Proj2   Proj1 Proj1
  Proj1
Proj1     Proj1 Proj1
  Proj1
Proj1   Proj2
Proj2   Proj2
Proj2    |
| 18 | | opth 4603 |
. . . . . . . 8
   Proj2 Proj2
  Proj1
Proj1     Proj2 Proj2   Proj1 Proj1     Proj2 Proj2   Proj2 Proj2   Proj1 Proj1   Proj1 Proj1    |
| 19 | 2, 9 | eqeq12i 2366 |
. . . . . . . . 9
       Proj1 Proj1   Proj2 Proj2     Proj1 Proj1
  Proj2
Proj2    |
| 20 | | opth 4603 |
. . . . . . . . 9
   Proj1 Proj1
  Proj2
Proj2     Proj1 Proj1   Proj2 Proj2     Proj1 Proj1   Proj1 Proj1   Proj2 Proj2   Proj2 Proj2    |
| 21 | 19, 20 | bitri 240 |
. . . . . . . 8
       Proj1 Proj1
  Proj1
Proj1   Proj2
Proj2   Proj2
Proj2    |
| 22 | 17, 18, 21 | 3bitr4i 268 |
. . . . . . 7
   Proj2 Proj2
  Proj1
Proj1     Proj2 Proj2   Proj1 Proj1        |
| 23 | 16, 22 | sylib 188 |
. . . . . 6
      Proj2 Proj2   Proj1 Proj1       Proj2 Proj2   Proj1 Proj1         |
| 24 | 8, 15, 23 | syl2anb 465 |
. . . . 5
    Swap Swap    Swap Swap        |
| 25 | 24 | gen2 1547 |
. . . 4
        Swap Swap    Swap Swap        |
| 26 | 1, 25 | mpgbir 1550 |
. . 3
  Swap
Swap |
| 27 | | eqv 3566 |
. . . 4
   Swap Swap     
   Swap Swap  |
| 28 | | opeq 4620 |
. . . . 5
    Proj1 Proj1   Proj2 Proj2   |
| 29 | | eqid 2353 |
. . . . . . 7
  Proj1 Proj1   Proj2 Proj2     Proj1 Proj1
  Proj2
Proj2   |
| 30 | | vex 2863 |
. . . . . . . . 9
    |
| 31 | 30 | proj2ex 4595 |
. . . . . . . 8
 Proj2 Proj2    |
| 32 | 30 | proj1ex 4594 |
. . . . . . . 8
 Proj1 Proj1    |
| 33 | 31, 32 | brswap2 4861 |
. . . . . . 7
   Proj1 Proj1
  Proj2
Proj2   Swap Swap  Proj2 Proj2   Proj1 Proj1     Proj1 Proj1   Proj2 Proj2     Proj1 Proj1   Proj2 Proj2    |
| 34 | 29, 33 | mpbir 200 |
. . . . . 6
  Proj1 Proj1   Proj2 Proj2   Swap Swap  Proj2 Proj2   Proj1 Proj1   |
| 35 | | breldm 4912 |
. . . . . 6
   Proj1 Proj1
  Proj2
Proj2   Swap Swap  Proj2 Proj2   Proj1 Proj1     Proj1 Proj1   Proj2 Proj2     Swap Swap  |
| 36 | 34, 35 | ax-mp 5 |
. . . . 5
  Proj1 Proj1   Proj2 Proj2     Swap Swap
|
| 37 | 28, 36 | eqeltri 2423 |
. . . 4
    Swap Swap |
| 38 | 27, 37 | mpgbir 1550 |
. . 3
  Swap
Swap   |
| 39 | | df-fn 4791 |
. . 3
  Swap Swap
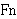     Swap Swap   Swap Swap
    |
| 40 | 26, 38, 39 | mpbir2an 886 |
. 2
 Swap Swap 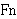  |
| 41 | | cnvswap 5511 |
. . . 4
  Swap
Swap  Swap Swap |
| 42 | 41 | fneq1i 5179 |
. . 3
   Swap Swap 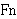   Swap Swap 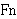   |
| 43 | 40, 42 | mpbir 200 |
. 2
  Swap
Swap 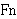  |
| 44 | | dff1o4 5295 |
. 2
  Swap Swap
      Swap Swap
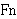    Swap Swap 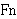    |
| 45 | 40, 43, 44 | mpbir2an 886 |
1
 Swap Swap     |