| Step | Hyp | Ref
| Expression |
|---|
| 1 | | pm5.19 349 |
. . . . 5
    
       |
| 2 | 1 | a1i 10 |
. . . 4
        
    
    |
| 3 | 2 | nrex 2717 |
. . 3
    
   
       |
| 4 | 3 | nex 1555 |
. 2
         
    
   |
| 5 | | bren 6031 |
. . 3
   1 1  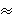         1 1      |
| 6 | | f1odm 5291 |
. . . . . . . . 9
     1 1          1 1   |
| 7 | | vex 2863 |
. . . . . . . . . 10
    |
| 8 | 7 | dmex 5107 |
. . . . . . . . 9
     |
| 9 | 6, 8 | syl6eqelr 2442 |
. . . . . . . 8
     1 1       1 1     |
| 10 | | pw1exb 4327 |
. . . . . . . 8
   1 1         |
| 11 | 9, 10 | sylib 188 |
. . . . . . 7
     1 1          |
| 12 | | nenpw1pwlem2.1 |
. . . . . . . 8
    
              |
| 13 | 12 | nenpw1pwlem1 6085 |
. . . . . . 7
          |
| 14 | 11, 13 | syl 15 |
. . . . . 6
     1 1          |
| 15 | | ssrab2 3352 |
. . . . . . . 8
                   |
| 16 | 12, 15 | eqsstri 3302 |
. . . . . . 7
    |
| 17 | | elpwg 3730 |
. . . . . . 7
        
        |
| 18 | 16, 17 | mpbiri 224 |
. . . . . 6
           |
| 19 | 14, 18 | syl 15 |
. . . . 5
     1 1           |
| 20 | | f1ofo 5294 |
. . . . . . . 8
     1 1         1 1      |
| 21 | | forn 5273 |
. . . . . . . 8
     1 1            |
| 22 | 20, 21 | syl 15 |
. . . . . . 7
     1 1            |
| 23 | 22 | eleq2d 2420 |
. . . . . 6
     1 1       
          |
| 24 | | elrn 4897 |
. . . . . . 7
             |
| 25 | | breldm 4912 |
. . . . . . . . . . . 12
           |
| 26 | 25 | adantl 452 |
. . . . . . . . . . 11
      1 1                |
| 27 | 6 | adantr 451 |
. . . . . . . . . . 11
      1 1               1 1   |
| 28 | 26, 27 | eleqtrd 2429 |
. . . . . . . . . 10
      1 1              1 1   |
| 29 | | elpw1 4145 |
. . . . . . . . . . 11
     1 1             |
| 30 | | breq1 4643 |
. . . . . . . . . . . . . . . 16
                    |
| 31 | 30 | 3anbi2d 1257 |
. . . . . . . . . . . . . . 15
             1 1                   1 1            
     |
| 32 | | id 19 |
. . . . . . . . . . . . . . . . . . 19
          |
| 33 | | sneq 3745 |
. . . . . . . . . . . . . . . . . . . 20
              |
| 34 | 33 | fveq2d 5333 |
. . . . . . . . . . . . . . . . . . 19
             
        |
| 35 | 32, 34 | eleq12d 2421 |
. . . . . . . . . . . . . . . . . 18
        
         
         |
| 36 | 35 | notbid 285 |
. . . . . . . . . . . . . . . . 17
        
                     |
| 37 | 36, 12 | elrab2 2997 |
. . . . . . . . . . . . . . . 16
             
         |
| 38 | | simp3 957 |
. . . . . . . . . . . . . . . . . 18
      1 1                     |
| 39 | 38 | biantrurd 494 |
. . . . . . . . . . . . . . . . 17
      1 1                                    
          |
| 40 | | simp2 956 |
. . . . . . . . . . . . . . . . . . . 20
      1 1                       |
| 41 | | f1ofn 5289 |
. . . . . . . . . . . . . . . . . . . . . 22
     1 1       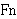  1 1   |
| 42 | 41 | 3ad2ant1 976 |
. . . . . . . . . . . . . . . . . . . . 21
      1 1                  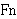  1 1   |
| 43 | | snelpw1 4147 |
. . . . . . . . . . . . . . . . . . . . . . 23
       1 1       |
| 44 | 43 | biimpri 197 |
. . . . . . . . . . . . . . . . . . . . . 22
           1 1   |
| 45 | 44 | 3ad2ant3 978 |
. . . . . . . . . . . . . . . . . . . . 21
      1 1                      1 1   |
| 46 | | fnbrfvb 5359 |
. . . . . . . . . . . . . . . . . . . . 21
    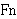  1 1        1 1             

       |
| 47 | 42, 45, 46 | syl2anc 642 |
. . . . . . . . . . . . . . . . . . . 20
      1 1                          

       |
| 48 | 40, 47 | mpbird 223 |
. . . . . . . . . . . . . . . . . . 19
      1 1                           |
| 49 | 48 | eleq2d 2420 |
. . . . . . . . . . . . . . . . . 18
      1 1                                 |
| 50 | 49 | notbid 285 |
. . . . . . . . . . . . . . . . 17
      1 1                                   |
| 51 | 39, 50 | bitr3d 246 |
. . . . . . . . . . . . . . . 16
      1 1                         
               |
| 52 | 37, 51 | syl5bb 248 |
. . . . . . . . . . . . . . 15
      1 1                            |
| 53 | 31, 52 | syl6bi 219 |
. . . . . . . . . . . . . 14
             1 1                 
         |
| 54 | 53 | com12 27 |
. . . . . . . . . . . . 13
      1 1           
                      |
| 55 | 54 | 3expa 1151 |
. . . . . . . . . . . 12
       1 1                  
                |
| 56 | 55 | reximdva 2727 |
. . . . . . . . . . 11
      1 1                                      |
| 57 | 29, 56 | syl5bi 208 |
. . . . . . . . . 10
      1 1               1 1        
    
     |
| 58 | 28, 57 | mpd 14 |
. . . . . . . . 9
      1 1                
    
    |
| 59 | 58 | ex 423 |
. . . . . . . 8
     1 1                           |
| 60 | 59 | exlimdv 1636 |
. . . . . . 7
     1 1                             |
| 61 | 24, 60 | syl5bi 208 |
. . . . . 6
     1 1       
                    |
| 62 | 23, 61 | sylbird 226 |
. . . . 5
     1 1       
                    |
| 63 | 19, 62 | mpd 14 |
. . . 4
     1 1                     |
| 64 | 63 | eximi 1576 |
. . 3
       1 1                       |
| 65 | 5, 64 | sylbi 187 |
. 2
   1 1             
    
    |
| 66 | 4, 65 | mto 167 |
1
   1 1     |