Theorem dflec3 6222

Description: Another potential definition of cardinal inequality. (Contributed by SF, 23-Mar-2015.)

Assertion

Ref	Expression
dflec3	|- ((M e. NC /\ N e. NC ) -> (M <_c N <-> E.a e. M E.b e. N E.f f:a-1-1->b))

Distinct variable groups:   M,a   N,a,b   f,a,b

Allowed substitution hints:   M(f,b)   N(f)

Proof of Theorem dflec3

Dummy variables x y c are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		elncs 6120	. . . 4 |- (M e. NC <-> E.x M = Nc x)
2		elncs 6120	. . . 4 |- (N e. NC <-> E.y N = Nc y)
3	1, 2	anbi12i 678	. . 3 |- ((M e. NC /\ N e. NC ) <-> (E.x M = Nc x /\ E.y N = Nc y))
4		eeanv 1913	. . 3 |- (E.xE.y(M = Nc x /\ N = Nc y) <-> (E.x M = Nc x /\ E.y N = Nc y))
5	3, 4	bitr4i 243	. 2 |- ((M e. NC /\ N e. NC ) <-> E.xE.y(M = Nc x /\ N = Nc y))
6		ncex 6118	. . . . . 6 |- Nc x e. _V
7		ncex 6118	. . . . . 6 |- Nc y e. _V
8	6, 7	brlec 6114	. . . . 5 |- ( Nc x <_c Nc y <-> E.c e. Nc xE.b e. Nc yc C_ b)
9		rexcom 2773	. . . . . 6 |- (E.c e. Nc xE.b e. Nc yc C_ b <-> E.b e. Nc yE.c e. Nc xc C_ b)
10		f1oi 5321	. . . . . . . . . . . . 13 |- ( _I |` c):c-1-1-onto->c
11		f1of1 5287	. . . . . . . . . . . . 13 |- (( _I |` c):c-1-1-onto->c -> ( _I |` c):c-1-1->c)
12	10, 11	ax-mp 5	. . . . . . . . . . . 12 |- ( _I |` c):c-1-1->c
13		f1ss 5263	. . . . . . . . . . . 12 |- ((( _I |` c):c-1-1->c /\ c C_ b) -> ( _I |` c):c-1-1->b)
14	12, 13	mpan 651	. . . . . . . . . . 11 |- (c C_ b -> ( _I |` c):c-1-1->b)
15		idex 5505	. . . . . . . . . . . . 13 |- _I e. _V
16		vex 2863	. . . . . . . . . . . . 13 |- c e. _V
17	15, 16	resex 5118	. . . . . . . . . . . 12 |- ( _I |` c) e. _V
18		f1eq1 5254	. . . . . . . . . . . 12 |- (f = ( _I |` c) -> (f:c-1-1->b <-> ( _I |` c):c-1-1->b))
19	17, 18	spcev 2947	. . . . . . . . . . 11 |- (( _I |` c):c-1-1->b -> E.f f:c-1-1->b)
20	14, 19	syl 15	. . . . . . . . . 10 |- (c C_ b -> E.f f:c-1-1->b)
21		f1eq2 5255	. . . . . . . . . . . 12 |- (a = c -> (f:a-1-1->b <-> f:c-1-1->b))
22	21	exbidv 1626	. . . . . . . . . . 11 |- (a = c -> (E.f f:a-1-1->b <-> E.f f:c-1-1->b))
23	22	rspcev 2956	. . . . . . . . . 10 |- ((c e. Nc x /\ E.f f:c-1-1->b) -> E.a e. Nc xE.f f:a-1-1->b)
24	20, 23	sylan2 460	. . . . . . . . 9 |- ((c e. Nc x /\ c C_ b) -> E.a e. Nc xE.f f:a-1-1->b)
25	24	rexlimiva 2734	. . . . . . . 8 |- (E.c e. Nc xc C_ b -> E.a e. Nc xE.f f:a-1-1->b)
26		vex 2863	. . . . . . . . . . . . . . . 16 |- a e. _V
27	26	eqnc 6128	. . . . . . . . . . . . . . 15 |- ( Nc a = Nc x <-> a ~~ x)
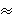28		elnc 6126	. . . . . . . . . . . . . . 15 |- (a e. Nc x <-> a ~~ x)
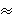29	27, 28	bitr4i 243	. . . . . . . . . . . . . 14 |- ( Nc a = Nc x <-> a e. Nc x)
30		f1f1orn 5298	. . . . . . . . . . . . . . . . . 18 |- (f:a-1-1->b -> f:a-1-1-onto->ran f)
31		vex 2863	. . . . . . . . . . . . . . . . . . 19 |- f e. _V
32	31	f1oen 6034	. . . . . . . . . . . . . . . . . 18 |- (f:a-1-1-onto->ran f -> a ~~ ran f)
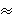33	30, 32	syl 15	. . . . . . . . . . . . . . . . 17 |- (f:a-1-1->b -> a ~~ ran f)
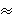34		ensym 6038	. . . . . . . . . . . . . . . . 17 |- (a ~~ ran f <-> ran f ~~ a)
35	33, 34	sylib 188	. . . . . . . . . . . . . . . 16 |- (f:a-1-1->b -> ran f ~~ a)
36		elnc 6126	. . . . . . . . . . . . . . . 16 |- (ran f e. Nc a <-> ran f ~~ a)
37	35, 36	sylibr 203	. . . . . . . . . . . . . . 15 |- (f:a-1-1->b -> ran f e. Nc a)
38		eleq2 2414	. . . . . . . . . . . . . . 15 |- ( Nc a = Nc x -> (ran f e. Nc a <-> ran f e. Nc x))
39	37, 38	syl5ib 210	. . . . . . . . . . . . . 14 |- ( Nc a = Nc x -> (f:a-1-1->b -> ran f e. Nc x))
40	29, 39	sylbir 204	. . . . . . . . . . . . 13 |- (a e. Nc x -> (f:a-1-1->b -> ran f e. Nc x))
41	40	imp 418	. . . . . . . . . . . 12 |- ((a e. Nc x /\ f:a-1-1->b) -> ran f e. Nc x)
42		f1f 5259	. . . . . . . . . . . . . 14 |- (f:a-1-1->b -> f:a-->b)
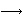43		frn 5229	. . . . . . . . . . . . . 14 |- (f:a-->b -> ran f C_ b)
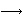
44	42, 43	syl 15	. . . . . . . . . . . . 13 |- (f:a-1-1->b -> ran f C_ b)
45	44	adantl 452	. . . . . . . . . . . 12 |- ((a e. Nc x /\ f:a-1-1->b) -> ran f C_ b)
46		sseq1 3293	. . . . . . . . . . . . 13 |- (c = ran f -> (c C_ b <-> ran f C_ b))
47	46	rspcev 2956	. . . . . . . . . . . 12 |- ((ran f e. Nc x /\ ran f C_ b) -> E.c e. Nc xc C_ b)
48	41, 45, 47	syl2anc 642	. . . . . . . . . . 11 |- ((a e. Nc x /\ f:a-1-1->b) -> E.c e. Nc xc C_ b)
49	48	ex 423	. . . . . . . . . 10 |- (a e. Nc x -> (f:a-1-1->b -> E.c e. Nc xc C_ b))
50	49	exlimdv 1636	. . . . . . . . 9 |- (a e. Nc x -> (E.f f:a-1-1->b -> E.c e. Nc xc C_ b))
51	50	rexlimiv 2733	. . . . . . . 8 |- (E.a e. Nc xE.f f:a-1-1->b -> E.c e. Nc xc C_ b)
52	25, 51	impbii 180	. . . . . . 7 |- (E.c e. Nc xc C_ b <-> E.a e. Nc xE.f f:a-1-1->b)
53	52	rexbii 2640	. . . . . 6 |- (E.b e. Nc yE.c e. Nc xc C_ b <-> E.b e. Nc yE.a e. Nc xE.f f:a-1-1->b)
54	9, 53	bitri 240	. . . . 5 |- (E.c e. Nc xE.b e. Nc yc C_ b <-> E.b e. Nc yE.a e. Nc xE.f f:a-1-1->b)
55		rexcom 2773	. . . . 5 |- (E.b e. Nc yE.a e. Nc xE.f f:a-1-1->b <-> E.a e. Nc xE.b e. Nc yE.f f:a-1-1->b)
56	8, 54, 55	3bitri 262	. . . 4 |- ( Nc x <_c Nc y <-> E.a e. Nc xE.b e. Nc yE.f f:a-1-1->b)
57		breq12 4645	. . . . 5 |- ((M = Nc x /\ N = Nc y) -> (M <_c N <-> Nc x <_c Nc y))
58		simpl 443	. . . . . 6 |- ((M = Nc x /\ N = Nc y) -> M = Nc x)
59		rexeq 2809	. . . . . . 7 |- (N = Nc y -> (E.b e. N E.f f:a-1-1->b <-> E.b e. Nc yE.f f:a-1-1->b))
60	59	adantl 452	. . . . . 6 |- ((M = Nc x /\ N = Nc y) -> (E.b e. N E.f f:a-1-1->b <-> E.b e. Nc yE.f f:a-1-1->b))
61	58, 60	rexeqbidv 2821	. . . . 5 |- ((M = Nc x /\ N = Nc y) -> (E.a e. M E.b e. N E.f f:a-1-1->b <-> E.a e. Nc xE.b e. Nc yE.f f:a-1-1->b))
62	57, 61	bibi12d 312	. . . 4 |- ((M = Nc x /\ N = Nc y) -> ((M <_c N <-> E.a e. M E.b e. N E.f f:a-1-1->b) <-> ( Nc x <_c Nc y <-> E.a e. Nc xE.b e. Nc yE.f f:a-1-1->b)))
63	56, 62	mpbiri 224	. . 3 |- ((M = Nc x /\ N = Nc y) -> (M <_c N <-> E.a e. M E.b e. N E.f f:a-1-1->b))
64	63	exlimivv 1635	. 2 |- (E.xE.y(M = Nc x /\ N = Nc y) -> (M <_c N <-> E.a e. M E.b e. N E.f f:a-1-1->b))
65	5, 64	sylbi 187	1 |- ((M e. NC /\ N e. NC ) -> (M <_c N <-> E.a e. M E.b e. N E.f f:a-1-1->b))

Syntax hints:   -> wi 4   <-> wb 176   /\ wa 358  E.wex 1541   = wceq 1642   e. wcel 1710  E.wrex 2616   C_ wss 3258   class class class wbr 4640   _I cid 4764  ran crn 4774   |` cres 4775  -->wf 4778  -1-1->wf1 4779  -1-1-onto->wf1o 4781   ~~ cen 6029   NC cncs 6089   <__c clec 6090   Nc cnc 6092

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1546  ax-5 1557  ax-17 1616  ax-9 1654  ax-8 1675  ax-13 1712  ax-14 1714  ax-6 1729  ax-7 1734  ax-11 1746  ax-12 1925  ax-ext 2334  ax-nin 4079  ax-xp 4080  ax-cnv 4081  ax-1c 4082  ax-sset 4083  ax-si 4084  ax-ins2 4085  ax-ins3 4086  ax-typlower 4087  ax-sn 4088

This theorem depends on definitions:  df-bi 177  df-or 359  df-an 360  df-3or 935  df-3an 936  df-nan 1288  df-tru 1319  df-ex 1542  df-nf 1545  df-sb 1649  df-eu 2208  df-mo 2209  df-clab 2340  df-cleq 2346  df-clel 2349  df-nfc 2479  df-ne 2519  df-ral 2620  df-rex 2621  df-reu 2622  df-rmo 2623  df-rab 2624  df-v 2862  df-sbc 3048  df-nin 3212  df-compl 3213  df-in 3214  df-un 3215  df-dif 3216  df-symdif 3217  df-ss 3260  df-pss 3262  df-nul 3552  df-if 3664  df-pw 3725  df-sn 3742  df-pr 3743  df-uni 3893  df-int 3928  df-opk 4059  df-1c 4137  df-pw1 4138  df-uni1 4139  df-xpk 4186  df-cnvk 4187  df-ins2k 4188  df-ins3k 4189  df-imak 4190  df-cok 4191  df-p6 4192  df-sik 4193  df-ssetk 4194  df-imagek 4195  df-idk 4196  df-iota 4340  df-0c 4378  df-addc 4379  df-nnc 4380  df-fin 4381  df-lefin 4441  df-ltfin 4442  df-ncfin 4443  df-tfin 4444  df-evenfin 4445  df-oddfin 4446  df-sfin 4447  df-spfin 4448  df-phi 4566  df-op 4567  df-proj1 4568  df-proj2 4569  df-opab 4624  df-br 4641  df-1st 4724  df-swap 4725  df-sset 4726  df-co 4727  df-ima 4728  df-si 4729  df-id 4768  df-xp 4785  df-cnv 4786  df-rn 4787  df-dm 4788  df-res 4789  df-fun 4790  df-fn 4791  df-f 4792  df-f1 4793  df-fo 4794  df-f1o 4795  df-2nd 4798  df-txp 5737  df-ins2 5751  df-ins3 5753  df-image 5755  df-ins4 5757  df-si3 5759  df-funs 5761  df-fns 5763  df-trans 5900  df-sym 5909  df-er 5910  df-ec 5948  df-qs 5952  df-en 6030  df-ncs 6099  df-lec 6100  df-nc 6102

This theorem is referenced by:  nclenc  6223