Definition df-pw 3623

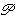Description: Define power class. Definition 5.10 of [TakeutiZaring] p. 17, but we also let it apply to proper classes, i.e. those that are not members of _V. When applied to a set, this produces its power set. A power set of S is the set of all subsets of S, including the empty set and S itself. For example, if A is { 3 , 5 , 7 }, then ~P A is { (/) , { 3 } , { 5 } , { 7 } , { 3 , 5 } , { 3 , 7 } , { 5 , 7 } , { 3 , 5 , 7 } }. We will later introduce the Axiom of Power Sets. Still later we will prove that the size of the power set of a finite set is 2 raised to the power of the size of the set. (Contributed by NM, 5-Aug-1993.)

Assertion

Ref	Expression
df-pw	|- ~P A = { x | x C_ A }

Distinct variable group:   x, A

Detailed syntax breakdown of Definition df-pw

Step	Hyp	Ref	Expression
1		cA	. . 3 class A
2	1	cpw 3621	. 2 class ~P A
3		vx	. . . . 5 setvar x
4	3	cv 1372	. . . 4 class x
5	4, 1	wss 3170	. . 3 wff x C_ A
6	5, 3	cab 2192	. 2 class { x | x C_ A }
7	2, 6	wceq 1373	1 wff ~P A = { x | x C_ A }

This definition is referenced by:  pweq  3624  elpw  3627  nfpw  3634  pwss  3637  pw0  3786  snsspw  3811  pwsnss  3850  vpwex  4231  abssexg  4234  iunpw  4535  iotass  5258  mapex  6754  ssenen  6963  tgvalex  13170  bdcpw  15943