| Step | Hyp | Ref
| Expression |
|---|
| 1 | | breldm 4912 |
. . . . . . . . . 10
           |
| 2 | 1 | adantl 452 |
. . . . . . . . 9
               |
| 3 | | fnfrec.1 |
. . . . . . . . . . 11
   FRec FRec    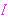  |
| 4 | | fnfrec.2 |
. . . . . . . . . . 11
      Funs Funs  |
| 5 | | fnfrec.3 |
. . . . . . . . . . 11
    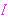     |
| 6 | | fnfrec.4 |
. . . . . . . . . . 11
          |
| 7 | 3, 4, 5, 6 | dmfrec 6317 |
. . . . . . . . . 10
       Nn Nn  |
| 8 | 7 | adantr 451 |
. . . . . . . . 9
             Nn Nn  |
| 9 | 2, 8 | eleqtrd 2429 |
. . . . . . . 8
            Nn Nn  |
| 10 | 9 | adantrr 697 |
. . . . . . 7
                  Nn
Nn  |
| 11 | 3 | frecexg 6313 |
. . . . . . . . . . . 12
    Funs Funs   
  |
| 12 | | fnfreclem1 6318 |
. . . . . . . . . . . 12
        
                       |
| 13 | 4, 11, 12 | 3syl 18 |
. . . . . . . . . . 11
                       
   
  |
| 14 | | breq1 4643 |
. . . . . . . . . . . . . 14
    0c 0c       0c 0c    |
| 15 | | breq1 4643 |
. . . . . . . . . . . . . 14
    0c 0c       0c 0c    |
| 16 | 14, 15 | anbi12d 691 |
. . . . . . . . . . . . 13
    0c 0c              0c 0c   0c 0c     |
| 17 | 16 | imbi1d 308 |
. . . . . . . . . . . 12
    0c 0c                     0c 0c   0c
0c     
    |
| 18 | 17 | 2albidv 1627 |
. . . . . . . . . . 11
    0c 0c                             0c 0c   0c 0c          |
| 19 | | breq1 4643 |
. . . . . . . . . . . . . 14
                |
| 20 | | breq1 4643 |
. . . . . . . . . . . . . 14
                |
| 21 | 19, 20 | anbi12d 691 |
. . . . . . . . . . . . 13
                            |
| 22 | 21 | imbi1d 308 |
. . . . . . . . . . . 12
                                        |
| 23 | 22 | 2albidv 1627 |
. . . . . . . . . . 11
                                                |
| 24 | | breq1 4643 |
. . . . . . . . . . . . . . 15
       1c 1c          1c
1c     |
| 25 | | breq1 4643 |
. . . . . . . . . . . . . . 15
       1c 1c          1c
1c     |
| 26 | 24, 25 | anbi12d 691 |
. . . . . . . . . . . . . 14
       1c 1c                 1c
1c     
 1c
1c      |
| 27 | 26 | imbi1d 308 |
. . . . . . . . . . . . 13
       1c 1c                        1c
1c     
 1c
1c           |
| 28 | 27 | 2albidv 1627 |
. . . . . . . . . . . 12
       1c 1c                                1c
1c     
 1c
1c           |
| 29 | | breq2 4644 |
. . . . . . . . . . . . . . 15
        
 1c
1c       1c 1c     |
| 30 | | breq2 4644 |
. . . . . . . . . . . . . . 15
        
 1c
1c       1c 1c     |
| 31 | 29, 30 | bi2anan9 843 |
. . . . . . . . . . . . . 14
        
        1c 1c       1c 1c         1c 1c  
    1c 1c      |
| 32 | | eqeq12 2365 |
. . . . . . . . . . . . . 14
        
             |
| 33 | 31, 32 | imbi12d 311 |
. . . . . . . . . . . . 13
        
         1c
1c     
 1c
1c               1c 1c       1c 1c           |
| 34 | 33 | cbval2v 2006 |
. . . . . . . . . . . 12
           1c
1c     
 1c
1c                   1c 1c  
    1c 1c          |
| 35 | 28, 34 | syl6bb 252 |
. . . . . . . . . . 11
       1c 1c                                1c
1c     
 1c
1c           |
| 36 | | breq1 4643 |
. . . . . . . . . . . . . 14
                |
| 37 | | breq1 4643 |
. . . . . . . . . . . . . 14
                |
| 38 | 36, 37 | anbi12d 691 |
. . . . . . . . . . . . 13
                            |
| 39 | 38 | imbi1d 308 |
. . . . . . . . . . . 12
                                        |
| 40 | 39 | 2albidv 1627 |
. . . . . . . . . . 11
                                                |
| 41 | 3, 4, 5, 6 | fnfreclem2 6319 |
. . . . . . . . . . . . . . . 16
     0c 0c     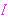   |
| 42 | 41 | imp 418 |
. . . . . . . . . . . . . . 15
     0c 0c     
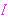  |
| 43 | 42 | adantrr 697 |
. . . . . . . . . . . . . 14
      0c 0c   0c
0c       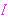  |
| 44 | 3, 4, 5, 6 | fnfreclem2 6319 |
. . . . . . . . . . . . . . . 16
     0c 0c     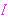   |
| 45 | 44 | imp 418 |
. . . . . . . . . . . . . . 15
     0c 0c     
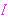  |
| 46 | 45 | adantrl 696 |
. . . . . . . . . . . . . 14
      0c 0c   0c
0c       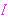  |
| 47 | 43, 46 | eqtr4d 2388 |
. . . . . . . . . . . . 13
      0c 0c   0c
0c         |
| 48 | 47 | ex 423 |
. . . . . . . . . . . 12
      0c 0c   0c
0c     
   |
| 49 | 48 | alrimivv 1632 |
. . . . . . . . . . 11
          0c 0c   0c
0c     
   |
| 50 | 4 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c       Funs Funs  |
| 51 | 5 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c     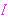     |
| 52 | 6 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c           |
| 53 | | simplr 731 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c       Nn Nn  |
| 54 | | simpr 447 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c        1c
1c    |
| 55 | 3, 50, 51, 52, 53, 54 | fnfreclem3 6320 |
. . . . . . . . . . . . . . . . . . 19
        Nn Nn      1c
1c                 |
| 56 | 55 | adantlrr 701 |
. . . . . . . . . . . . . . . . . 18
         Nn
Nn                           1c 1c                 |
| 57 | 56 | ex 423 |
. . . . . . . . . . . . . . . . 17
        Nn Nn                  
   
   
 1c
1c                 |
| 58 | 4 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c       Funs Funs  |
| 59 | 5 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c     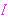     |
| 60 | 6 | ad2antrr 706 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c           |
| 61 | | simplr 731 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c       Nn Nn  |
| 62 | | simpr 447 |
. . . . . . . . . . . . . . . . . . . 20
        Nn Nn      1c
1c        1c
1c    |
| 63 | 3, 58, 59, 60, 61, 62 | fnfreclem3 6320 |
. . . . . . . . . . . . . . . . . . 19
        Nn Nn      1c
1c                 |
| 64 | 63 | adantlrr 701 |
. . . . . . . . . . . . . . . . . 18
         Nn
Nn                           1c 1c                 |
| 65 | 64 | ex 423 |
. . . . . . . . . . . . . . . . 17
        Nn Nn                  
   
   
 1c
1c                 |
| 66 | 57, 65 | anim12d 546 |
. . . . . . . . . . . . . . . 16
        Nn Nn                  
   
      1c
1c     
 1c
1c                                |
| 67 | | eeanv 1913 |
. . . . . . . . . . . . . . . 16
                                                      |
| 68 | 66, 67 | syl6ibr 218 |
. . . . . . . . . . . . . . 15
        Nn Nn                  
   
      1c
1c     
 1c
1c                                |
| 69 | | 19.29 1596 |
. . . . . . . . . . . . . . . . . . 19
                                                                                                |
| 70 | | 19.29 1596 |
. . . . . . . . . . . . . . . . . . . 20
                                                                                        |
| 71 | 70 | eximi 1576 |
. . . . . . . . . . . . . . . . . . 19
                                                                 
                          |
| 72 | 69, 71 | syl 15 |
. . . . . . . . . . . . . . . . . 18
                                                                                              |
| 73 | | pm3.35 570 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
                               
  |
| 74 | | breq1 4643 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
32
                |
| 75 | 74 | anbi1d 685 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
31
                            |
| 76 | 75 | biimpa 470 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
                            |
| 77 | | elfunsi 5832 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
32
    Funs Funs     |
| 78 | | funbrfv 5357 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
32
                   |
| 79 | 4, 77, 78 | 3syl 18 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
31
              
   |
| 80 | | funbrfv 5357 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
32
                   |
| 81 | 4, 77, 80 | 3syl 18 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
31
              
   |
| 82 | 79, 81 | anim12d 546 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
                                  |
| 83 | | eqtr2 2371 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
        
            
  |
| 84 | 76, 82, 83 | syl56 30 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
                      
   |
| 85 | 84 | exp3a 425 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
                          |
| 86 | 73, 85 | syl5 28 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
                            
                     |
| 87 | 86 | exp3a 425 |
. . . . . . . . . . . . . . . . . . . . . . . . . 26
                                                  |
| 88 | 87 | com34 77 |
. . . . . . . . . . . . . . . . . . . . . . . . 25
                                                  |
| 89 | 88 | imp3a 420 |
. . . . . . . . . . . . . . . . . . . . . . . 24
                                                  |
| 90 | 89 | com12 27 |
. . . . . . . . . . . . . . . . . . . . . . 23
                                                  |
| 91 | 90 | an4s 799 |
. . . . . . . . . . . . . . . . . . . . . 22
                                                  |
| 92 | 91 | com3l 75 |
. . . . . . . . . . . . . . . . . . . . 21
                                                  |
| 93 | 92 | imp3a 420 |
. . . . . . . . . . . . . . . . . . . 20
                  
                               |
| 94 | 93 | exlimdvv 1637 |
. . . . . . . . . . . . . . . . . . 19
                                                      |
| 95 | 94 | adantr 451 |
. . . . . . . . . . . . . . . . . 18
       Nn Nn                                                     |
| 96 | 72, 95 | syl5 28 |
. . . . . . . . . . . . . . . . 17
       Nn Nn                                                         |
| 97 | 96 | exp3a 425 |
. . . . . . . . . . . . . . . 16
       Nn Nn                    
                               
    |
| 98 | 97 | impr 602 |
. . . . . . . . . . . . . . 15
        Nn Nn                  
   
                                 |
| 99 | 68, 98 | syld 40 |
. . . . . . . . . . . . . 14
        Nn Nn                  
   
      1c
1c     
 1c
1c          |
| 100 | 99 | alrimivv 1632 |
. . . . . . . . . . . . 13
        Nn Nn                  
   
          1c
1c     
 1c
1c          |
| 101 | 100 | expr 598 |
. . . . . . . . . . . 12
       Nn Nn                    
            1c 1c  
    1c 1c           |
| 102 | 101 | ancoms 439 |
. . . . . . . . . . 11
     Nn Nn                                   1c 1c  
    1c 1c           |
| 103 | 13, 18, 23, 35, 40, 49, 102 | findsd 4411 |
. . . . . . . . . 10
     Nn Nn                         |
| 104 | 103 | 19.21bbi 1865 |
. . . . . . . . 9
     Nn Nn                     |
| 105 | 104 | ex 423 |
. . . . . . . 8
    Nn Nn                 
    |
| 106 | 105 | imp3a 420 |
. . . . . . 7
    Nn Nn                      |
| 107 | 10, 106 | mpcom 32 |
. . . . . 6
                 
  |
| 108 | 107 | ex 423 |
. . . . 5
                    |
| 109 | 108 | alrimivv 1632 |
. . . 4
                        |
| 110 | 109 | alrimiv 1631 |
. . 3
                          |
| 111 | | dffun2 5120 |
. . 3
                           |
| 112 | 110, 111 | sylibr 203 |
. 2
       |
| 113 | | df-fn 4791 |
. 2
   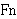 Nn Nn         Nn Nn   |
| 114 | 112, 7, 113 | sylanbrc 645 |
1
     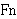 Nn Nn  |