| Step | Hyp | Ref
| Expression |
|---|
| 1 | | pm2.1 406 |
. . . 4
     NC NC    NC NC  |
| 2 | | fnspac 6284 |
. . . . . . . . . . 11
 Spac Spac 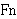 NC NC |
| 3 | | fndm 5183 |
. . . . . . . . . . 11
  Spac Spac 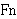 NC NC   Spac Spac
 NC NC  |
| 4 | 2, 3 | ax-mp 5 |
. . . . . . . . . 10
  Spac Spac  NC NC |
| 5 | 4 | eleq2i 2417 |
. . . . . . . . 9
     Spac Spac    NC
NC  |
| 6 | | ndmfv 5350 |
. . . . . . . . 9
      Spac Spac   Spac Spac   
   |
| 7 | 5, 6 | sylnbir 298 |
. . . . . . . 8
     NC NC   Spac Spac       |
| 8 | | 0fin 4424 |
. . . . . . . 8
   Fin Fin |
| 9 | 7, 8 | syl6eqel 2441 |
. . . . . . 7
     NC NC   Spac Spac     Fin Fin  |
| 10 | 9 | pm4.71i 613 |
. . . . . 6
     NC NC      NC NC   Spac Spac     Fin Fin   |
| 11 | 10 | orbi1i 506 |
. . . . 5
      NC NC     NC NC   Spac Spac     Fin Fin         NC NC   Spac Spac     Fin Fin      NC NC   Spac Spac   
 Fin Fin    |
| 12 | | elun 3221 |
. . . . . 6
     ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin        ∼ NC ∼ NC     NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin     |
| 13 | | vex 2863 |
. . . . . . . 8
    |
| 14 | 13 | elcompl 3226 |
. . . . . . 7
    ∼ NC ∼ NC     NC NC  |
| 15 | | elin 3220 |
. . . . . . . 8
     NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin       NC NC    ⋃1
⋃1 ∼
∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin    |
| 16 | | spacval 6283 |
. . . . . . . . . . 11
    NC NC   Spac Spac   
 Clos1 Clos1                NC NC    NC NC     2c ↑c 2c ↑c
      |
| 17 | 16 | eleq1d 2419 |
. . . . . . . . . 10
    NC NC    Spac Spac     Fin Fin  Clos1 Clos1         
      NC NC    NC NC     2c ↑c 2c ↑c
      Fin Fin   |
| 18 | 13 | eluni1 4174 |
. . . . . . . . . . 11
    ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin        ∼
∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin   |
| 19 | | df-br 4641 |
. . . . . . . . . . . . . 14
   ∼ ∼   Ins3 S
Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c             ∼ ∼
  Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  |
| 20 | | spacvallem1 6282 |
. . . . . . . . . . . . . . 15
    
      NC NC    NC NC     2c ↑c 2c ↑c
      |
| 21 | | snex 4112 |
. . . . . . . . . . . . . . 15
    
 |
| 22 | 20, 21 | nchoicelem10 6299 |
. . . . . . . . . . . . . 14
    
     ∼ ∼
  Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c    Clos1
Clos1     
          NC NC    NC NC     2c ↑c 2c ↑c
      |
| 23 | 19, 22 | bitri 240 |
. . . . . . . . . . . . 13
   ∼ ∼   Ins3 S
Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c       Clos1
Clos1                NC NC    NC NC   
 2c
↑c 2c
↑c       |
| 24 | 23 | rexbii 2640 |
. . . . . . . . . . . 12
     Fin
Fin  ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c      
 Fin Fin   Clos1 Clos1                NC NC    NC NC     2c ↑c 2c ↑c
      |
| 25 | | elima 4755 |
. . . . . . . . . . . 12
       ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin      Fin Fin  ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c     |
| 26 | | risset 2662 |
. . . . . . . . . . . 12
  Clos1 Clos1
    
          NC NC    NC NC     2c ↑c 2c ↑c
      Fin Fin     Fin Fin   Clos1 Clos1                NC NC    NC NC     2c ↑c 2c ↑c
      |
| 27 | 24, 25, 26 | 3bitr4i 268 |
. . . . . . . . . . 11
       ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin   Clos1 Clos1                NC NC    NC NC   
 2c
↑c 2c
↑c       Fin
Fin  |
| 28 | 18, 27 | bitri 240 |
. . . . . . . . . 10
    ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin   Clos1 Clos1                NC NC    NC NC   
 2c
↑c 2c
↑c       Fin
Fin  |
| 29 | 17, 28 | syl6rbbr 255 |
. . . . . . . . 9
    NC NC     ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin    Spac Spac     Fin Fin   |
| 30 | 29 | pm5.32i 618 |
. . . . . . . 8
     NC NC    ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin       NC NC   Spac Spac   
 Fin Fin   |
| 31 | 15, 30 | bitri 240 |
. . . . . . 7
     NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin       NC NC   Spac Spac   
 Fin Fin   |
| 32 | 14, 31 | orbi12i 507 |
. . . . . 6
     ∼
NC ∼
NC  
  NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin         NC NC     NC NC   Spac Spac     Fin Fin    |
| 33 | 12, 32 | bitri 240 |
. . . . 5
     ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin         NC NC     NC NC   Spac Spac     Fin Fin    |
| 34 | | andir 838 |
. . . . 5
       NC NC    NC NC    Spac
Spac     Fin Fin        NC NC   Spac Spac     Fin Fin      NC NC   Spac Spac   
 Fin Fin    |
| 35 | 11, 33, 34 | 3bitr4i 268 |
. . . 4
     ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin          NC NC    NC NC    Spac Spac     Fin Fin   |
| 36 | 1, 35 | mpbiran 884 |
. . 3
     ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin      Spac Spac     Fin Fin  |
| 37 | 36 | eqabi 2465 |
. 2
  ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin      
  Spac Spac     Fin Fin  |
| 38 | | ncsex 6112 |
. . . 4
 NC NC   |
| 39 | 38 | complex 4105 |
. . 3
 ∼ NC ∼ NC   |
| 40 | | ssetex 4745 |
. . . . . . . . . 10
 S S   |
| 41 | 40 | ins3ex 5799 |
. . . . . . . . 9
 Ins3 S Ins3 S   |
| 42 | 40 | complex 4105 |
. . . . . . . . . . . . . 14
 ∼ S ∼ S   |
| 43 | 42 | cnvex 5103 |
. . . . . . . . . . . . 13
  ∼ S ∼ S   |
| 44 | 40 | cnvex 5103 |
. . . . . . . . . . . . . 14
  S S
  |
| 45 | 20 | imageex 5802 |
. . . . . . . . . . . . . . . 16
 Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
      |
| 46 | 40, 45 | coex 4751 |
. . . . . . . . . . . . . . 15
  S S
 Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
       |
| 47 | 46 | fixex 5790 |
. . . . . . . . . . . . . 14
   S S  Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
       |
| 48 | 44, 47 | resex 5118 |
. . . . . . . . . . . . 13
   S S    S S  Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
        |
| 49 | 43, 48 | txpex 5786 |
. . . . . . . . . . . 12
   ∼ S ∼ S    S S    S S  Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
         |
| 50 | 49 | rnex 5108 |
. . . . . . . . . . 11
    ∼ S ∼ S    S S    S S  Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
         |
| 51 | 50 | complex 4105 |
. . . . . . . . . 10
 ∼ ∼    ∼ S ∼ S    S S    S S  Image Image   
      NC NC    NC NC     2c ↑c 2c ↑c
         |
| 52 | 51 | ins2ex 5798 |
. . . . . . . . 9
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         |
| 53 | 41, 52 | symdifex 4109 |
. . . . . . . 8
  Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
          |
| 54 | | 1cex 4143 |
. . . . . . . 8
 1c
1c 
 |
| 55 | 53, 54 | imaex 4748 |
. . . . . . 7
   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c   |
| 56 | 55 | complex 4105 |
. . . . . 6
 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c   |
| 57 | | finex 4398 |
. . . . . 6
 Fin Fin   |
| 58 | 56, 57 | imaex 4748 |
. . . . 5
  ∼ ∼   Ins3 S Ins3 S
 Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin    |
| 59 | 58 | uni1ex 4294 |
. . . 4
 ⋃1
⋃1 ∼
∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin    |
| 60 | 38, 59 | inex 4106 |
. . 3
  NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin     |
| 61 | 39, 60 | unex 4107 |
. 2
  ∼ NC ∼ NC   NC NC  ⋃1 ⋃1 ∼ ∼   Ins3 S Ins3 S  Ins2 ∼ Ins2 ∼    ∼
S ∼
S    S S    S S  Image Image          NC NC    NC NC     2c ↑c 2c ↑c
         1c 1c  Fin Fin      |
| 62 | 37, 61 | eqeltrri 2424 |
1
     Spac
Spac     Fin Fin    |