| Step | Hyp | Ref
| Expression |
|---|
| 1 | | eleq1 2413 |
. . . . . . . . . . . . 13
    0c 0c       0c 0c     |
| 2 | 1 | biimpcd 215 |
. . . . . . . . . . . 12
         0c
0c  0c 0c     |
| 3 | 2 | con3d 125 |
. . . . . . . . . . 11
        0c 0c       0c 0c  |
| 4 | 3 | impcom 419 |
. . . . . . . . . 10
    0c 0c            0c
0c |
| 5 | 4 | adantll 694 |
. . . . . . . . 9
      Nn Nn   0c
0c 
   
       0c 0c |
| 6 | | ssel2 3269 |
. . . . . . . . . . 11
     Nn
Nn  
      Nn Nn  |
| 7 | 6 | adantlr 695 |
. . . . . . . . . 10
      Nn Nn   0c
0c 
   
      Nn Nn  |
| 8 | | nnc0suc 4413 |
. . . . . . . . . 10
    Nn Nn     0c
0c     Nn Nn      1c
1c   |
| 9 | 7, 8 | sylib 188 |
. . . . . . . . 9
      Nn Nn   0c
0c 
   
       0c 0c   
 Nn Nn      1c 1c   |
| 10 | | orel1 371 |
. . . . . . . . 9
     0c
0c      0c 0c     Nn Nn      1c 1c 
    Nn Nn      1c 1c   |
| 11 | 5, 9, 10 | sylc 56 |
. . . . . . . 8
      Nn Nn   0c
0c 
   
       Nn Nn      1c
1c  |
| 12 | | anidm 625 |
. . . . . . . . . 10
        1c 1c       1c
1c        1c
1c  |
| 13 | | eqeq1 2359 |
. . . . . . . . . . 11
        
   1c 1c       1c 1c   |
| 14 | 13 | anbi2d 684 |
. . . . . . . . . 10
        
    1c 1c       1c
1c         1c 1c  
    1c 1c    |
| 15 | 12, 14 | syl5bbr 250 |
. . . . . . . . 9
        
   1c 1c        1c
1c
      1c
1c    |
| 16 | 15 | rexbidv 2636 |
. . . . . . . 8
        
 Nn Nn      1c 1c     Nn Nn       1c
1c
      1c
1c    |
| 17 | 11, 16 | syl5ibcom 211 |
. . . . . . 7
      Nn Nn   0c
0c 
   
            Nn Nn       1c 1c       1c
1c    |
| 18 | | eqtr3 2372 |
. . . . . . . 8
        1c 1c       1c
1c       |
| 19 | 18 | rexlimivw 2735 |
. . . . . . 7
     Nn
Nn       1c
1c
      1c
1c       |
| 20 | 17, 19 | impbid1 194 |
. . . . . 6
      Nn Nn   0c
0c 
   
            Nn Nn       1c 1c  
    1c 1c    |
| 21 | 20 | rexbidva 2632 |
. . . . 5
     Nn
Nn   0c 0c           
         Nn Nn       1c 1c  
    1c 1c    |
| 22 | | risset 2662 |
. . . . 5
              |
| 23 | | rexcom 2773 |
. . . . 5
     Nn
Nn  
   
    1c 1c       1c
1c          Nn Nn       1c 1c  
    1c 1c   |
| 24 | 21, 22, 23 | 3bitr4g 279 |
. . . 4
     Nn
Nn   0c 0c      
    
 Nn Nn      
    1c 1c       1c
1c    |
| 25 | 24 | eqabdv 2469 |
. . 3
     Nn
Nn   0c 0c      
      Nn Nn      
    1c 1c       1c
1c    |
| 26 | | df-phi 4566 |
. . . 4
 Phi Phi    Nn Nn           1c
1c     
      Nn Nn           1c
1c    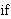    Nn Nn     1c 1c     |
| 27 | | addceq1 4384 |
. . . . . . . . 9
         1c 1c     1c 1c  |
| 28 | 27 | eqeq2d 2364 |
. . . . . . . 8
        
   1c 1c       1c 1c   |
| 29 | 28 | rexbidv 2636 |
. . . . . . 7
        
   
   1c 1c       
   1c 1c   |
| 30 | 29 | rexrab 3001 |
. . . . . 6
    
   Nn Nn   
   
   1c 1c    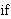  
 Nn Nn     1c 1c        Nn Nn   
   
   1c 1c    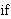  
 Nn Nn     1c 1c      |
| 31 | | iftrue 3669 |
. . . . . . . . . 10
    Nn Nn  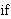    Nn Nn     1c 1c        1c 1c  |
| 32 | 31 | eqeq2d 2364 |
. . . . . . . . 9
    Nn Nn     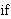  
 Nn Nn     1c 1c          1c
1c   |
| 33 | 32 | anbi2d 684 |
. . . . . . . 8
    Nn Nn             1c
1c
   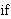    Nn Nn     1c 1c                1c
1c
      1c
1c    |
| 34 | | r19.41v 2765 |
. . . . . . . 8
    
   
   1c 1c       1c
1c      
      1c
1c
      1c
1c   |
| 35 | 33, 34 | syl6bbr 254 |
. . . . . . 7
    Nn Nn             1c
1c
   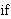    Nn Nn     1c 1c                1c
1c
      1c
1c    |
| 36 | 35 | rexbiia 2648 |
. . . . . 6
     Nn
Nn           1c
1c
   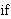    Nn Nn     1c 1c         Nn Nn           1c
1c
      1c
1c   |
| 37 | 30, 36 | bitri 240 |
. . . . 5
    
   Nn Nn   
   
   1c 1c    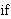  
 Nn Nn     1c 1c        Nn Nn   
   
   1c 1c       1c
1c   |
| 38 | 37 | abbii 2466 |
. . . 4
          Nn
Nn           1c
1c    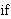    Nn Nn     1c 1c            Nn Nn      
    1c 1c       1c
1c   |
| 39 | 26, 38 | eqtri 2373 |
. . 3
 Phi Phi    Nn Nn           1c
1c     
   Nn Nn   
   
   1c 1c       1c
1c   |
| 40 | 25, 39 | syl6eqr 2403 |
. 2
     Nn
Nn   0c 0c       Phi
Phi  
 Nn Nn           1c
1c   |
| 41 | | dfrab2 3531 |
. . . 4
    Nn Nn   
   
   1c 1c      
         1c
1c   Nn Nn  |
| 42 | | vex 2863 |
. . . . . . . . 9
    |
| 43 | 42 | elimak 4260 |
. . . . . . . 8
      kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k              kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  |
| 44 | | vex 2863 |
. . . . . . . . . . 11
    |
| 45 | 42, 44 | opkelimagek 4273 |
. . . . . . . . . 10
        Imagek
Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c       Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k   |
| 46 | 44, 42 | opkelcnvk 4251 |
. . . . . . . . . 10
         kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c        Imagek Imagek  Ins3k ∼ Ins3k ∼
  Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  |
| 47 | | dfaddc2 4382 |
. . . . . . . . . . 11
    1c 1c 
   Ins3k ∼ Ins3k ∼
  Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k  |
| 48 | 47 | eqeq2i 2363 |
. . . . . . . . . 10
       1c 1c   
   Ins3k ∼ Ins3k ∼
  Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k   |
| 49 | 45, 46, 48 | 3bitr4i 268 |
. . . . . . . . 9
         kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c       1c 1c  |
| 50 | 49 | rexbii 2640 |
. . . . . . . 8
    
     
  kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c       
   1c 1c  |
| 51 | 43, 50 | bitri 240 |
. . . . . . 7
      kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k            1c
1c  |
| 52 | 51 | eqabi 2465 |
. . . . . 6
   kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k     
         1c
1c  |
| 53 | | addcexlem 4383 |
. . . . . . . . . 10
  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c 
  |
| 54 | | 1cex 4143 |
. . . . . . . . . . . 12
 1c
1c 
 |
| 55 | 54 | pw1ex 4304 |
. . . . . . . . . . 11
  1
1c 1
1c 
 |
| 56 | 55 | pw1ex 4304 |
. . . . . . . . . 10
  1 1  1
1c 1
1c 
 |
| 57 | 53, 56 | imakex 4301 |
. . . . . . . . 9
   Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c 
 |
| 58 | 57 | imagekex 4313 |
. . . . . . . 8
 Imagek
Imagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c 
 |
| 59 | 58 | cnvkex 4288 |
. . . . . . 7
  kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c 
 |
| 60 | | phiall.1 |
. . . . . . 7
    |
| 61 | 59, 60 | imakex 4301 |
. . . . . 6
   kImagek kImagek  Ins3k ∼ Ins3k ∼   Ins3k Sk Ins3k Sk  Ins2k Sk Ins2k Sk   k k 1 1  1 1c 1 1c    Ins2k Ins2k Sk Ins2k Ins2k Sk   Ins2k Ins3k Sk Ins2k Ins3k Sk  Ins3k SIk SIk Sk Ins3k SIk SIk Sk    k k 1 1  1 1  1 1  1 1c 1 1c   k k 1 1  1 1c 1 1c  k k    |
| 62 | 52, 61 | eqeltrri 2424 |
. . . . 5
         
   1c 1c    |
| 63 | | nncex 4397 |
. . . . 5
 Nn Nn   |
| 64 | 62, 63 | inex 4106 |
. . . 4
    
         1c
1c   Nn Nn    |
| 65 | 41, 64 | eqeltri 2423 |
. . 3
    Nn Nn   
   
   1c 1c    |
| 66 | | phieq 4571 |
. . . 4
       Nn Nn    
      1c
1c   Phi
Phi   Phi Phi
   Nn Nn    
      1c
1c   |
| 67 | 66 | eqeq2d 2364 |
. . 3
       Nn Nn    
      1c
1c      Phi
Phi     Phi Phi    Nn Nn           1c
1c    |
| 68 | 65, 67 | spcev 2947 |
. 2
    Phi Phi    Nn Nn           1c
1c     
 Phi Phi   |
| 69 | 40, 68 | syl 15 |
1
     Nn
Nn   0c 0c      
  Phi Phi   |