| Colors of
variables: wff set class |
Syntax hints:  wtru 1354 wtru 1354
 wnf 1460 wnf 1460
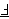 wnfc 2306 wnfc 2306
 wrex 2456 wrex 2456 |
| This theorem was proved from axioms:
ax-mp 5 ax-1 6 ax-2 7
ax-ia1 106 ax-ia2 107 ax-ia3 108 ax-5 1447 ax-7 1448
ax-gen 1449 ax-ie1 1493 ax-ie2 1494 ax-4 1510 ax-17 1526 ax-ial 1534 ax-i5r 1535 ax-ext 2159 |
| This theorem depends on definitions:
df-bi 117 df-tru 1356 df-nf 1461 df-cleq 2170 df-clel 2173 df-nfc 2308 df-rex 2461 |
| This theorem is referenced by: r19.12
2583 sbcrext
3041 nfuni
3816 nfiunxy
3913 rexxpf
4775 abrexex2g
6121 abrexex2
6125 nfrecs
6308 fimaxre2
11235 nfsum
11365 nfcprod1
11562 nfcprod
11563 bezoutlemmain
11999 ctiunctlemfo
12440 bj-findis
14734 strcollnfALT
14741 |