Theorem addcfnex 5825

Description: The cardinal addition function exists. (Contributed by SF, 12-Feb-2015.)

Assertion

Ref	Expression
addcfnex	|- AddC e. _V

Proof of Theorem addcfnex

Dummy variables x y z a b p are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		df-addcfn 5747	. . 3 |- AddC = (x e. _V, y e. _V |-> (x +o y))
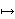
2		elin 3220	. . . . . . . 8 |- (<.{b}, <.{z}, <.x, y>.>.>. e. ( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)) <-> (<.{b}, <.{z}, <.x, y>.>.>. e. Ins2 Ins2 S /\ <.{b}, <.{z}, <.x, y>.>.>. e. Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)))
3		snex 4112	. . . . . . . . . . 11 |- {z} e. _V
4	3	otelins2 5792	. . . . . . . . . 10 |- (<.{b}, <.{z}, <.x, y>.>.>. e. Ins2 Ins2 S <-> <.{b}, <.x, y>.>. e. Ins2 S )
5		vex 2863	. . . . . . . . . . 11 |- x e. _V
6	5	otelins2 5792	. . . . . . . . . 10 |- (<.{b}, <.x, y>.>. e. Ins2 S <-> <.{b}, y>. e. S )
7		vex 2863	. . . . . . . . . . 11 |- b e. _V
8		vex 2863	. . . . . . . . . . 11 |- y e. _V
9	7, 8	opelssetsn 4761	. . . . . . . . . 10 |- (<.{b}, y>. e. S <-> b e. y)
10	4, 6, 9	3bitri 262	. . . . . . . . 9 |- (<.{b}, <.{z}, <.x, y>.>.>. e. Ins2 Ins2 S <-> b e. y)
11	8	oqelins4 5795	. . . . . . . . . 10 |- (<.{b}, <.{z}, <.x, y>.>.>. e. Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) <-> <.{b}, <.{z}, x>.>. e. (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))
12		elin 3220	. . . . . . . . . . . . 13 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. ( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))) <-> (<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins2 Ins2 S /\ <.{a}, <.{b}, <.{z}, x>.>.>. e. Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))))
13		snex 4112	. . . . . . . . . . . . . . . 16 |- {b} e. _V
14	13	otelins2 5792	. . . . . . . . . . . . . . 15 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins2 Ins2 S <-> <.{a}, <.{z}, x>.>. e. Ins2 S )
15	3	otelins2 5792	. . . . . . . . . . . . . . 15 |- (<.{a}, <.{z}, x>.>. e. Ins2 S <-> <.{a}, x>. e. S )
16		vex 2863	. . . . . . . . . . . . . . . 16 |- a e. _V
17	16, 5	opelssetsn 4761	. . . . . . . . . . . . . . 15 |- (<.{a}, x>. e. S <-> a e. x)
18	14, 15, 17	3bitri 262	. . . . . . . . . . . . . 14 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins2 Ins2 S <-> a e. x)
19	5	oqelins4 5795	. . . . . . . . . . . . . . 15 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> <.{a}, <.{b}, {z}>.>. e. SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))
20		vex 2863	. . . . . . . . . . . . . . . 16 |- z e. _V
21	16, 7, 20	otsnelsi3 5806	. . . . . . . . . . . . . . 15 |- (<.{a}, <.{b}, {z}>.>. e. SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> <.a, <.b, z>.>. e. ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))
22		elin 3220	. . . . . . . . . . . . . . . 16 |- (<.a, <.b, z>.>. e. ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> (<.a, <.b, z>.>. e. Ins3 Disj /\ <.a, <.b, z>.>. e. (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))
23	20	otelins3 5793	. . . . . . . . . . . . . . . . . 18 |- (<.a, <.b, z>.>. e. Ins3 Disj <-> <.a, b>. e. Disj )
24		df-br 4641	. . . . . . . . . . . . . . . . . 18 |- (a Disj b <-> <.a, b>. e. Disj )
25	16, 7	brdisj 5823	. . . . . . . . . . . . . . . . . 18 |- (a Disj b <-> (a i^i b) = (/))
26	23, 24, 25	3bitr2i 264	. . . . . . . . . . . . . . . . 17 |- (<.a, <.b, z>.>. e. Ins3 Disj <-> (a i^i b) = (/))
27		trtxp 5782	. . . . . . . . . . . . . . . . . . . . . . 23 |- (p((2nd o. 1st) (x) 2nd)<.b, z>. <-> (p(2nd o. 1st)b /\ p2ndz))
28	27	anbi2i 675	. . . . . . . . . . . . . . . . . . . . . 22 |- ((p(1st o. 1st)a /\ p((2nd o. 1st) (x) 2nd)<.b, z>.) <-> (p(1st o. 1st)a /\ (p(2nd o. 1st)b /\ p2ndz)))
29		trtxp 5782	. . . . . . . . . . . . . . . . . . . . . 22 |- (p((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))<.a, <.b, z>.>. <-> (p(1st o. 1st)a /\ p((2nd o. 1st) (x) 2nd)<.b, z>.))
30		anass 630	. . . . . . . . . . . . . . . . . . . . . 22 |- (((p(1st o. 1st)a /\ p(2nd o. 1st)b) /\ p2ndz) <-> (p(1st o. 1st)a /\ (p(2nd o. 1st)b /\ p2ndz)))
31	28, 29, 30	3bitr4i 268	. . . . . . . . . . . . . . . . . . . . 21 |- (p((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))<.a, <.b, z>.>. <-> ((p(1st o. 1st)a /\ p(2nd o. 1st)b) /\ p2ndz))
32		brco 4884	. . . . . . . . . . . . . . . . . . . . . . . . . 26 |- (p(1st o. 1st)a <-> E.x(p1stx /\ x1sta))
33	16	br1st 4859	. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29 |- (x1sta <-> E.y x = <.a, y>.)
34	33	anbi2i 675	. . . . . . . . . . . . . . . . . . . . . . . . . . . 28 |- ((p1stx /\ x1sta) <-> (p1stx /\ E.y x = <.a, y>.))
35		19.42v 1905	. . . . . . . . . . . . . . . . . . . . . . . . . . . 28 |- (E.y(p1stx /\ x = <.a, y>.) <-> (p1stx /\ E.y x = <.a, y>.))
36	34, 35	bitr4i 243	. . . . . . . . . . . . . . . . . . . . . . . . . . 27 |- ((p1stx /\ x1sta) <-> E.y(p1stx /\ x = <.a, y>.))
37	36	exbii 1582	. . . . . . . . . . . . . . . . . . . . . . . . . 26 |- (E.x(p1stx /\ x1sta) <-> E.xE.y(p1stx /\ x = <.a, y>.))
38		excom 1741	. . . . . . . . . . . . . . . . . . . . . . . . . . 27 |- (E.xE.y(p1stx /\ x = <.a, y>.) <-> E.yE.x(p1stx /\ x = <.a, y>.))
39		exancom 1586	. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29 |- (E.x(p1stx /\ x = <.a, y>.) <-> E.x(x = <.a, y>. /\ p1stx))
40	16, 8	opex 4589	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . 30 |- <.a, y>. e. _V
41		breq2 4644	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . 30 |- (x = <.a, y>. -> (p1stx <-> p1st<.a, y>.))
42	40, 41	ceqsexv 2895	. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29 |- (E.x(x = <.a, y>. /\ p1stx) <-> p1st<.a, y>.)
43	39, 42	bitri 240	. . . . . . . . . . . . . . . . . . . . . . . . . . . 28 |- (E.x(p1stx /\ x = <.a, y>.) <-> p1st<.a, y>.)
44	43	exbii 1582	. . . . . . . . . . . . . . . . . . . . . . . . . . 27 |- (E.yE.x(p1stx /\ x = <.a, y>.) <-> E.y p1st<.a, y>.)
45	38, 44	bitri 240	. . . . . . . . . . . . . . . . . . . . . . . . . 26 |- (E.xE.y(p1stx /\ x = <.a, y>.) <-> E.y p1st<.a, y>.)
46	32, 37, 45	3bitri 262	. . . . . . . . . . . . . . . . . . . . . . . . 25 |- (p(1st o. 1st)a <-> E.y p1st<.a, y>.)
47	46	anbi1i 676	. . . . . . . . . . . . . . . . . . . . . . . 24 |- ((p(1st o. 1st)a /\ p(2nd o. 1st)b) <-> (E.y p1st<.a, y>. /\ p(2nd o. 1st)b))
48		19.41v 1901	. . . . . . . . . . . . . . . . . . . . . . . 24 |- (E.y(p1st<.a, y>. /\ p(2nd o. 1st)b) <-> (E.y p1st<.a, y>. /\ p(2nd o. 1st)b))
49	40	br1st 4859	. . . . . . . . . . . . . . . . . . . . . . . . . . . 28 |- (p1st<.a, y>. <-> E.z p = <.<.a, y>., z>.)
50		breq1 4643	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . 30 |- (p = <.<.a, y>., z>. -> (p(2nd o. 1st)b <-> <.<.a, y>., z>.(2nd o. 1st)b))
51	40, 20	brco1st 5778	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 31 |- (<.<.a, y>., z>.(2nd o. 1st)b <-> <.a, y>.2ndb)
52	16, 8	opbr2nd 5503	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 31 |- (<.a, y>.2ndb <-> y = b)
53	51, 52	bitri 240	. . . . . . . . . . . . . . . . . . . . . . . . . . . . . 30 |- (<.<.a, y>., z>.(2nd o. 1st)b <-> y = b)
54	50, 53	syl6bb 252	. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29 |- (p = <.<.a, y>., z>. -> (p(2nd o. 1st)b <-> y = b))
55	54	exlimiv 1634	. . . . . . . . . . . . . . . . . . . . . . . . . . . 28 |- (E.z p = <.<.a, y>., z>. -> (p(2nd o. 1st)b <-> y = b))
56	49, 55	sylbi 187	. . . . . . . . . . . . . . . . . . . . . . . . . . 27 |- (p1st<.a, y>. -> (p(2nd o. 1st)b <-> y = b))
57	56	pm5.32i 618	. . . . . . . . . . . . . . . . . . . . . . . . . 26 |- ((p1st<.a, y>. /\ p(2nd o. 1st)b) <-> (p1st<.a, y>. /\ y = b))
58	57	exbii 1582	. . . . . . . . . . . . . . . . . . . . . . . . 25 |- (E.y(p1st<.a, y>. /\ p(2nd o. 1st)b) <-> E.y(p1st<.a, y>. /\ y = b))
59		exancom 1586	. . . . . . . . . . . . . . . . . . . . . . . . 25 |- (E.y(p1st<.a, y>. /\ y = b) <-> E.y(y = b /\ p1st<.a, y>.))
60	58, 59	bitri 240	. . . . . . . . . . . . . . . . . . . . . . . 24 |- (E.y(p1st<.a, y>. /\ p(2nd o. 1st)b) <-> E.y(y = b /\ p1st<.a, y>.))
61	47, 48, 60	3bitr2i 264	. . . . . . . . . . . . . . . . . . . . . . 23 |- ((p(1st o. 1st)a /\ p(2nd o. 1st)b) <-> E.y(y = b /\ p1st<.a, y>.))
62		opeq2 4580	. . . . . . . . . . . . . . . . . . . . . . . . 25 |- (y = b -> <.a, y>. = <.a, b>.)
63	62	breq2d 4652	. . . . . . . . . . . . . . . . . . . . . . . 24 |- (y = b -> (p1st<.a, y>. <-> p1st<.a, b>.))
64	7, 63	ceqsexv 2895	. . . . . . . . . . . . . . . . . . . . . . 23 |- (E.y(y = b /\ p1st<.a, y>.) <-> p1st<.a, b>.)
65	61, 64	bitri 240	. . . . . . . . . . . . . . . . . . . . . 22 |- ((p(1st o. 1st)a /\ p(2nd o. 1st)b) <-> p1st<.a, b>.)
66	65	anbi1i 676	. . . . . . . . . . . . . . . . . . . . 21 |- (((p(1st o. 1st)a /\ p(2nd o. 1st)b) /\ p2ndz) <-> (p1st<.a, b>. /\ p2ndz))
67	16, 7	opex 4589	. . . . . . . . . . . . . . . . . . . . . 22 |- <.a, b>. e. _V
68	67, 20	op1st2nd 5791	. . . . . . . . . . . . . . . . . . . . 21 |- ((p1st<.a, b>. /\ p2ndz) <-> p = <.<.a, b>., z>.)
69	31, 66, 68	3bitri 262	. . . . . . . . . . . . . . . . . . . 20 |- (p((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))<.a, <.b, z>.>. <-> p = <.<.a, b>., z>.)
70	69	rexbii 2640	. . . . . . . . . . . . . . . . . . 19 |- (E.p e. Cup p((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))<.a, <.b, z>.>. <-> E.p e. Cup p = <.<.a, b>., z>.)
71		elima 4755	. . . . . . . . . . . . . . . . . . 19 |- (<.a, <.b, z>.>. e. (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ) <-> E.p e. Cup p((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))<.a, <.b, z>.>.)
72		risset 2662	. . . . . . . . . . . . . . . . . . 19 |- (<.<.a, b>., z>. e. Cup <-> E.p e. Cup p = <.<.a, b>., z>.)
73	70, 71, 72	3bitr4i 268	. . . . . . . . . . . . . . . . . 18 |- (<.a, <.b, z>.>. e. (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ) <-> <.<.a, b>., z>. e. Cup )
74		df-br 4641	. . . . . . . . . . . . . . . . . 18 |- (<.a, b>. Cup z <-> <.<.a, b>., z>. e. Cup )
75	16, 7	brcup 5816	. . . . . . . . . . . . . . . . . 18 |- (<.a, b>. Cup z <-> z = (a u. b))
76	73, 74, 75	3bitr2i 264	. . . . . . . . . . . . . . . . 17 |- (<.a, <.b, z>.>. e. (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ) <-> z = (a u. b))
77	26, 76	anbi12i 678	. . . . . . . . . . . . . . . 16 |- ((<.a, <.b, z>.>. e. Ins3 Disj /\ <.a, <.b, z>.>. e. (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> ((a i^i b) = (/) /\ z = (a u. b)))
78	22, 77	bitri 240	. . . . . . . . . . . . . . 15 |- (<.a, <.b, z>.>. e. ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> ((a i^i b) = (/) /\ z = (a u. b)))
79	19, 21, 78	3bitri 262	. . . . . . . . . . . . . 14 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) <-> ((a i^i b) = (/) /\ z = (a u. b)))
80	18, 79	anbi12i 678	. . . . . . . . . . . . 13 |- ((<.{a}, <.{b}, <.{z}, x>.>.>. e. Ins2 Ins2 S /\ <.{a}, <.{b}, <.{z}, x>.>.>. e. Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))) <-> (a e. x /\ ((a i^i b) = (/) /\ z = (a u. b))))
81	12, 80	bitri 240	. . . . . . . . . . . 12 |- (<.{a}, <.{b}, <.{z}, x>.>.>. e. ( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))) <-> (a e. x /\ ((a i^i b) = (/) /\ z = (a u. b))))
82	81	exbii 1582	. . . . . . . . . . 11 |- (E.a<.{a}, <.{b}, <.{z}, x>.>.>. e. ( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))) <-> E.a(a e. x /\ ((a i^i b) = (/) /\ z = (a u. b))))
83		elima1c 4948	. . . . . . . . . . 11 |- (<.{b}, <.{z}, x>.>. e. (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) <-> E.a<.{a}, <.{b}, <.{z}, x>.>.>. e. ( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))))
84		df-rex 2621	. . . . . . . . . . 11 |- (E.a e. x ((a i^i b) = (/) /\ z = (a u. b)) <-> E.a(a e. x /\ ((a i^i b) = (/) /\ z = (a u. b))))
85	82, 83, 84	3bitr4i 268	. . . . . . . . . 10 |- (<.{b}, <.{z}, x>.>. e. (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) <-> E.a e. x ((a i^i b) = (/) /\ z = (a u. b)))
86	11, 85	bitri 240	. . . . . . . . 9 |- (<.{b}, <.{z}, <.x, y>.>.>. e. Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) <-> E.a e. x ((a i^i b) = (/) /\ z = (a u. b)))
87	10, 86	anbi12i 678	. . . . . . . 8 |- ((<.{b}, <.{z}, <.x, y>.>.>. e. Ins2 Ins2 S /\ <.{b}, <.{z}, <.x, y>.>.>. e. Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)) <-> (b e. y /\ E.a e. x ((a i^i b) = (/) /\ z = (a u. b))))
88	2, 87	bitri 240	. . . . . . 7 |- (<.{b}, <.{z}, <.x, y>.>.>. e. ( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)) <-> (b e. y /\ E.a e. x ((a i^i b) = (/) /\ z = (a u. b))))
89	88	exbii 1582	. . . . . 6 |- (E.b<.{b}, <.{z}, <.x, y>.>.>. e. ( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)) <-> E.b(b e. y /\ E.a e. x ((a i^i b) = (/) /\ z = (a u. b))))
90		elima1c 4948	. . . . . 6 |- (<.{z}, <.x, y>.>. e. (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c) <-> E.b<.{b}, <.{z}, <.x, y>.>.>. e. ( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)))
91		df-rex 2621	. . . . . 6 |- (E.b e. y E.a e. x ((a i^i b) = (/) /\ z = (a u. b)) <-> E.b(b e. y /\ E.a e. x ((a i^i b) = (/) /\ z = (a u. b))))
92	89, 90, 91	3bitr4i 268	. . . . 5 |- (<.{z}, <.x, y>.>. e. (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c) <-> E.b e. y E.a e. x ((a i^i b) = (/) /\ z = (a u. b)))
93		eladdc 4399	. . . . . 6 |- (z e. (x +o y) <-> E.a e. x E.b e. y ((a i^i b) = (/) /\ z = (a u. b)))
94		rexcom 2773	. . . . . 6 |- (E.a e. x E.b e. y ((a i^i b) = (/) /\ z = (a u. b)) <-> E.b e. y E.a e. x ((a i^i b) = (/) /\ z = (a u. b)))
95	93, 94	bitri 240	. . . . 5 |- (z e. (x +o y) <-> E.b e. y E.a e. x ((a i^i b) = (/) /\ z = (a u. b)))
96	92, 95	bitr4i 243	. . . 4 |- (<.{z}, <.x, y>.>. e. (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c) <-> z e. (x +o y))
97	96	releqmpt2 5810	. . 3 |- (((_V X. _V) X. _V) \ (( Ins2 S (+) Ins3 (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c))"1c)) = (x e. _V, y e. _V |-> (x +o y))
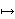98	1, 97	eqtr4i 2376	. 2 |- AddC = (((_V X. _V) X. _V) \ (( Ins2 S (+) Ins3 (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c))"1c))
99		vvex 4110	. . 3 |- _V e. _V
100		ssetex 4745	. . . . . . 7 |- S e. _V
101	100	ins2ex 5798	. . . . . 6 |- Ins2 S e. _V
102	101	ins2ex 5798	. . . . 5 |- Ins2 Ins2 S e. _V
103		disjex 5824	. . . . . . . . . . . 12 |- Disj e. _V
104	103	ins3ex 5799	. . . . . . . . . . 11 |- Ins3 Disj e. _V
105		1stex 4740	. . . . . . . . . . . . . 14 |- 1st e. _V
106	105, 105	coex 4751	. . . . . . . . . . . . 13 |- (1st o. 1st) e. _V
107		2ndex 5113	. . . . . . . . . . . . . . 15 |- 2nd e. _V
108	107, 105	coex 4751	. . . . . . . . . . . . . 14 |- (2nd o. 1st) e. _V
109	108, 107	txpex 5786	. . . . . . . . . . . . 13 |- ((2nd o. 1st) (x) 2nd) e. _V
110	106, 109	txpex 5786	. . . . . . . . . . . 12 |- ((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd)) e. _V
111		cupex 5817	. . . . . . . . . . . 12 |- Cup e. _V
112	110, 111	imaex 4748	. . . . . . . . . . 11 |- (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ) e. _V
113	104, 112	inex 4106	. . . . . . . . . 10 |- ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) e. _V
114	113	si3ex 5807	. . . . . . . . 9 |- SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) e. _V
115	114	ins4ex 5800	. . . . . . . 8 |- Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )) e. _V
116	102, 115	inex 4106	. . . . . . 7 |- ( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup ))) e. _V
117		1cex 4143	. . . . . . 7 |- 1c e. _V
118	116, 117	imaex 4748	. . . . . 6 |- (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) e. _V
119	118	ins4ex 5800	. . . . 5 |- Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c) e. _V
120	102, 119	inex 4106	. . . 4 |- ( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c)) e. _V
121	120, 117	imaex 4748	. . 3 |- (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c) e. _V
122	99, 99, 121	mpt2exlem 5812	. 2 |- (((_V X. _V) X. _V) \ (( Ins2 S (+) Ins3 (( Ins2 Ins2 S i^i Ins4 (( Ins2 Ins2 S i^i Ins4 SI3 ( Ins3 Disj i^i (((1st o. 1st) (x) ((2nd o. 1st) (x) 2nd))" Cup )))"1c))"1c))"1c)) e. _V
123	98, 122	eqeltri 2423	1 |- AddC e. _V

Syntax hints:   <-> wb 176   /\ wa 358  E.wex 1541   = wceq 1642   e. wcel 1710  E.wrex 2616  _Vcvv 2860   \ cdif 3207   u. cun 3208   i^i cin 3209   (+) csymdif 3210  (/)c0 3551  {csn 3738  1_cc1c 4135   +o cplc 4376  <.cop 4562   class class class wbr 4640  1stc1st 4718   S csset 4720   o. ccom 4722  "cima 4723   X. cxp 4771  2ndc2nd 4784   |-> cmpt2 5654   (x) ctxp 5736   Cup ccup 5742   Disj cdisj 5744   AddC caddcfn 5746   Ins2 cins2 5750   Ins3 cins3 5752   Ins4 cins4 5756   SI₃ csi3 5758

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1546  ax-5 1557  ax-17 1616  ax-9 1654  ax-8 1675  ax-13 1712  ax-14 1714  ax-6 1729  ax-7 1734  ax-11 1746  ax-12 1925  ax-ext 2334  ax-nin 4079  ax-xp 4080  ax-cnv 4081  ax-1c 4082  ax-sset 4083  ax-si 4084  ax-ins2 4085  ax-ins3 4086  ax-typlower 4087  ax-sn 4088

This theorem depends on definitions:  df-bi 177  df-or 359  df-an 360  df-3or 935  df-3an 936  df-nan 1288  df-tru 1319  df-ex 1542  df-nf 1545  df-sb 1649  df-eu 2208  df-mo 2209  df-clab 2340  df-cleq 2346  df-clel 2349  df-nfc 2479  df-ne 2519  df-ral 2620  df-rex 2621  df-reu 2622  df-rmo 2623  df-rab 2624  df-v 2862  df-sbc 3048  df-csb 3138  df-nin 3212  df-compl 3213  df-in 3214  df-un 3215  df-dif 3216  df-symdif 3217  df-ss 3260  df-pss 3262  df-nul 3552  df-if 3664  df-pw 3725  df-sn 3742  df-pr 3743  df-uni 3893  df-int 3928  df-iun 3972  df-opk 4059  df-1c 4137  df-pw1 4138  df-uni1 4139  df-xpk 4186  df-cnvk 4187  df-ins2k 4188  df-ins3k 4189  df-imak 4190  df-cok 4191  df-p6 4192  df-sik 4193  df-ssetk 4194  df-imagek 4195  df-idk 4196  df-iota 4340  df-0c 4378  df-addc 4379  df-nnc 4380  df-fin 4381  df-lefin 4441  df-ltfin 4442  df-ncfin 4443  df-tfin 4444  df-evenfin 4445  df-oddfin 4446  df-sfin 4447  df-spfin 4448  df-phi 4566  df-op 4567  df-proj1 4568  df-proj2 4569  df-opab 4624  df-br 4641  df-1st 4724  df-swap 4725  df-sset 4726  df-co 4727  df-ima 4728  df-si 4729  df-id 4768  df-xp 4785  df-cnv 4786  df-rn 4787  df-dm 4788  df-res 4789  df-fun 4790  df-fn 4791  df-f 4792  df-fo 4794  df-fv 4796  df-2nd 4798  df-ov 5527  df-oprab 5529  df-mpt 5653  df-mpt2 5655  df-txp 5737  df-cup 5743  df-disj 5745  df-addcfn 5747  df-ins2 5751  df-ins3 5753  df-ins4 5757  df-si3 5759

This theorem is referenced by:  csucex  6260  addccan2nclem2  6265  nncdiv3lem2  6277  nnc3n3p1  6279  nchoicelem16  6305