Proof of Theorem endjusym
| Step | Hyp | Ref
| Expression |
|---|
| 1 | | djulf1o 7119 |
. . . . . . . . 9
 inl inl          |
| 2 | | f1of1 5500 |
. . . . . . . . 9
  inl inl           inl inl      
    |
| 3 | 1, 2 | ax-mp 5 |
. . . . . . . 8
 inl inl          |
| 4 | | ssv 3202 |
. . . . . . . 8
    |
| 5 | | f1ores 5516 |
. . . . . . . 8
   inl inl      
    
     inl inl        inl inl    |
| 6 | 3, 4, 5 | mp2an 426 |
. . . . . . 7
  inl inl        inl inl   |
| 7 | | f1oeng 6813 |
. . . . . . 7
        inl inl        inl inl    
   inl inl    |
| 8 | 6, 7 | mpan2 425 |
. . . . . 6
         inl inl    |
| 9 | 8 | ensymd 6839 |
. . . . 5
       inl inl      |
| 10 | | djurf1o 7120 |
. . . . . . . 8
 inr inr          |
| 11 | | f1of1 5500 |
. . . . . . . 8
  inr inr         
 inr inr           |
| 12 | 10, 11 | ax-mp 5 |
. . . . . . 7
 inr inr          |
| 13 | | f1ores 5516 |
. . . . . . 7
   inr inr                 inr inr        inr inr    |
| 14 | 12, 4, 13 | mp2an 426 |
. . . . . 6
  inr inr        inr inr   |
| 15 | | f1oeng 6813 |
. . . . . 6
        inr inr        inr inr    
   inr inr    |
| 16 | 14, 15 | mpan2 425 |
. . . . 5
         inr inr    |
| 17 | | entr 6840 |
. . . . 5
    inl inl         inr inr    
 inl inl   
 inr inr    |
| 18 | 9, 16, 17 | syl2anc 411 |
. . . 4
       inl inl     inr inr    |
| 19 | 18 | adantr 276 |
. . 3
        
    inl inl     inr inr    |
| 20 | | ssv 3202 |
. . . . . . . 8
    |
| 21 | | f1ores 5516 |
. . . . . . . 8
   inr inr                 inr inr        inr inr    |
| 22 | 12, 20, 21 | mp2an 426 |
. . . . . . 7
  inr inr        inr inr   |
| 23 | | f1oeng 6813 |
. . . . . . 7
        inr inr        inr inr    
   inr inr    |
| 24 | 22, 23 | mpan2 425 |
. . . . . 6
         inr inr    |
| 25 | 24 | adantl 277 |
. . . . 5
        
      inr inr    |
| 26 | 25 | ensymd 6839 |
. . . 4
        
    inr inr      |
| 27 | | f1ores 5516 |
. . . . . . 7
   inl inl      
    
     inl inl        inl inl    |
| 28 | 3, 20, 27 | mp2an 426 |
. . . . . 6
  inl inl        inl inl   |
| 29 | | f1oeng 6813 |
. . . . . 6
        inl inl        inl inl    
   inl inl    |
| 30 | 28, 29 | mpan2 425 |
. . . . 5
         inl inl    |
| 31 | 30 | adantl 277 |
. . . 4
        
      inl inl    |
| 32 | | entr 6840 |
. . . 4
    inr inr         inl inl    
 inr inr   
 inl inl    |
| 33 | 26, 31, 32 | syl2anc 411 |
. . 3
        
    inr inr     inl inl    |
| 34 | | djuin 7125 |
. . . 4
   inl inl   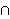  inr inr      |
| 35 | 34 | a1i 9 |
. . 3
        
     inl inl   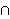
 inr inr       |
| 36 | | incom 3352 |
. . . . 5
   inl inl   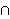  inr inr       inr inr   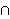
 inl inl    |
| 37 | | djuin 7125 |
. . . . 5
   inl inl   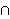  inr inr      |
| 38 | 36, 37 | eqtr3i 2216 |
. . . 4
   inr inr   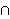  inl inl      |
| 39 | 38 | a1i 9 |
. . 3
        
     inr inr   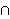
 inl inl       |
| 40 | | unen 6872 |
. . 3
     inl inl   
 inr inr   
 inr inr   
 inl inl        inl inl   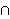
 inr inr         inr inr   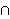
 inl inl           inl inl     inr inr       inr inr     inl inl     |
| 41 | 19, 33, 35, 39, 40 | syl22anc 1250 |
. 2
        
     inl inl     inr inr       inr inr     inl inl     |
| 42 | | djuun 7128 |
. 2
   inl inl     inr inr       ⊔ ⊔
  |
| 43 | | uncom 3304 |
. . 3
   inr inr     inl inl       inl inl     inr inr    |
| 44 | | djuun 7128 |
. . 3
   inl inl     inr inr       ⊔ ⊔
  |
| 45 | 43, 44 | eqtri 2214 |
. 2
   inr inr     inl inl       ⊔ ⊔
  |
| 46 | 41, 42, 45 | 3brtr3g 4063 |
1
        
     ⊔ ⊔
     ⊔ ⊔
   |