Theorem 2ndconst 6277

Description: The mapping of a restriction of the 2nd function to a converse constant function. (Contributed by NM, 27-Mar-2008.)

Assertion

Ref	Expression
2ndconst	|- ( A e. V -> ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -1-1-onto-> B )

Proof of Theorem 2ndconst

Dummy variables x y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		snmg 3737	. . 3 |- ( A e. V -> E. x x e. { A } )
2		fo2ndresm 6217	. . 3 |- ( E. x x e. { A } -> ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -onto-> B )
3	1, 2	syl 14	. 2 |- ( A e. V -> ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -onto-> B )
4		moeq 2936	. . . . . 6 |- E* x x = <. A , y >.
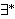
5	4	moani 2112	. . . . 5 |- E* x ( y e. B /\ x = <. A , y >. )
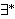
6		vex 2763	. . . . . . . 8 |- y e. _V
7	6	brres 4949	. . . . . . 7 |- ( x ( 2nd |` ( { A } X. B ) ) y <-> ( x 2nd y /\ x e. ( { A } X. B ) ) )
8		fo2nd 6213	. . . . . . . . . . 11 |- 2nd : _V -onto-> _V
9		fofn 5479	. . . . . . . . . . 11 |- ( 2nd : _V -onto-> _V -> 2nd Fn _V )
10	8, 9	ax-mp 5	. . . . . . . . . 10 |- 2nd Fn _V
11		vex 2763	. . . . . . . . . 10 |- x e. _V
12		fnbrfvb 5598	. . . . . . . . . 10 |- ( ( 2nd Fn _V /\ x e. _V ) -> ( ( 2nd ` x ) = y <-> x 2nd y ) )
13	10, 11, 12	mp2an 426	. . . . . . . . 9 |- ( ( 2nd ` x ) = y <-> x 2nd y )
14	13	anbi1i 458	. . . . . . . 8 |- ( ( ( 2nd ` x ) = y /\ x e. ( { A } X. B ) ) <-> ( x 2nd y /\ x e. ( { A } X. B ) ) )
15		elxp7 6225	. . . . . . . . . . 11 |- ( x e. ( { A } X. B ) <-> ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. { A } /\ ( 2nd ` x ) e. B ) ) )
16		eleq1 2256	. . . . . . . . . . . . . . 15 |- ( ( 2nd ` x ) = y -> ( ( 2nd ` x ) e. B <-> y e. B ) )
17	16	biimpa 296	. . . . . . . . . . . . . 14 |- ( ( ( 2nd ` x ) = y /\ ( 2nd ` x ) e. B ) -> y e. B )
18	17	adantrl 478	. . . . . . . . . . . . 13 |- ( ( ( 2nd ` x ) = y /\ ( ( 1st ` x ) e. { A } /\ ( 2nd ` x ) e. B ) ) -> y e. B )
19	18	adantrl 478	. . . . . . . . . . . 12 |- ( ( ( 2nd ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. { A } /\ ( 2nd ` x ) e. B ) ) ) -> y e. B )
20		elsni 3637	. . . . . . . . . . . . . 14 |- ( ( 1st ` x ) e. { A } -> ( 1st ` x ) = A )
21		eqopi 6227	. . . . . . . . . . . . . . . 16 |- ( ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) = A /\ ( 2nd ` x ) = y ) ) -> x = <. A , y >. )
22	21	ancom2s 566	. . . . . . . . . . . . . . 15 |- ( ( x e. ( _V X. _V ) /\ ( ( 2nd ` x ) = y /\ ( 1st ` x ) = A ) ) -> x = <. A , y >. )
23	22	an12s 565	. . . . . . . . . . . . . 14 |- ( ( ( 2nd ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( 1st ` x ) = A ) ) -> x = <. A , y >. )
24	20, 23	sylanr2 405	. . . . . . . . . . . . 13 |- ( ( ( 2nd ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( 1st ` x ) e. { A } ) ) -> x = <. A , y >. )
25	24	adantrrr 487	. . . . . . . . . . . 12 |- ( ( ( 2nd ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. { A } /\ ( 2nd ` x ) e. B ) ) ) -> x = <. A , y >. )
26	19, 25	jca 306	. . . . . . . . . . 11 |- ( ( ( 2nd ` x ) = y /\ ( x e. ( _V X. _V ) /\ ( ( 1st ` x ) e. { A } /\ ( 2nd ` x ) e. B ) ) ) -> ( y e. B /\ x = <. A , y >. ) )
27	15, 26	sylan2b 287	. . . . . . . . . 10 |- ( ( ( 2nd ` x ) = y /\ x e. ( { A } X. B ) ) -> ( y e. B /\ x = <. A , y >. ) )
28	27	adantl 277	. . . . . . . . 9 |- ( ( A e. V /\ ( ( 2nd ` x ) = y /\ x e. ( { A } X. B ) ) ) -> ( y e. B /\ x = <. A , y >. ) )
29		fveq2 5555	. . . . . . . . . . . 12 |- ( x = <. A , y >. -> ( 2nd ` x ) = ( 2nd ` <. A , y >. ) )
30		op2ndg 6206	. . . . . . . . . . . . 13 |- ( ( A e. V /\ y e. _V ) -> ( 2nd ` <. A , y >. ) = y )
31	6, 30	mpan2 425	. . . . . . . . . . . 12 |- ( A e. V -> ( 2nd ` <. A , y >. ) = y )
32	29, 31	sylan9eqr 2248	. . . . . . . . . . 11 |- ( ( A e. V /\ x = <. A , y >. ) -> ( 2nd ` x ) = y )
33	32	adantrl 478	. . . . . . . . . 10 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> ( 2nd ` x ) = y )
34		simprr 531	. . . . . . . . . . 11 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> x = <. A , y >. )
35		snidg 3648	. . . . . . . . . . . . 13 |- ( A e. V -> A e. { A } )
36	35	adantr 276	. . . . . . . . . . . 12 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> A e. { A } )
37		simprl 529	. . . . . . . . . . . 12 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> y e. B )
38		opelxpi 4692	. . . . . . . . . . . 12 |- ( ( A e. { A } /\ y e. B ) -> <. A , y >. e. ( { A } X. B ) )
39	36, 37, 38	syl2anc 411	. . . . . . . . . . 11 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> <. A , y >. e. ( { A } X. B ) )
40	34, 39	eqeltrd 2270	. . . . . . . . . 10 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> x e. ( { A } X. B ) )
41	33, 40	jca 306	. . . . . . . . 9 |- ( ( A e. V /\ ( y e. B /\ x = <. A , y >. ) ) -> ( ( 2nd ` x ) = y /\ x e. ( { A } X. B ) ) )
42	28, 41	impbida 596	. . . . . . . 8 |- ( A e. V -> ( ( ( 2nd ` x ) = y /\ x e. ( { A } X. B ) ) <-> ( y e. B /\ x = <. A , y >. ) ) )
43	14, 42	bitr3id 194	. . . . . . 7 |- ( A e. V -> ( ( x 2nd y /\ x e. ( { A } X. B ) ) <-> ( y e. B /\ x = <. A , y >. ) ) )
44	7, 43	bitrid 192	. . . . . 6 |- ( A e. V -> ( x ( 2nd |` ( { A } X. B ) ) y <-> ( y e. B /\ x = <. A , y >. ) ) )
45	44	mobidv 2078	. . . . 5 |- ( A e. V -> ( E* x x ( 2nd |` ( { A } X. B ) ) y <-> E* x ( y e. B /\ x = <. A , y >. ) ) )
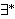
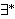46	5, 45	mpbiri 168	. . . 4 |- ( A e. V -> E* x x ( 2nd |` ( { A } X. B ) ) y )
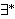47	46	alrimiv 1885	. . 3 |- ( A e. V -> A. y E* x x ( 2nd |` ( { A } X. B ) ) y )
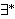
48		funcnv2 5315	. . 3 |- ( Fun `' ( 2nd |` ( { A } X. B ) ) <-> A. y E* x x ( 2nd |` ( { A } X. B ) ) y )
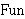
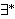
49	47, 48	sylibr 134	. 2 |- ( A e. V -> Fun `' ( 2nd |` ( { A } X. B ) ) )
50		dff1o3 5507	. 2 |- ( ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -1-1-onto-> B <-> ( ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -onto-> B /\ Fun `' ( 2nd |` ( { A } X. B ) ) ) )
51	3, 49, 50	sylanbrc 417	1 |- ( A e. V -> ( 2nd |` ( { A } X. B ) ) : ( { A } X. B ) -1-1-onto-> B )

Syntax hints:   -> wi 4   /\ wa 104   <-> wb 105   A.wal 1362   = wceq 1364   E.wex 1503   E*wmo 2043   e. wcel 2164   _Vcvv 2760   {csn 3619   <.cop 3622   class class class wbr 4030   X. cxp 4658   `'ccnv 4659   |` cres 4662   Fun wfun 5249   Fn wfn 5250   -onto->wfo 5253   -1-1-onto->wf1o 5254   ` cfv 5255   1stc1st 6193   2ndc2nd 6194

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-sep 4148  ax-pow 4204  ax-pr 4239  ax-un 4465

This theorem depends on definitions:  df-bi 117  df-3an 982  df-tru 1367  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ral 2477  df-rex 2478  df-rab 2481  df-v 2762  df-sbc 2987  df-csb 3082  df-un 3158  df-in 3160  df-ss 3167  df-pw 3604  df-sn 3625  df-pr 3626  df-op 3628  df-uni 3837  df-iun 3915  df-br 4031  df-opab 4092  df-mpt 4093  df-id 4325  df-xp 4666  df-rel 4667  df-cnv 4668  df-co 4669  df-dm 4670  df-rn 4671  df-res 4672  df-ima 4673  df-iota 5216  df-fun 5257  df-fn 5258  df-f 5259  df-f1 5260  df-fo 5261  df-f1o 5262  df-fv 5263  df-1st 6195  df-2nd 6196

This theorem is referenced by:  xpfi  6988  fsum2dlemstep  11580  fprod2dlemstep  11768