| Step | Hyp | Ref
| Expression |
|---|
| 1 | | updjud.a |
. . . . . 6
        |
| 2 | | updjud.b |
. . . . . 6
        |
| 3 | 1, 2 | jca 306 |
. . . . 5
         
    |
| 4 | | djuex 7104 |
. . . . 5
        
     ⊔ ⊔
     |
| 5 | | mptexg 5784 |
. . . . 5
    ⊔ ⊔           ⊔ ⊔                                      |
| 6 | 3, 4, 5 | 3syl 17 |
. . . 4
         ⊔ ⊔                                      |
| 7 | | feq1 5387 |
. . . . . . 7
         ⊔ ⊔
                                        ⊔ ⊔     
     ⊔ ⊔
                                     ⊔ ⊔
      |
| 8 | | coeq1 4820 |
. . . . . . . 8
         ⊔ ⊔
                                       inl inl            ⊔ ⊔
                                    inl inl      |
| 9 | 8 | eqeq1d 2202 |
. . . . . . 7
         ⊔ ⊔
                                        inl inl       
      ⊔ ⊔                                     inl inl         |
| 10 | | coeq1 4820 |
. . . . . . . 8
         ⊔ ⊔
                                       inr inr            ⊔ ⊔
                                    inr inr      |
| 11 | 10 | eqeq1d 2202 |
. . . . . . 7
         ⊔ ⊔
                                        inr inr       
      ⊔ ⊔                                     inr inr         |
| 12 | 7, 9, 11 | 3anbi123d 1323 |
. . . . . 6
         ⊔ ⊔
                                         ⊔ ⊔
         inl inl            inr inr               ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr          |
| 13 | | eqeq1 2200 |
. . . . . . . 8
         ⊔ ⊔
                                             ⊔ ⊔                                       |
| 14 | 13 | imbi2d 230 |
. . . . . . 7
         ⊔ ⊔
                                          ⊔ ⊔          inl inl            inr inr             
      ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                       |
| 15 | 14 | ralbidv 2494 |
. . . . . 6
         ⊔ ⊔
                                              ⊔ ⊔          inl inl            inr inr             
          ⊔ ⊔          inl inl            inr inr              ⊔ ⊔                                        |
| 16 | 12, 15 | anbi12d 473 |
. . . . 5
         ⊔ ⊔
                                          ⊔ ⊔          inl inl         
  inr inr           
       ⊔ ⊔          inl inl            inr inr              
       ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr           
       ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                        |
| 17 | 16 | adantl 277 |
. . . 4
    
   
   ⊔ ⊔                                            ⊔ ⊔          inl inl         
  inr inr           
       ⊔ ⊔          inl inl            inr inr              
       ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr           
       ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                        |
| 18 | | updjud.f |
. . . . . 6
          |
| 19 | | updjud.g |
. . . . . 6
          |
| 20 | | eqid 2193 |
. . . . . 6
      ⊔ ⊔                                         ⊔ ⊔                                   |
| 21 | 18, 19, 20 | updjudhf 7140 |
. . . . 5
         ⊔ ⊔                                      ⊔ ⊔      |
| 22 | 18, 19, 20 | updjudhcoinlf 7141 |
. . . . 5
          ⊔ ⊔
                                    inl inl        |
| 23 | 18, 19, 20 | updjudhcoinrg 7142 |
. . . . 5
          ⊔ ⊔
                                    inr inr        |
| 24 | | simpr 110 |
. . . . . . 7
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr                ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr         |
| 25 | | eqeq2 2203 |
. . . . . . . . . . . . . . . . . . . . . 22
        ⊔ ⊔                                     inl inl       
     inl inl            ⊔ ⊔                                     inl inl     
    inl inl         |
| 26 | | fvres 5579 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
        inl inl         inl inl    |
| 27 | 26 | eqcomd 2199 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
       inl inl      inl inl        |
| 28 | 27 | eqeq2d 2205 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
          inl inl   
    inl inl
        |
| 29 | 28 | adantl 277 |
. . . . . . . . . . . . . . . . . . . . . . . . . 26
          ⊔ ⊔                                     inl inl          inl inl          
       inl inl   
    inl inl
        |
| 30 | | fveq1 5554 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
        ⊔ ⊔                                     inl inl          inl inl             ⊔ ⊔                                     inl inl              inl inl         |
| 31 | 30 | ad2antrr 488 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inl inl          inl inl          
          ⊔ ⊔                                     inl inl              inl inl         |
| 32 | | inlresf1 7122 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
  inl inl         ⊔ ⊔   |
| 33 | | f1fn 5462 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
   inl inl         ⊔ ⊔     inl inl       |
| 34 | 32, 33 | mp1i 10 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
         ⊔ ⊔
                                    inl inl          inl inl          inl inl       |
| 35 | | fvco2 5627 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
    inl inl                   ⊔ ⊔
                                    inl inl               ⊔ ⊔
                                     inl inl
        |
| 36 | 34, 35 | sylan 283 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inl inl          inl inl          
          ⊔ ⊔                                     inl inl               ⊔ ⊔
                                     inl inl
        |
| 37 | | fvco2 5627 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
    inl inl                 inl inl              inl inl         |
| 38 | 34, 37 | sylan 283 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inl inl          inl inl          
        inl inl              inl inl         |
| 39 | 31, 36, 38 | 3eqtr3d 2234 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
          ⊔ ⊔                                     inl inl          inl inl          
         ⊔ ⊔
                                     inl inl
             inl inl
        |
| 40 | | fveq2 5555 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
      inl inl              ⊔ ⊔
                                            ⊔ ⊔                                      inl inl         |
| 41 | | fveq2 5555 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
      inl inl                   inl inl         |
| 42 | 40, 41 | eqeq12d 2208 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
      inl inl               ⊔ ⊔                                                   ⊔ ⊔
                                     inl inl
             inl inl
         |
| 43 | 39, 42 | syl5ibrcom 157 |
. . . . . . . . . . . . . . . . . . . . . . . . . 26
          ⊔ ⊔                                     inl inl          inl inl          
        inl inl              ⊔ ⊔                                              |
| 44 | 29, 43 | sylbid 150 |
. . . . . . . . . . . . . . . . . . . . . . . . 25
          ⊔ ⊔                                     inl inl          inl inl          
       inl inl          ⊔ ⊔
                                             |
| 45 | 44 | expimpd 363 |
. . . . . . . . . . . . . . . . . . . . . . . 24
         ⊔ ⊔
                                    inl inl          inl inl                  inl inl    
      ⊔ ⊔                                              |
| 46 | 45 | ex 115 |
. . . . . . . . . . . . . . . . . . . . . . 23
        ⊔ ⊔                                     inl inl          inl inl       
        
 inl inl           ⊔ ⊔                                               |
| 47 | 46 | eqcoms 2196 |
. . . . . . . . . . . . . . . . . . . . . 22
      inl inl            ⊔ ⊔                                     inl inl       
        
 inl inl           ⊔ ⊔                                               |
| 48 | 25, 47 | biimtrrdi 164 |
. . . . . . . . . . . . . . . . . . . . 21
        ⊔ ⊔                                     inl inl       
     inl inl         
        
 inl inl           ⊔ ⊔                                                |
| 49 | 48 | com23 78 |
. . . . . . . . . . . . . . . . . . . 20
        ⊔ ⊔                                     inl inl       
        inl inl              
  inl inl           ⊔ ⊔
                                               |
| 50 | 49 | 3ad2ant2 1021 |
. . . . . . . . . . . . . . . . . . 19
        ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr                 inl inl                 inl inl    
      ⊔ ⊔                                                |
| 51 | 50 | impcom 125 |
. . . . . . . . . . . . . . . . . 18
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               inl inl              
  inl inl           ⊔ ⊔
                                              |
| 52 | 51 | com12 30 |
. . . . . . . . . . . . . . . . 17
      inl inl                  ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr                   inl inl    
      ⊔ ⊔                                               |
| 53 | 52 | 3ad2ant2 1021 |
. . . . . . . . . . . . . . . 16
       ⊔ ⊔          inl inl            inr inr                   ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr                   inl inl    
      ⊔ ⊔                                               |
| 54 | 53 | impcom 125 |
. . . . . . . . . . . . . . 15
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
       
 inl inl           ⊔ ⊔                                              |
| 55 | 54 | com12 30 |
. . . . . . . . . . . . . 14
        
 inl inl                ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                              |
| 56 | 55 | rexlimiva 2606 |
. . . . . . . . . . . . 13
         inl inl   
   
       ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                              |
| 57 | | eqeq2 2203 |
. . . . . . . . . . . . . . . . . . . . . 22
        ⊔ ⊔                                     inr inr       
     inr inr            ⊔ ⊔                                     inr inr     
    inr inr         |
| 58 | | fvres 5579 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
        inr inr         inr inr    |
| 59 | 58 | eqcomd 2199 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
       inr inr      inr inr        |
| 60 | 59 | eqeq2d 2205 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
          inr inr   
    inr inr
        |
| 61 | 60 | adantl 277 |
. . . . . . . . . . . . . . . . . . . . . . . . . 26
          ⊔ ⊔                                     inr inr          inr inr          
       inr inr   
    inr inr
        |
| 62 | | fveq1 5554 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
        ⊔ ⊔                                     inr inr          inr inr             ⊔ ⊔                                     inr inr              inr inr         |
| 63 | 62 | ad2antrr 488 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inr inr          inr inr          
          ⊔ ⊔                                     inr inr              inr inr         |
| 64 | | inrresf1 7123 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
  inr inr         ⊔ ⊔   |
| 65 | | f1fn 5462 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . .
30
   inr inr         ⊔ ⊔     inr inr       |
| 66 | 64, 65 | mp1i 10 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
         ⊔ ⊔
                                    inr inr          inr inr          inr inr       |
| 67 | | fvco2 5627 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
    inr inr                   ⊔ ⊔
                                    inr inr               ⊔ ⊔
                                     inr inr
        |
| 68 | 66, 67 | sylan 283 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inr inr          inr inr          
          ⊔ ⊔                                     inr inr               ⊔ ⊔
                                     inr inr
        |
| 69 | | fvco2 5627 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . . 29
    inr inr                 inr inr              inr inr         |
| 70 | 66, 69 | sylan 283 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
          ⊔ ⊔                                     inr inr          inr inr          
        inr inr              inr inr         |
| 71 | 63, 68, 70 | 3eqtr3d 2234 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
          ⊔ ⊔                                     inr inr          inr inr          
         ⊔ ⊔
                                     inr inr
             inr inr
        |
| 72 | | fveq2 5555 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
      inr inr              ⊔ ⊔
                                            ⊔ ⊔                                      inr inr         |
| 73 | | fveq2 5555 |
. . . . . . . . . . . . . . . . . . . . . . . . . . . 28
      inr inr                   inr inr         |
| 74 | 72, 73 | eqeq12d 2208 |
. . . . . . . . . . . . . . . . . . . . . . . . . . 27
      inr inr               ⊔ ⊔                                                   ⊔ ⊔
                                     inr inr
             inr inr
         |
| 75 | 71, 74 | syl5ibrcom 157 |
. . . . . . . . . . . . . . . . . . . . . . . . . 26
          ⊔ ⊔                                     inr inr          inr inr          
        inr inr              ⊔ ⊔                                              |
| 76 | 61, 75 | sylbid 150 |
. . . . . . . . . . . . . . . . . . . . . . . . 25
          ⊔ ⊔                                     inr inr          inr inr          
       inr inr          ⊔ ⊔
                                             |
| 77 | 76 | expimpd 363 |
. . . . . . . . . . . . . . . . . . . . . . . 24
         ⊔ ⊔
                                    inr inr          inr inr                  inr inr    
      ⊔ ⊔                                              |
| 78 | 77 | ex 115 |
. . . . . . . . . . . . . . . . . . . . . . 23
        ⊔ ⊔                                     inr inr          inr inr       
        
 inr inr           ⊔ ⊔                                               |
| 79 | 78 | eqcoms 2196 |
. . . . . . . . . . . . . . . . . . . . . 22
      inr inr            ⊔ ⊔                                     inr inr       
        
 inr inr           ⊔ ⊔                                               |
| 80 | 57, 79 | biimtrrdi 164 |
. . . . . . . . . . . . . . . . . . . . 21
        ⊔ ⊔                                     inr inr       
     inr inr         
        
 inr inr           ⊔ ⊔                                                |
| 81 | 80 | com23 78 |
. . . . . . . . . . . . . . . . . . . 20
        ⊔ ⊔                                     inr inr       
        inr inr              
  inr inr           ⊔ ⊔
                                               |
| 82 | 81 | 3ad2ant3 1022 |
. . . . . . . . . . . . . . . . . . 19
        ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr                 inr inr                 inr inr    
      ⊔ ⊔                                                |
| 83 | 82 | impcom 125 |
. . . . . . . . . . . . . . . . . 18
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               inr inr              
  inr inr           ⊔ ⊔
                                              |
| 84 | 83 | com12 30 |
. . . . . . . . . . . . . . . . 17
      inr inr                  ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr                   inr inr    
      ⊔ ⊔                                               |
| 85 | 84 | 3ad2ant3 1022 |
. . . . . . . . . . . . . . . 16
       ⊔ ⊔          inl inl            inr inr                   ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr                   inr inr    
      ⊔ ⊔                                               |
| 86 | 85 | impcom 125 |
. . . . . . . . . . . . . . 15
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
       
 inr inr           ⊔ ⊔                                              |
| 87 | 86 | com12 30 |
. . . . . . . . . . . . . 14
        
 inr inr                ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                              |
| 88 | 87 | rexlimiva 2606 |
. . . . . . . . . . . . 13
         inr inr   
   
       ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                              |
| 89 | 56, 88 | jaoi 717 |
. . . . . . . . . . . 12
        
 inl inl       
   inr inr         
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                              |
| 90 | | djur 7130 |
. . . . . . . . . . . . 13
      ⊔ ⊔            inl inl         
 inr inr     |
| 91 | 90 | biimpi 120 |
. . . . . . . . . . . 12
      ⊔ ⊔        
   inl inl         
 inr inr     |
| 92 | 89, 91 | syl11 31 |
. . . . . . . . . . 11
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
     ⊔ ⊔
         ⊔ ⊔
                                             |
| 93 | 92 | ralrimiv 2566 |
. . . . . . . . . 10
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
     ⊔ ⊔
        ⊔ ⊔
                                            |
| 94 | | ffn 5404 |
. . . . . . . . . . . . 13
       ⊔ ⊔                                      ⊔ ⊔           ⊔ ⊔                                      ⊔ ⊔    |
| 95 | 94 | 3ad2ant1 1020 |
. . . . . . . . . . . 12
        ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr              ⊔ ⊔                                      ⊔ ⊔    |
| 96 | 95 | adantl 277 |
. . . . . . . . . . 11
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔                                      ⊔ ⊔    |
| 97 | | ffn 5404 |
. . . . . . . . . . . 12
      ⊔ ⊔     
    ⊔
⊔    |
| 98 | 97 | 3ad2ant1 1020 |
. . . . . . . . . . 11
       ⊔ ⊔          inl inl            inr inr             ⊔ ⊔    |
| 99 | | eqfnfv 5656 |
. . . . . . . . . . 11
        ⊔ ⊔                                      ⊔ ⊔        ⊔ ⊔
   
      ⊔ ⊔                                       
   ⊔ ⊔         ⊔ ⊔                                              |
| 100 | 96, 98, 99 | syl2an 289 |
. . . . . . . . . 10
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
      ⊔ ⊔                                       
   ⊔ ⊔         ⊔ ⊔                                              |
| 101 | 93, 100 | mpbird 167 |
. . . . . . . . 9
            ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr               ⊔ ⊔          inl inl            inr inr         
     ⊔ ⊔
                                     |
| 102 | 101 | ex 115 |
. . . . . . . 8
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr                ⊔ ⊔
         inl inl            inr inr              ⊔ ⊔
                                      |
| 103 | 102 | ralrimivw 2568 |
. . . . . . 7
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr           
        ⊔ ⊔
         inl inl            inr inr              ⊔ ⊔
                                      |
| 104 | 24, 103 | jca 306 |
. . . . . 6
    
      ⊔ ⊔                                      ⊔ ⊔            ⊔ ⊔
                                    inl inl              ⊔ ⊔                                     inr inr                 ⊔ ⊔                                      ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr           
       ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                       |
| 105 | 104 | ex 115 |
. . . . 5
           ⊔ ⊔                                      ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr                ⊔ ⊔
                                     ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr           
       ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                        |
| 106 | 21, 22, 23, 105 | mp3and 1351 |
. . . 4
           ⊔ ⊔                                      ⊔ ⊔
           ⊔ ⊔                                     inl inl              ⊔ ⊔                                     inr inr           
       ⊔ ⊔          inl inl            inr inr              ⊔ ⊔
                                       |
| 107 | 6, 17, 106 | rspcedvd 2871 |
. . 3
              ⊔ ⊔
         inl inl            inr inr                   ⊔ ⊔          inl inl            inr inr               |
| 108 | | feq1 5387 |
. . . . 5
           ⊔ ⊔     
    ⊔ ⊔
      |
| 109 | | coeq1 4820 |
. . . . . 6
          inl inl          inl inl      |
| 110 | 109 | eqeq1d 2202 |
. . . . 5
           inl inl            inl inl         |
| 111 | | coeq1 4820 |
. . . . . 6
          inr inr          inr inr      |
| 112 | 111 | eqeq1d 2202 |
. . . . 5
           inr inr            inr inr         |
| 113 | 108, 110,
112 | 3anbi123d 1323 |
. . . 4
            ⊔ ⊔       
  inl inl            inr inr              ⊔ ⊔          inl inl            inr inr          |
| 114 | 113 | reu8 2957 |
. . 3
  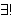         ⊔ ⊔       
  inl inl            inr inr          
        ⊔ ⊔
         inl inl            inr inr                   ⊔ ⊔          inl inl            inr inr               |
| 115 | 107, 114 | sylibr 134 |
. 2
    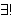         ⊔ ⊔       
  inl inl            inr inr         |
| 116 | | reuv 2779 |
. 2
  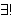         ⊔ ⊔       
  inl inl            inr inr         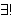       ⊔ ⊔          inl inl         
  inr inr         |
| 117 | 115, 116 | sylib 122 |
1
    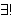       ⊔ ⊔          inl inl         
  inr inr         |