Theorem phpm 6923

Description: Pigeonhole Principle. A natural number is not equinumerous to a proper subset of itself. By "proper subset" here we mean that there is an element which is in the natural number and not in the subset, or in symbols E. x x e. ( A \ B ) (which is stronger than not being equal in the absence of excluded middle). Theorem (Pigeonhole Principle) of [Enderton] p. 134. The theorem is so-called because you can't put n + 1 pigeons into n holes (if each hole holds only one pigeon). The proof consists of lemmas phplem1 6910 through phplem4 6913, nneneq 6915, and this final piece of the proof. (Contributed by NM, 29-May-1998.)

Assertion

Ref	Expression
phpm	|- ( ( A e. om /\ B C_ A /\ E. x x e. ( A \ B ) ) -> -. A ~~ B )

Distinct variable groups:   x, A   x, B

Proof of Theorem phpm

Dummy variable y is distinct from all other variables.

Step	Hyp	Ref	Expression
1		simpr 110	. . . . . 6 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ A = (/) ) -> A = (/) )
2		eldifi 3282	. . . . . . . . 9 |- ( x e. ( A \ B ) -> x e. A )
3		ne0i 3454	. . . . . . . . 9 |- ( x e. A -> A =/= (/) )
4	2, 3	syl 14	. . . . . . . 8 |- ( x e. ( A \ B ) -> A =/= (/) )
5	4	neneqd 2385	. . . . . . 7 |- ( x e. ( A \ B ) -> -. A = (/) )
6	5	ad2antlr 489	. . . . . 6 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ A = (/) ) -> -. A = (/) )
7	1, 6	pm2.21dd 621	. . . . 5 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ A = (/) ) -> -. A ~~ B )
8		php5dom 6921	. . . . . . . . . 10 |- ( y e. om -> -. suc y ~<_ y )
9	8	ad2antlr 489	. . . . . . . . 9 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> -. suc y ~<_ y )
10		simplr 528	. . . . . . . . . 10 |- ( ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) /\ A ~~ B ) -> A = suc y )
11		simpr 110	. . . . . . . . . . 11 |- ( ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) /\ A ~~ B ) -> A ~~ B )
12		vex 2763	. . . . . . . . . . . . . . . 16 |- y e. _V
13	12	sucex 4532	. . . . . . . . . . . . . . 15 |- suc y e. _V
14		difss 3286	. . . . . . . . . . . . . . 15 |- ( suc y \ { x } ) C_ suc y
15	13, 14	ssexi 4168	. . . . . . . . . . . . . 14 |- ( suc y \ { x } ) e. _V
16		eldifn 3283	. . . . . . . . . . . . . . . 16 |- ( x e. ( A \ B ) -> -. x e. B )
17	16	ad3antlr 493	. . . . . . . . . . . . . . 15 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> -. x e. B )
18		simpllr 534	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) -> B C_ A )
19	18	adantr 276	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> B C_ A )
20		simpr 110	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> A = suc y )
21	19, 20	sseqtrd 3218	. . . . . . . . . . . . . . 15 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> B C_ suc y )
22		ssdif 3295	. . . . . . . . . . . . . . . 16 |- ( B C_ suc y -> ( B \ { x } ) C_ ( suc y \ { x } ) )
23		disjsn 3681	. . . . . . . . . . . . . . . . . 18 |- ( ( B i^i { x } ) = (/) <-> -. x e. B )
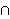
24		disj3 3500	. . . . . . . . . . . . . . . . . 18 |- ( ( B i^i { x } ) = (/) <-> B = ( B \ { x } ) )
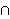25	23, 24	bitr3i 186	. . . . . . . . . . . . . . . . 17 |- ( -. x e. B <-> B = ( B \ { x } ) )
26		sseq1 3203	. . . . . . . . . . . . . . . . 17 |- ( B = ( B \ { x } ) -> ( B C_ ( suc y \ { x } ) <-> ( B \ { x } ) C_ ( suc y \ { x } ) ) )
27	25, 26	sylbi 121	. . . . . . . . . . . . . . . 16 |- ( -. x e. B -> ( B C_ ( suc y \ { x } ) <-> ( B \ { x } ) C_ ( suc y \ { x } ) ) )
28	22, 27	imbitrrid 156	. . . . . . . . . . . . . . 15 |- ( -. x e. B -> ( B C_ suc y -> B C_ ( suc y \ { x } ) ) )
29	17, 21, 28	sylc 62	. . . . . . . . . . . . . 14 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> B C_ ( suc y \ { x } ) )
30		ssdomg 6834	. . . . . . . . . . . . . 14 |- ( ( suc y \ { x } ) e. _V -> ( B C_ ( suc y \ { x } ) -> B ~<_ ( suc y \ { x } ) ) )
31	15, 29, 30	mpsyl 65	. . . . . . . . . . . . 13 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> B ~<_ ( suc y \ { x } ) )
32		simplr 528	. . . . . . . . . . . . . 14 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> y e. om )
33	2	ad3antlr 493	. . . . . . . . . . . . . . 15 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> x e. A )
34	33, 20	eleqtrd 2272	. . . . . . . . . . . . . 14 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> x e. suc y )
35		phplem3g 6914	. . . . . . . . . . . . . . 15 |- ( ( y e. om /\ x e. suc y ) -> y ~~ ( suc y \ { x } ) )
36	35	ensymd 6839	. . . . . . . . . . . . . 14 |- ( ( y e. om /\ x e. suc y ) -> ( suc y \ { x } ) ~~ y )
37	32, 34, 36	syl2anc 411	. . . . . . . . . . . . 13 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> ( suc y \ { x } ) ~~ y )
38		domentr 6847	. . . . . . . . . . . . 13 |- ( ( B ~<_ ( suc y \ { x } ) /\ ( suc y \ { x } ) ~~ y ) -> B ~<_ y )
39	31, 37, 38	syl2anc 411	. . . . . . . . . . . 12 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> B ~<_ y )
40	39	adantr 276	. . . . . . . . . . 11 |- ( ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) /\ A ~~ B ) -> B ~<_ y )
41		endomtr 6846	. . . . . . . . . . 11 |- ( ( A ~~ B /\ B ~<_ y ) -> A ~<_ y )
42	11, 40, 41	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) /\ A ~~ B ) -> A ~<_ y )
43	10, 42	eqbrtrrd 4054	. . . . . . . . 9 |- ( ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) /\ A ~~ B ) -> suc y ~<_ y )
44	9, 43	mtand 666	. . . . . . . 8 |- ( ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) /\ A = suc y ) -> -. A ~~ B )
45	44	ex 115	. . . . . . 7 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ y e. om ) -> ( A = suc y -> -. A ~~ B ) )
46	45	rexlimdva 2611	. . . . . 6 |- ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) -> ( E. y e. om A = suc y -> -. A ~~ B ) )
47	46	imp 124	. . . . 5 |- ( ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) /\ E. y e. om A = suc y ) -> -. A ~~ B )
48		nn0suc 4637	. . . . . 6 |- ( A e. om -> ( A = (/) \/ E. y e. om A = suc y ) )
49	48	ad2antrr 488	. . . . 5 |- ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) -> ( A = (/) \/ E. y e. om A = suc y ) )
50	7, 47, 49	mpjaodan 799	. . . 4 |- ( ( ( A e. om /\ B C_ A ) /\ x e. ( A \ B ) ) -> -. A ~~ B )
51	50	ex 115	. . 3 |- ( ( A e. om /\ B C_ A ) -> ( x e. ( A \ B ) -> -. A ~~ B ) )
52	51	exlimdv 1830	. 2 |- ( ( A e. om /\ B C_ A ) -> ( E. x x e. ( A \ B ) -> -. A ~~ B ) )
53	52	3impia 1202	1 |- ( ( A e. om /\ B C_ A /\ E. x x e. ( A \ B ) ) -> -. A ~~ B )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105   \/ wo 709   /\ w3a 980   = wceq 1364   E.wex 1503   e. wcel 2164   =/= wne 2364   E.wrex 2473   _Vcvv 2760   \ cdif 3151   i^i cin 3153   C_ wss 3154   (/)c0 3447   {csn 3619   class class class wbr 4030   suc csuc 4397   omcom 4623   ~~ cen 6794   ~<_ cdom 6795

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-sep 4148  ax-nul 4156  ax-pow 4204  ax-pr 4239  ax-un 4465  ax-setind 4570  ax-iinf 4621

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-ral 2477  df-rex 2478  df-rab 2481  df-v 2762  df-sbc 2987  df-dif 3156  df-un 3158  df-in 3160  df-ss 3167  df-nul 3448  df-pw 3604  df-sn 3625  df-pr 3626  df-op 3628  df-uni 3837  df-int 3872  df-br 4031  df-opab 4092  df-tr 4129  df-id 4325  df-iord 4398  df-on 4400  df-suc 4403  df-iom 4624  df-xp 4666  df-rel 4667  df-cnv 4668  df-co 4669  df-dm 4670  df-rn 4671  df-res 4672  df-ima 4673  df-iota 5216  df-fun 5257  df-fn 5258  df-f 5259  df-f1 5260  df-fo 5261  df-f1o 5262  df-fv 5263  df-er 6589  df-en 6797  df-dom 6798

This theorem is referenced by:  phpelm  6924