Theorem xnn0xadd0 9933

Description: The sum of two extended nonnegative integers is 0 iff each of the two extended nonnegative integers is 0. (Contributed by AV, 14-Dec-2020.)

Assertion

Ref	Expression
xnn0xadd0	|- ( ( A e. NN0* /\ B e. NN0* ) -> ( ( A +e B ) = 0 <-> ( A = 0 /\ B = 0 ) ) )

Proof of Theorem xnn0xadd0

Step	Hyp	Ref	Expression
1		elxnn0 9305	. . . 4 |- ( A e. NN0* <-> ( A e. NN0 \/ A = +oo ) )
2		elxnn0 9305	. . . . . . 7 |- ( B e. NN0* <-> ( B e. NN0 \/ B = +oo ) )
3		nn0re 9249	. . . . . . . . . . . . 13 |- ( A e. NN0 -> A e. RR )
4		nn0re 9249	. . . . . . . . . . . . 13 |- ( B e. NN0 -> B e. RR )
5		rexadd 9918	. . . . . . . . . . . . 13 |- ( ( A e. RR /\ B e. RR ) -> ( A +e B ) = ( A + B ) )
6	3, 4, 5	syl2an 289	. . . . . . . . . . . 12 |- ( ( A e. NN0 /\ B e. NN0 ) -> ( A +e B ) = ( A + B ) )
7	6	eqeq1d 2202	. . . . . . . . . . 11 |- ( ( A e. NN0 /\ B e. NN0 ) -> ( ( A +e B ) = 0 <-> ( A + B ) = 0 ) )
8		nn0ge0 9265	. . . . . . . . . . . . 13 |- ( A e. NN0 -> 0 <_ A )
9	3, 8	jca 306	. . . . . . . . . . . 12 |- ( A e. NN0 -> ( A e. RR /\ 0 <_ A ) )
10		nn0ge0 9265	. . . . . . . . . . . . 13 |- ( B e. NN0 -> 0 <_ B )
11	4, 10	jca 306	. . . . . . . . . . . 12 |- ( B e. NN0 -> ( B e. RR /\ 0 <_ B ) )
12		add20 8493	. . . . . . . . . . . 12 |- ( ( ( A e. RR /\ 0 <_ A ) /\ ( B e. RR /\ 0 <_ B ) ) -> ( ( A + B ) = 0 <-> ( A = 0 /\ B = 0 ) ) )
13	9, 11, 12	syl2an 289	. . . . . . . . . . 11 |- ( ( A e. NN0 /\ B e. NN0 ) -> ( ( A + B ) = 0 <-> ( A = 0 /\ B = 0 ) ) )
14	7, 13	bitrd 188	. . . . . . . . . 10 |- ( ( A e. NN0 /\ B e. NN0 ) -> ( ( A +e B ) = 0 <-> ( A = 0 /\ B = 0 ) ) )
15	14	biimpd 144	. . . . . . . . 9 |- ( ( A e. NN0 /\ B e. NN0 ) -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) )
16	15	expcom 116	. . . . . . . 8 |- ( B e. NN0 -> ( A e. NN0 -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
17		oveq2 5926	. . . . . . . . . . . . 13 |- ( B = +oo -> ( A +e B ) = ( A +e +oo ) )
18	17	eqeq1d 2202	. . . . . . . . . . . 12 |- ( B = +oo -> ( ( A +e B ) = 0 <-> ( A +e +oo ) = 0 ) )
19	18	adantr 276	. . . . . . . . . . 11 |- ( ( B = +oo /\ A e. NN0 ) -> ( ( A +e B ) = 0 <-> ( A +e +oo ) = 0 ) )
20		nn0xnn0 9307	. . . . . . . . . . . . . 14 |- ( A e. NN0 -> A e. NN0* )
21		xnn0xrnemnf 9315	. . . . . . . . . . . . . 14 |- ( A e. NN0* -> ( A e. RR* /\ A =/= -oo ) )
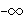22		xaddpnf1 9912	. . . . . . . . . . . . . 14 |- ( ( A e. RR* /\ A =/= -oo ) -> ( A +e +oo ) = +oo )
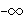23	20, 21, 22	3syl 17	. . . . . . . . . . . . 13 |- ( A e. NN0 -> ( A +e +oo ) = +oo )
24	23	adantl 277	. . . . . . . . . . . 12 |- ( ( B = +oo /\ A e. NN0 ) -> ( A +e +oo ) = +oo )
25	24	eqeq1d 2202	. . . . . . . . . . 11 |- ( ( B = +oo /\ A e. NN0 ) -> ( ( A +e +oo ) = 0 <-> +oo = 0 ) )
26	19, 25	bitrd 188	. . . . . . . . . 10 |- ( ( B = +oo /\ A e. NN0 ) -> ( ( A +e B ) = 0 <-> +oo = 0 ) )
27		0re 8019	. . . . . . . . . . . . 13 |- 0 e. RR
28		renepnf 8067	. . . . . . . . . . . . 13 |- ( 0 e. RR -> 0 =/= +oo )
29	27, 28	ax-mp 5	. . . . . . . . . . . 12 |- 0 =/= +oo
30	29	nesymi 2410	. . . . . . . . . . 11 |- -. +oo = 0
31	30	pm2.21i 647	. . . . . . . . . 10 |- ( +oo = 0 -> ( A = 0 /\ B = 0 ) )
32	26, 31	biimtrdi 163	. . . . . . . . 9 |- ( ( B = +oo /\ A e. NN0 ) -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) )
33	32	ex 115	. . . . . . . 8 |- ( B = +oo -> ( A e. NN0 -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
34	16, 33	jaoi 717	. . . . . . 7 |- ( ( B e. NN0 \/ B = +oo ) -> ( A e. NN0 -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
35	2, 34	sylbi 121	. . . . . 6 |- ( B e. NN0* -> ( A e. NN0 -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
36	35	com12 30	. . . . 5 |- ( A e. NN0 -> ( B e. NN0* -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
37		oveq1 5925	. . . . . . . . 9 |- ( A = +oo -> ( A +e B ) = ( +oo +e B ) )
38	37	eqeq1d 2202	. . . . . . . 8 |- ( A = +oo -> ( ( A +e B ) = 0 <-> ( +oo +e B ) = 0 ) )
39		xnn0xrnemnf 9315	. . . . . . . . . 10 |- ( B e. NN0* -> ( B e. RR* /\ B =/= -oo ) )
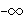40		xaddpnf2 9913	. . . . . . . . . 10 |- ( ( B e. RR* /\ B =/= -oo ) -> ( +oo +e B ) = +oo )
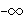41	39, 40	syl 14	. . . . . . . . 9 |- ( B e. NN0* -> ( +oo +e B ) = +oo )
42	41	eqeq1d 2202	. . . . . . . 8 |- ( B e. NN0* -> ( ( +oo +e B ) = 0 <-> +oo = 0 ) )
43	38, 42	sylan9bb 462	. . . . . . 7 |- ( ( A = +oo /\ B e. NN0* ) -> ( ( A +e B ) = 0 <-> +oo = 0 ) )
44	43, 31	biimtrdi 163	. . . . . 6 |- ( ( A = +oo /\ B e. NN0* ) -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) )
45	44	ex 115	. . . . 5 |- ( A = +oo -> ( B e. NN0* -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
46	36, 45	jaoi 717	. . . 4 |- ( ( A e. NN0 \/ A = +oo ) -> ( B e. NN0* -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
47	1, 46	sylbi 121	. . 3 |- ( A e. NN0* -> ( B e. NN0* -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) ) )
48	47	imp 124	. 2 |- ( ( A e. NN0* /\ B e. NN0* ) -> ( ( A +e B ) = 0 -> ( A = 0 /\ B = 0 ) ) )
49		oveq12 5927	. . 3 |- ( ( A = 0 /\ B = 0 ) -> ( A +e B ) = ( 0 +e 0 ) )
50		0xr 8066	. . . 4 |- 0 e. RR*
51		xaddid1 9928	. . . 4 |- ( 0 e. RR* -> ( 0 +e 0 ) = 0 )
52	50, 51	ax-mp 5	. . 3 |- ( 0 +e 0 ) = 0
53	49, 52	eqtrdi 2242	. 2 |- ( ( A = 0 /\ B = 0 ) -> ( A +e B ) = 0 )
54	48, 53	impbid1 142	1 |- ( ( A e. NN0* /\ B e. NN0* ) -> ( ( A +e B ) = 0 <-> ( A = 0 /\ B = 0 ) ) )

Syntax hints:   -> wi 4   /\ wa 104   <-> wb 105   \/ wo 709   = wceq 1364   e. wcel 2164   =/= wne 2364   class class class wbr 4029  (class class class)co 5918   RRcr 7871   0cc0 7872   + caddc 7875   +oocpnf 8051   -oocmnf 8052   RR*cxr 8053   <_ cle 8055   NN0cn0 9240  NN0*cxnn0 9303   +ecxad 9836

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-sep 4147  ax-pow 4203  ax-pr 4238  ax-un 4464  ax-setind 4569  ax-cnex 7963  ax-resscn 7964  ax-1cn 7965  ax-1re 7966  ax-icn 7967  ax-addcl 7968  ax-addrcl 7969  ax-mulcl 7970  ax-addcom 7972  ax-addass 7974  ax-i2m1 7977  ax-0lt1 7978  ax-0id 7980  ax-rnegex 7981  ax-pre-ltirr 7984  ax-pre-ltwlin 7985  ax-pre-lttrn 7986  ax-pre-apti 7987  ax-pre-ltadd 7988

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-nel 2460  df-ral 2477  df-rex 2478  df-rab 2481  df-v 2762  df-sbc 2986  df-dif 3155  df-un 3157  df-in 3159  df-ss 3166  df-if 3558  df-pw 3603  df-sn 3624  df-pr 3625  df-op 3627  df-uni 3836  df-int 3871  df-br 4030  df-opab 4091  df-id 4324  df-xp 4665  df-rel 4666  df-cnv 4667  df-co 4668  df-dm 4669  df-iota 5215  df-fun 5256  df-fv 5262  df-ov 5921  df-oprab 5922  df-mpo 5923  df-pnf 8056  df-mnf 8057  df-xr 8058  df-ltxr 8059  df-le 8060  df-inn 8983  df-n0 9241  df-xnn0 9304  df-xadd 9839

This theorem is referenced by: (None)