Proof of Theorem indpi
| Step | Hyp | Ref
| Expression |
|---|
| 1 | | indpi.4 |
. 2
         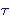   |
| 2 | | elni 7370 |
. . 3
     
          |
| 3 | | eqid 2193 |
. . . . . . . . . 10
    |
| 4 | 3 | orci 732 |
. . . . . . . . 9
          ![]. ].](_drbrack.gif)   |
| 5 | | nfv 1539 |
. . . . . . . . . . 11
      |
| 6 | | nfsbc1v 3005 |
. . . . . . . . . . 11
       ![]. ].](_drbrack.gif)  |
| 7 | 5, 6 | nfor 1585 |
. . . . . . . . . 10
            ![]. ].](_drbrack.gif)   |
| 8 | | 0ex 4157 |
. . . . . . . . . 10
    |
| 9 | | eqeq1 2200 |
. . . . . . . . . . 11
    
    
      |
| 10 | | sbceq1a 2996 |
. . . . . . . . . . 11
    
        ![]. ].](_drbrack.gif)    |
| 11 | 9, 10 | orbi12d 794 |
. . . . . . . . . 10
    
           
       ![]. ].](_drbrack.gif)     |
| 12 | 7, 8, 11 | elabf 2904 |
. . . . . . . . 9
              
          ![]. ].](_drbrack.gif)    |
| 13 | 4, 12 | mpbir 146 |
. . . . . . . 8
         
    |
| 14 | | suceq 4434 |
. . . . . . . . . . . . . 14
    
       |
| 15 | | df-1o 6471 |
. . . . . . . . . . . . . 14
     |
| 16 | 14, 15 | eqtr4di 2244 |
. . . . . . . . . . . . 13
    
      |
| 17 | | indpi.5 |
. . . . . . . . . . . . . . 15
  |
| 18 | 17 | olci 733 |
. . . . . . . . . . . . . 14
    
   |
| 19 | | 1oex 6479 |
. . . . . . . . . . . . . . 15
    |
| 20 | | eqeq1 2200 |
. . . . . . . . . . . . . . . 16
          
     |
| 21 | | indpi.1 |
. . . . . . . . . . . . . . . 16
            |
| 22 | 20, 21 | orbi12d 794 |
. . . . . . . . . . . . . . 15
              
         |
| 23 | 19, 22 | elab 2905 |
. . . . . . . . . . . . . 14
               
        |
| 24 | 18, 23 | mpbir 146 |
. . . . . . . . . . . . 13
         
    |
| 25 | 16, 24 | eqeltrdi 2284 |
. . . . . . . . . . . 12
    
                |
| 26 | 25 | a1d 22 |
. . . . . . . . . . 11
    
                                |
| 27 | 26 | a1i 9 |
. . . . . . . . . 10
                          
        
       |
| 28 | | indpi.6 |
. . . . . . . . . . . 12
            |
| 29 | | elni 7370 |
. . . . . . . . . . . . . . . 16
     
          |
| 30 | 29 | simprbi 275 |
. . . . . . . . . . . . . . 15
          |
| 31 | 30 | neneqd 2385 |
. . . . . . . . . . . . . 14
        
  |
| 32 | | biorf 745 |
. . . . . . . . . . . . . 14
                   |
| 33 | 31, 32 | syl 14 |
. . . . . . . . . . . . 13
                  |
| 34 | | vex 2763 |
. . . . . . . . . . . . . 14
    |
| 35 | | eqeq1 2200 |
. . . . . . . . . . . . . . 15
          
     |
| 36 | | indpi.2 |
. . . . . . . . . . . . . . 15
            |
| 37 | 35, 36 | orbi12d 794 |
. . . . . . . . . . . . . 14
              
         |
| 38 | 34, 37 | elab 2905 |
. . . . . . . . . . . . 13
               
        |
| 39 | 33, 38 | bitr4di 198 |
. . . . . . . . . . . 12
                        |
| 40 | | 1pi 7377 |
. . . . . . . . . . . . . . . . . 18
    |
| 41 | | addclpi 7389 |
. . . . . . . . . . . . . . . . . 18
                 
  |
| 42 | 40, 41 | mpan2 425 |
. . . . . . . . . . . . . . . . 17
              |
| 43 | | elni 7370 |
. . . . . . . . . . . . . . . . 17
         
                  |
| 44 | 42, 43 | sylib 122 |
. . . . . . . . . . . . . . . 16
            
           |
| 45 | 44 | simprd 114 |
. . . . . . . . . . . . . . 15
              |
| 46 | 45 | neneqd 2385 |
. . . . . . . . . . . . . 14
            
  |
| 47 | | biorf 745 |
. . . . . . . . . . . . . 14
        
                  |
| 48 | 46, 47 | syl 14 |
. . . . . . . . . . . . 13
                      |
| 49 | | eqeq1 2200 |
. . . . . . . . . . . . . . . 16
              
         |
| 50 | | indpi.3 |
. . . . . . . . . . . . . . . 16
                |
| 51 | 49, 50 | orbi12d 794 |
. . . . . . . . . . . . . . 15
                  
       
     |
| 52 | 51 | elabg 2907 |
. . . . . . . . . . . . . 14
                
                         |
| 53 | 42, 52 | syl 14 |
. . . . . . . . . . . . 13
            
                         |
| 54 | | addpiord 7378 |
. . . . . . . . . . . . . . . 16
                 
  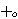    |
| 55 | 40, 54 | mpan2 425 |
. . . . . . . . . . . . . . 15
              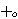    |
| 56 | | pion 7372 |
. . . . . . . . . . . . . . . 16
          |
| 57 | | oa1suc 6522 |
. . . . . . . . . . . . . . . 16
        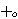       |
| 58 | 56, 57 | syl 14 |
. . . . . . . . . . . . . . 15
        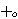       |
| 59 | 55, 58 | eqtrd 2226 |
. . . . . . . . . . . . . 14
               |
| 60 | 59 | eleq1d 2262 |
. . . . . . . . . . . . 13
            
             
              |
| 61 | 48, 53, 60 | 3bitr2d 216 |
. . . . . . . . . . . 12
                  
      |
| 62 | 28, 39, 61 | 3imtr3d 202 |
. . . . . . . . . . 11
                     
        
      |
| 63 | 62 | a1i 9 |
. . . . . . . . . 10
                          
        
       |
| 64 | | nndceq0 4651 |
. . . . . . . . . . . 12
      DECID
DECID 
   |
| 65 | | df-dc 836 |
. . . . . . . . . . . 12
  DECID DECID                |
| 66 | 64, 65 | sylib 122 |
. . . . . . . . . . 11
             
   |
| 67 | | idd 21 |
. . . . . . . . . . . . . . 15
                |
| 68 | 67 | necon3bd 2407 |
. . . . . . . . . . . . . 14
        
        |
| 69 | 68 | anc2li 329 |
. . . . . . . . . . . . 13
        
              |
| 70 | 69, 29 | imbitrrdi 162 |
. . . . . . . . . . . 12
        
        |
| 71 | 70 | orim2d 789 |
. . . . . . . . . . 11
                             |
| 72 | 66, 71 | mpd 13 |
. . . . . . . . . 10
                |
| 73 | 27, 63, 72 | mpjaod 719 |
. . . . . . . . 9
                     
        
      |
| 74 | 73 | rgen 2547 |
. . . . . . . 8
                  
                |
| 75 | | peano5 4631 |
. . . . . . . 8
    
                             
                                |
| 76 | 13, 74, 75 | mp2an 426 |
. . . . . . 7
              |
| 77 | 76 | sseli 3176 |
. . . . . 6
                    |
| 78 | | abid 2181 |
. . . . . 6
               
        |
| 79 | 77, 78 | sylib 122 |
. . . . 5
              |
| 80 | 79 | adantr 276 |
. . . 4
                    |
| 81 | | df-ne 2365 |
. . . . . 6
      
    |
| 82 | | biorf 745 |
. . . . . 6
                   |
| 83 | 81, 82 | sylbi 121 |
. . . . 5
     
            |
| 84 | 83 | adantl 277 |
. . . 4
                        |
| 85 | 80, 84 | mpbird 167 |
. . 3
              |
| 86 | 2, 85 | sylbi 121 |
. 2
        |
| 87 | 1, 86 | vtoclga 2827 |
1
      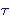  |