Theorem ctmlemr 7167

Description: Lemma for ctm 7168. One of the directions of the biconditional. (Contributed by Jim Kingdon, 16-Mar-2023.)

Assertion

Ref	Expression
ctmlemr	|- ( E. x x e. A -> ( E. f f : om -onto-> A -> E. f f : om -onto-> ( A ⊔ 1o ) ) )

Distinct variable groups:   A, f   x, f

Allowed substitution hint:   A( x)

Proof of Theorem ctmlemr

Dummy variables g n u w y z are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		0lt1o 6493	. . . . . . . . . 10 |- (/) e. 1o
2		djurcl 7111	. . . . . . . . . 10 |- ( (/) e. 1o -> (inr ` (/) ) e. ( A ⊔ 1o ) )
3	1, 2	ax-mp 5	. . . . . . . . 9 |- (inr ` (/) ) e. ( A ⊔ 1o )
4	3	a1i 9	. . . . . . . 8 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ n = (/) ) -> (inr ` (/) ) e. ( A ⊔ 1o ) )
5		simpllr 534	. . . . . . . . . . 11 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ -. n = (/) ) -> f : om -onto-> A )
6		fof 5476	. . . . . . . . . . 11 |- ( f : om -onto-> A -> f : om --> A )
7	5, 6	syl 14	. . . . . . . . . 10 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ -. n = (/) ) -> f : om --> A )
8		nnpredcl 4655	. . . . . . . . . . 11 |- ( n e. om -> U. n e. om )
9	8	ad2antlr 489	. . . . . . . . . 10 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ -. n = (/) ) -> U. n e. om )
10	7, 9	ffvelcdmd 5694	. . . . . . . . 9 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ -. n = (/) ) -> ( f ` U. n ) e. A )
11		djulcl 7110	. . . . . . . . 9 |- ( ( f ` U. n ) e. A -> (inl ` ( f ` U. n ) ) e. ( A ⊔ 1o ) )
12	10, 11	syl 14	. . . . . . . 8 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) /\ -. n = (/) ) -> (inl ` ( f ` U. n ) ) e. ( A ⊔ 1o ) )
13		nndceq0 4650	. . . . . . . . 9 |- ( n e. om -> DECID n = (/) )
14	13	adantl 277	. . . . . . . 8 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) -> DECID n = (/) )
15	4, 12, 14	ifcldadc 3586	. . . . . . 7 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ n e. om ) -> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) e. ( A ⊔ 1o ) )
16	15	fmpttd 5713	. . . . . 6 |- ( ( E. x x e. A /\ f : om -onto-> A ) -> ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om --> ( A ⊔ 1o ) )
17		simpllr 534	. . . . . . . . . . 11 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) -> f : om -onto-> A )
18		simprl 529	. . . . . . . . . . 11 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) -> w e. A )
19		foelrn 5795	. . . . . . . . . . 11 |- ( ( f : om -onto-> A /\ w e. A ) -> E. u e. om w = ( f ` u ) )
20	17, 18, 19	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) -> E. u e. om w = ( f ` u ) )
21		peano2 4627	. . . . . . . . . . . 12 |- ( u e. om -> suc u e. om )
22	21	ad2antrl 490	. . . . . . . . . . 11 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> suc u e. om )
23		simplrr 536	. . . . . . . . . . . . . 14 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> y = (inl ` w ) )
24		simprl 529	. . . . . . . . . . . . . . . . . . 19 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> u e. om )
25		nnord 4644	. . . . . . . . . . . . . . . . . . 19 |- ( u e. om -> Ord u )
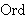26		ordtr 4409	. . . . . . . . . . . . . . . . . . 19 |- ( Ord u -> Tr u )
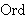
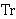27	24, 25, 26	3syl 17	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> Tr u )
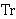
28		unisucg 4445	. . . . . . . . . . . . . . . . . . 19 |- ( u e. om -> ( Tr u <-> U. suc u = u ) )
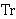
29	28	ad2antrl 490	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> ( Tr u <-> U. suc u = u ) )
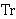30	27, 29	mpbid 147	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> U. suc u = u )
31	30	fveq2d 5558	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> ( f ` U. suc u ) = ( f ` u ) )
32		simprr 531	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> w = ( f ` u ) )
33	31, 32	eqtr4d 2229	. . . . . . . . . . . . . . 15 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> ( f ` U. suc u ) = w )
34	33	fveq2d 5558	. . . . . . . . . . . . . 14 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> (inl ` ( f ` U. suc u ) ) = (inl ` w ) )
35	23, 34	eqtr4d 2229	. . . . . . . . . . . . 13 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> y = (inl ` ( f ` U. suc u ) ) )
36		peano3 4628	. . . . . . . . . . . . . . . 16 |- ( u e. om -> suc u =/= (/) )
37	36	neneqd 2385	. . . . . . . . . . . . . . 15 |- ( u e. om -> -. suc u = (/) )
38	37	ad2antrl 490	. . . . . . . . . . . . . 14 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> -. suc u = (/) )
39	38	iffalsed 3567	. . . . . . . . . . . . 13 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> if ( suc u = (/) , (inr ` (/) ) , (inl ` ( f ` U. suc u ) ) ) = (inl ` ( f ` U. suc u ) ) )
40	35, 39	eqtr4d 2229	. . . . . . . . . . . 12 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> y = if ( suc u = (/) , (inr ` (/) ) , (inl ` ( f ` U. suc u ) ) ) )
41		eqid 2193	. . . . . . . . . . . . 13 |- ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) = ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) )
42		eqeq1 2200	. . . . . . . . . . . . . 14 |- ( n = suc u -> ( n = (/) <-> suc u = (/) ) )
43		unieq 3844	. . . . . . . . . . . . . . . 16 |- ( n = suc u -> U. n = U. suc u )
44	43	fveq2d 5558	. . . . . . . . . . . . . . 15 |- ( n = suc u -> ( f ` U. n ) = ( f ` U. suc u ) )
45	44	fveq2d 5558	. . . . . . . . . . . . . 14 |- ( n = suc u -> (inl ` ( f ` U. n ) ) = (inl ` ( f ` U. suc u ) ) )
46	42, 45	ifbieq2d 3581	. . . . . . . . . . . . 13 |- ( n = suc u -> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) = if ( suc u = (/) , (inr ` (/) ) , (inl ` ( f ` U. suc u ) ) ) )
47		simpllr 534	. . . . . . . . . . . . . 14 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> y e. ( A ⊔ 1o ) )
48	40, 47	eqeltrrd 2271	. . . . . . . . . . . . 13 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> if ( suc u = (/) , (inr ` (/) ) , (inl ` ( f ` U. suc u ) ) ) e. ( A ⊔ 1o ) )
49	41, 46, 22, 48	fvmptd3 5651	. . . . . . . . . . . 12 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` suc u ) = if ( suc u = (/) , (inr ` (/) ) , (inl ` ( f ` U. suc u ) ) ) )
50	40, 49	eqtr4d 2229	. . . . . . . . . . 11 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` suc u ) )
51		fveq2 5554	. . . . . . . . . . . 12 |- ( z = suc u -> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` suc u ) )
52	51	rspceeqv 2882	. . . . . . . . . . 11 |- ( ( suc u e. om /\ y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` suc u ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
53	22, 50, 52	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) /\ ( u e. om /\ w = ( f ` u ) ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
54	20, 53	rexlimddv 2616	. . . . . . . . 9 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. A /\ y = (inl ` w ) ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
55	54	rexlimdvaa 2612	. . . . . . . 8 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) -> ( E. w e. A y = (inl ` w ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) ) )
56		peano1 4626	. . . . . . . . . 10 |- (/) e. om
57		simprr 531	. . . . . . . . . . . . 13 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> y = (inr ` w ) )
58		simprl 529	. . . . . . . . . . . . . . 15 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> w e. 1o )
59		el1o 6490	. . . . . . . . . . . . . . 15 |- ( w e. 1o <-> w = (/) )
60	58, 59	sylib 122	. . . . . . . . . . . . . 14 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> w = (/) )
61	60	fveq2d 5558	. . . . . . . . . . . . 13 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> (inr ` w ) = (inr ` (/) ) )
62	57, 61	eqtrd 2226	. . . . . . . . . . . 12 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> y = (inr ` (/) ) )
63		eqid 2193	. . . . . . . . . . . . 13 |- (/) = (/)
64	63	iftruei 3563	. . . . . . . . . . . 12 |- if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) = (inr ` (/) )
65	62, 64	eqtr4di 2244	. . . . . . . . . . 11 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> y = if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) )
66	64, 3	eqeltri 2266	. . . . . . . . . . . . 13 |- if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) e. ( A ⊔ 1o )
67	66	a1i 9	. . . . . . . . . . . 12 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) e. ( A ⊔ 1o ) )
68		eqeq1 2200	. . . . . . . . . . . . . 14 |- ( n = (/) -> ( n = (/) <-> (/) = (/) ) )
69		unieq 3844	. . . . . . . . . . . . . . . 16 |- ( n = (/) -> U. n = U. (/) )
70	69	fveq2d 5558	. . . . . . . . . . . . . . 15 |- ( n = (/) -> ( f ` U. n ) = ( f ` U. (/) ) )
71	70	fveq2d 5558	. . . . . . . . . . . . . 14 |- ( n = (/) -> (inl ` ( f ` U. n ) ) = (inl ` ( f ` U. (/) ) ) )
72	68, 71	ifbieq2d 3581	. . . . . . . . . . . . 13 |- ( n = (/) -> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) = if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) )
73	72, 41	fvmptg 5633	. . . . . . . . . . . 12 |- ( ( (/) e. om /\ if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) e. ( A ⊔ 1o ) ) -> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` (/) ) = if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) )
74	56, 67, 73	sylancr 414	. . . . . . . . . . 11 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` (/) ) = if ( (/) = (/) , (inr ` (/) ) , (inl ` ( f ` U. (/) ) ) ) )
75	65, 74	eqtr4d 2229	. . . . . . . . . 10 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` (/) ) )
76		fveq2 5554	. . . . . . . . . . 11 |- ( z = (/) -> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` (/) ) )
77	76	rspceeqv 2882	. . . . . . . . . 10 |- ( ( (/) e. om /\ y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` (/) ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
78	56, 75, 77	sylancr 414	. . . . . . . . 9 |- ( ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) /\ ( w e. 1o /\ y = (inr ` w ) ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
79	78	rexlimdvaa 2612	. . . . . . . 8 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) -> ( E. w e. 1o y = (inr ` w ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) ) )
80		djur 7128	. . . . . . . . . 10 |- ( y e. ( A ⊔ 1o ) <-> ( E. w e. A y = (inl ` w ) \/ E. w e. 1o y = (inr ` w ) ) )
81	80	biimpi 120	. . . . . . . . 9 |- ( y e. ( A ⊔ 1o ) -> ( E. w e. A y = (inl ` w ) \/ E. w e. 1o y = (inr ` w ) ) )
82	81	adantl 277	. . . . . . . 8 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) -> ( E. w e. A y = (inl ` w ) \/ E. w e. 1o y = (inr ` w ) ) )
83	55, 79, 82	mpjaod 719	. . . . . . 7 |- ( ( ( E. x x e. A /\ f : om -onto-> A ) /\ y e. ( A ⊔ 1o ) ) -> E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
84	83	ralrimiva 2567	. . . . . 6 |- ( ( E. x x e. A /\ f : om -onto-> A ) -> A. y e. ( A ⊔ 1o ) E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) )
85		dffo3 5705	. . . . . 6 |- ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om -onto-> ( A ⊔ 1o ) <-> ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om --> ( A ⊔ 1o ) /\ A. y e. ( A ⊔ 1o ) E. z e. om y = ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) ` z ) ) )
86	16, 84, 85	sylanbrc 417	. . . . 5 |- ( ( E. x x e. A /\ f : om -onto-> A ) -> ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om -onto-> ( A ⊔ 1o ) )
87		omex 4625	. . . . . . 7 |- om e. _V
88	87	mptex 5784	. . . . . 6 |- ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) e. _V
89		foeq1 5472	. . . . . 6 |- ( g = ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) -> ( g : om -onto-> ( A ⊔ 1o ) <-> ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om -onto-> ( A ⊔ 1o ) ) )
90	88, 89	spcev 2855	. . . . 5 |- ( ( n e. om |-> if ( n = (/) , (inr ` (/) ) , (inl ` ( f ` U. n ) ) ) ) : om -onto-> ( A ⊔ 1o ) -> E. g g : om -onto-> ( A ⊔ 1o ) )
91	86, 90	syl 14	. . . 4 |- ( ( E. x x e. A /\ f : om -onto-> A ) -> E. g g : om -onto-> ( A ⊔ 1o ) )
92	91	ex 115	. . 3 |- ( E. x x e. A -> ( f : om -onto-> A -> E. g g : om -onto-> ( A ⊔ 1o ) ) )
93	92	exlimdv 1830	. 2 |- ( E. x x e. A -> ( E. f f : om -onto-> A -> E. g g : om -onto-> ( A ⊔ 1o ) ) )
94		foeq1 5472	. . 3 |- ( f = g -> ( f : om -onto-> ( A ⊔ 1o ) <-> g : om -onto-> ( A ⊔ 1o ) ) )
95	94	cbvexv 1930	. 2 |- ( E. f f : om -onto-> ( A ⊔ 1o ) <-> E. g g : om -onto-> ( A ⊔ 1o ) )
96	93, 95	imbitrrdi 162	1 |- ( E. x x e. A -> ( E. f f : om -onto-> A -> E. f f : om -onto-> ( A ⊔ 1o ) ) )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105   \/ wo 709  DECID wdc 835   = wceq 1364   E.wex 1503   e. wcel 2164   A.wral 2472   E.wrex 2473   (/)c0 3446   ifcif 3557   U.cuni 3835   |-> cmpt 4090   Tr wtr 4127   Ord word 4393   suc csuc 4396   omcom 4622   -->wf 5250   -onto->wfo 5252   ` cfv 5254   1oc1o 6462   ⊔ cdju 7096  inlcinl 7104  inrcinr 7105

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-coll 4144  ax-sep 4147  ax-nul 4155  ax-pow 4203  ax-pr 4238  ax-un 4464  ax-iinf 4620

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-ral 2477  df-rex 2478  df-reu 2479  df-rab 2481  df-v 2762  df-sbc 2986  df-csb 3081  df-dif 3155  df-un 3157  df-in 3159  df-ss 3166  df-nul 3447  df-if 3558  df-pw 3603  df-sn 3624  df-pr 3625  df-op 3627  df-uni 3836  df-int 3871  df-iun 3914  df-br 4030  df-opab 4091  df-mpt 4092  df-tr 4128  df-id 4324  df-iord 4397  df-on 4399  df-suc 4402  df-iom 4623  df-xp 4665  df-rel 4666  df-cnv 4667  df-co 4668  df-dm 4669  df-rn 4670  df-res 4671  df-ima 4672  df-iota 5215  df-fun 5256  df-fn 5257  df-f 5258  df-f1 5259  df-fo 5260  df-f1o 5261  df-fv 5262  df-1st 6193  df-2nd 6194  df-1o 6469  df-dju 7097  df-inl 7106  df-inr 7107

This theorem is referenced by:  ctm  7168