| Step | Hyp | Ref
| Expression |
|---|
| 1 | | f1od2.2 |
. . . 4
    
   
        
  |
| 2 | 1 | ralrimivva 2576 |
. . 3
         
   
  |
| 3 | | f1od2.1 |
. . . 4
        
     |
| 4 | 3 | fnmpo 6257 |
. . 3
    
   
   
    
    |
| 5 | 2, 4 | syl 14 |
. 2
            |
| 6 | | f1od2.3 |
. . . . . 6
    
           
   |
| 7 | | opelxpi 4692 |
. . . . . 6
        
               |
| 8 | 6, 7 | syl 14 |
. . . . 5
    
         
       |
| 9 | 8 | ralrimiva 2567 |
. . . 4
                    |
| 10 | | eqid 2193 |
. . . . 5
                        |
| 11 | 10 | fnmpt 5381 |
. . . 4
    
                           |
| 12 | 9, 11 | syl 14 |
. . 3
                  |
| 13 | | elxp7 6225 |
. . . . . . . 8
         
                            |
| 14 | 13 | anbi1i 458 |
. . . . . . 7
            
        ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
                                       ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 15 | | anass 401 |
. . . . . . . . 9
                                         ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
                                      ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)     |
| 16 | | f1od2.4 |
. . . . . . . . . . . . 13
                    
   
       
     |
| 17 | 16 | sbcbidv 3045 |
. . . . . . . . . . . 12
             ![]. ].](_drbrack.gif)                
        ![]. ].](_drbrack.gif)                  |
| 18 | 17 | sbcbidv 3045 |
. . . . . . . . . . 11
             ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                
        ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                  |
| 19 | | sbcan 3029 |
. . . . . . . . . . . . . 14
          ![]. ].](_drbrack.gif)       
   
    
         ![]. ].](_drbrack.gif)       
           ![]. ].](_drbrack.gif)  
   |
| 20 | | sbcan 3029 |
. . . . . . . . . . . . . . . 16
          ![]. ].](_drbrack.gif)       
            ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
   |
| 21 | | vex 2763 |
. . . . . . . . . . . . . . . . . . 19
    |
| 22 | | 2ndexg 6223 |
. . . . . . . . . . . . . . . . . . 19
              |
| 23 | 21, 22 | ax-mp 5 |
. . . . . . . . . . . . . . . . . 18
        |
| 24 | | sbcg 3056 |
. . . . . . . . . . . . . . . . . 18
                   ![]. ].](_drbrack.gif)  
 
     |
| 25 | 23, 24 | ax-mp 5 |
. . . . . . . . . . . . . . . . 17
          ![]. ].](_drbrack.gif)   
 
   |
| 26 | | sbcel1v 3049 |
. . . . . . . . . . . . . . . . 17
          ![]. ].](_drbrack.gif)   
         |
| 27 | 25, 26 | anbi12i 460 |
. . . . . . . . . . . . . . . 16
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
                 |
| 28 | 20, 27 | bitri 184 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)       
                 |
| 29 | | sbceq2g 3103 |
. . . . . . . . . . . . . . . 16
                   ![]. ].](_drbrack.gif)  
 
          ![]_ ]_](_urbrack.gif)    |
| 30 | 23, 29 | ax-mp 5 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)   
           ![]_ ]_](_urbrack.gif)   |
| 31 | 28, 30 | anbi12i 460 |
. . . . . . . . . . . . . 14
           ![]. ].](_drbrack.gif)  
   
   
        ![]. ].](_drbrack.gif) 
                             ![]_ ]_](_urbrack.gif)    |
| 32 | 19, 31 | bitri 184 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)       
   
    
                
        ![]_ ]_](_urbrack.gif)    |
| 33 | 32 | sbcbii 3046 |
. . . . . . . . . . . 12
          ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                
        ![]. ].](_drbrack.gif)               
          ![]_ ]_](_urbrack.gif)    |
| 34 | | sbcan 3029 |
. . . . . . . . . . . 12
          ![]. ].](_drbrack.gif)                          ![]_ ]_](_urbrack.gif)             ![]. ].](_drbrack.gif)              
        ![]. ].](_drbrack.gif) 
         ![]_ ]_](_urbrack.gif)    |
| 35 | | sbcan 3029 |
. . . . . . . . . . . . . 14
          ![]. ].](_drbrack.gif)              
         ![]. ].](_drbrack.gif)   
         ![]. ].](_drbrack.gif)          |
| 36 | | sbcel1v 3049 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)   
         |
| 37 | | 1stexg 6222 |
. . . . . . . . . . . . . . . . 17
              |
| 38 | 21, 37 | ax-mp 5 |
. . . . . . . . . . . . . . . 16
        |
| 39 | | sbcg 3056 |
. . . . . . . . . . . . . . . 16
                   ![]. ].](_drbrack.gif)        
         |
| 40 | 38, 39 | ax-mp 5 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)                 |
| 41 | 36, 40 | anbi12i 460 |
. . . . . . . . . . . . . 14
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)         
                  |
| 42 | 35, 41 | bitri 184 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)              
                  |
| 43 | | sbceq2g 3103 |
. . . . . . . . . . . . . 14
                   ![]. ].](_drbrack.gif)  
        ![]_ ]_](_urbrack.gif) 
           ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 44 | 38, 43 | ax-mp 5 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)           ![]_ ]_](_urbrack.gif)             ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   |
| 45 | 42, 44 | anbi12i 460 |
. . . . . . . . . . . 12
           ![]. ].](_drbrack.gif)  
                    ![]. ].](_drbrack.gif) 
         ![]_ ]_](_urbrack.gif)   
                    
        ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 46 | 33, 34, 45 | 3bitri 206 |
. . . . . . . . . . 11
          ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                
                    
        ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 47 | | sbcan 3029 |
. . . . . . . . . . . . . 14
          ![]. ].](_drbrack.gif)       
   
    
         ![]. ].](_drbrack.gif)   
         ![]. ].](_drbrack.gif)            |
| 48 | | sbcg 3056 |
. . . . . . . . . . . . . . . 16
                   ![]. ].](_drbrack.gif)  
 
     |
| 49 | 23, 48 | ax-mp 5 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)   
     |
| 50 | | sbcan 3029 |
. . . . . . . . . . . . . . . 16
          ![]. ].](_drbrack.gif)       
            ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
   |
| 51 | | sbcg 3056 |
. . . . . . . . . . . . . . . . . 18
                   ![]. ].](_drbrack.gif)  
 
     |
| 52 | 23, 51 | ax-mp 5 |
. . . . . . . . . . . . . . . . 17
          ![]. ].](_drbrack.gif)   
 
   |
| 53 | | sbceq1g 3101 |
. . . . . . . . . . . . . . . . . . 19
                   ![]. ].](_drbrack.gif)  
 
        ![]_ ]_](_urbrack.gif) 
    |
| 54 | 23, 53 | ax-mp 5 |
. . . . . . . . . . . . . . . . . 18
          ![]. ].](_drbrack.gif)   
         ![]_ ]_](_urbrack.gif)  
  |
| 55 | | csbvarg 3109 |
. . . . . . . . . . . . . . . . . . . 20
                  ![]_ ]_](_urbrack.gif)         |
| 56 | 23, 55 | ax-mp 5 |
. . . . . . . . . . . . . . . . . . 19
         ![]_ ]_](_urbrack.gif)        |
| 57 | 56 | eqeq1i 2201 |
. . . . . . . . . . . . . . . . . 18
          ![]_ ]_](_urbrack.gif)   
         |
| 58 | 54, 57 | bitri 184 |
. . . . . . . . . . . . . . . . 17
          ![]. ].](_drbrack.gif)   
         |
| 59 | 52, 58 | anbi12i 460 |
. . . . . . . . . . . . . . . 16
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
                 |
| 60 | 50, 59 | bitri 184 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)       
                 |
| 61 | 49, 60 | anbi12i 460 |
. . . . . . . . . . . . . 14
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
   
    
   
                |
| 62 | 47, 61 | bitri 184 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)       
   
    
   
                |
| 63 | 62 | sbcbii 3046 |
. . . . . . . . . . . 12
          ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                
        ![]. ].](_drbrack.gif)                     |
| 64 | | sbcan 3029 |
. . . . . . . . . . . 12
          ![]. ].](_drbrack.gif)       
                      ![]. ].](_drbrack.gif)             ![]. ].](_drbrack.gif)  
             |
| 65 | | sbcg 3056 |
. . . . . . . . . . . . . 14
                   ![]. ].](_drbrack.gif)  
 
     |
| 66 | 38, 65 | ax-mp 5 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)   
     |
| 67 | | sbcan 3029 |
. . . . . . . . . . . . . 14
          ![]. ].](_drbrack.gif)              
         ![]. ].](_drbrack.gif)   
         ![]. ].](_drbrack.gif)          |
| 68 | | sbceq1g 3101 |
. . . . . . . . . . . . . . . . 17
                   ![]. ].](_drbrack.gif)  
 
        ![]_ ]_](_urbrack.gif) 
    |
| 69 | 38, 68 | ax-mp 5 |
. . . . . . . . . . . . . . . 16
          ![]. ].](_drbrack.gif)   
         ![]_ ]_](_urbrack.gif)  
  |
| 70 | | csbvarg 3109 |
. . . . . . . . . . . . . . . . . 18
                  ![]_ ]_](_urbrack.gif)         |
| 71 | 38, 70 | ax-mp 5 |
. . . . . . . . . . . . . . . . 17
         ![]_ ]_](_urbrack.gif)        |
| 72 | 71 | eqeq1i 2201 |
. . . . . . . . . . . . . . . 16
          ![]_ ]_](_urbrack.gif)   
         |
| 73 | 69, 72 | bitri 184 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)   
         |
| 74 | | sbcg 3056 |
. . . . . . . . . . . . . . . 16
                   ![]. ].](_drbrack.gif)        
         |
| 75 | 38, 74 | ax-mp 5 |
. . . . . . . . . . . . . . 15
          ![]. ].](_drbrack.gif)                 |
| 76 | 73, 75 | anbi12i 460 |
. . . . . . . . . . . . . 14
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)         
                  |
| 77 | 67, 76 | bitri 184 |
. . . . . . . . . . . . 13
          ![]. ].](_drbrack.gif)              
                  |
| 78 | 66, 77 | anbi12i 460 |
. . . . . . . . . . . 12
           ![]. ].](_drbrack.gif)  
          ![]. ].](_drbrack.gif)  
                                     |
| 79 | 63, 64, 78 | 3bitri 206 |
. . . . . . . . . . 11
          ![]. ].](_drbrack.gif)         ![]. ].](_drbrack.gif)                
   
                    |
| 80 | 18, 46, 79 | 3bitr3g 222 |
. . . . . . . . . 10
                                  ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
   
                     |
| 81 | 80 | anbi2d 464 |
. . . . . . . . 9
                                           ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    
                                   |
| 82 | 15, 81 | bitrid 192 |
. . . . . . . 8
             
                              ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
                                   |
| 83 | | xpss 4768 |
. . . . . . . . . . . 12
        
   |
| 84 | | simprr 531 |
. . . . . . . . . . . . 13
    
   
                   |
| 85 | 8 | adantrr 479 |
. . . . . . . . . . . . 13
    
   
               
       |
| 86 | 84, 85 | eqeltrd 2270 |
. . . . . . . . . . . 12
    
   
                   |
| 87 | 83, 86 | sselid 3178 |
. . . . . . . . . . 11
    
   
                   |
| 88 | 87 | ex 115 |
. . . . . . . . . 10
                            |
| 89 | 88 | pm4.71rd 394 |
. . . . . . . . 9
                                 
          |
| 90 | | eqop 6232 |
. . . . . . . . . . 11
        
                             |
| 91 | 90 | anbi2d 464 |
. . . . . . . . . 10
        
        
      
   
                     |
| 92 | 91 | pm5.32i 454 |
. . . . . . . . 9
                 
       
                                  |
| 93 | 89, 92 | bitr2di 197 |
. . . . . . . 8
                                            
         |
| 94 | 82, 93 | bitrd 188 |
. . . . . . 7
             
                              ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
   
           |
| 95 | 14, 94 | bitrid 192 |
. . . . . 6
             
          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
   
           |
| 96 | 95 | opabbidv 4096 |
. . . . 5
          
        
          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)           
   
           |
| 97 | | df-mpo 5924 |
. . . . . . . 8
                       
       
   
    |
| 98 | 3, 97 | eqtri 2214 |
. . . . . . 7
          
                   |
| 99 | 98 | cnveqi 4838 |
. . . . . 6
            
                   |
| 100 | | nfv 1539 |
. . . . . . . 8
    
     |
| 101 | | nfcsb1v 3114 |
. . . . . . . . 9
 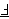          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)  |
| 102 | 101 | nfeq2 2348 |
. . . . . . . 8
    
        ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)  |
| 103 | 100, 102 | nfan 1576 |
. . . . . . 7
                      ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   |
| 104 | | nfv 1539 |
. . . . . . . 8
          |
| 105 | | nfcv 2336 |
. . . . . . . . . 10
 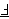       |
| 106 | | nfcsb1v 3114 |
. . . . . . . . . 10
 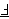          ![]_ ]_](_urbrack.gif)  |
| 107 | 105, 106 | nfcsb 3119 |
. . . . . . . . 9
 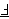          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)  |
| 108 | 107 | nfeq2 2348 |
. . . . . . . 8
             ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)  |
| 109 | 104, 108 | nfan 1576 |
. . . . . . 7
                      ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   |
| 110 | | eleq1 2256 |
. . . . . . . . 9
                                |
| 111 | | opelxp 4690 |
. . . . . . . . 9
                        |
| 112 | 110, 111 | bitrdi 196 |
. . . . . . . 8
                              |
| 113 | | csbopeq1a 6243 |
. . . . . . . . 9
                  ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)  
  |
| 114 | 113 | eqeq2d 2205 |
. . . . . . . 8
                     ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)        |
| 115 | 112, 114 | anbi12d 473 |
. . . . . . 7
                              ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)   
       
   
     |
| 116 | | xpss 4768 |
. . . . . . . . 9
        
   |
| 117 | 116 | sseli 3176 |
. . . . . . . 8
                  |
| 118 | 117 | adantr 276 |
. . . . . . 7
            
        ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)            |
| 119 | 103, 109,
115, 118 | cnvoprab 6289 |
. . . . . 6
         
                          
        
          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 120 | 99, 119 | eqtri 2214 |
. . . . 5
          
        
          ![]_ ]_](_urbrack.gif)         ![]_ ]_](_urbrack.gif)    |
| 121 | | df-mpt 4093 |
. . . . 5
                                  |
| 122 | 96, 120, 121 | 3eqtr4g 2251 |
. . . 4
     
             |
| 123 | 122 | fneq1d 5345 |
. . 3
                         |
| 124 | 12, 123 | mpbird 167 |
. 2
     
   |
| 125 | | dff1o4 5509 |
. 2
                
   
      |
| 126 | 5, 124, 125 | sylanbrc 417 |
1
              |