Theorem frecuzrdgfunlem 10490

Description: The recursive definition generator on upper integers produces a a function. (Contributed by Jim Kingdon, 24-Apr-2022.)

Hypotheses

Ref	Expression
frecuzrdgrclt.c	|- ( ph -> C e. ZZ )
frecuzrdgrclt.a	|- ( ph -> A e. S )
frecuzrdgrclt.t	|- ( ph -> S C_ T )
frecuzrdgrclt.f	|- ( ( ph /\ ( x e. ( ZZ>= ` C ) /\ y e. S ) ) -> ( x F y ) e. S )
frecuzrdgrclt.r	|- R = frec ( ( x e. ( ZZ>= ` C ) , y e. T |-> <. ( x + 1 ) , ( x F y ) >. ) , <. C , A >. )
frecuzrdgfunlem.g	|- G = frec ( ( x e. ZZ |-> ( x + 1 ) ) , C )

Assertion

Ref	Expression
frecuzrdgfunlem	|- ( ph -> Fun ran R )

Distinct variable groups:   x, C, y   x, F, y   x, S, y   x, T, y   ph, x, y   x, G, y   x, R, y

Allowed substitution hints:   A( x, y)

Proof of Theorem frecuzrdgfunlem

Dummy variables z w v are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		frecuzrdgrclt.c	. . . . . 6 |- ( ph -> C e. ZZ )
2		frecuzrdgrclt.a	. . . . . 6 |- ( ph -> A e. S )
3		frecuzrdgrclt.t	. . . . . 6 |- ( ph -> S C_ T )
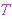4		frecuzrdgrclt.f	. . . . . 6 |- ( ( ph /\ ( x e. ( ZZ>= ` C ) /\ y e. S ) ) -> ( x F y ) e. S )
5		frecuzrdgrclt.r	. . . . . 6 |- R = frec ( ( x e. ( ZZ>= ` C ) , y e. T |-> <. ( x + 1 ) , ( x F y ) >. ) , <. C , A >. )
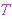6	1, 2, 3, 4, 5	frecuzrdgrclt 10486	. . . . 5 |- ( ph -> R : om --> ( ( ZZ>= ` C ) X. S ) )
7		frn 5412	. . . . 5 |- ( R : om --> ( ( ZZ>= ` C ) X. S ) -> ran R C_ ( ( ZZ>= ` C ) X. S ) )
8	6, 7	syl 14	. . . 4 |- ( ph -> ran R C_ ( ( ZZ>= ` C ) X. S ) )
9		xpss 4767	. . . 4 |- ( ( ZZ>= ` C ) X. S ) C_ ( _V X. _V )
10	8, 9	sstrdi 3191	. . 3 |- ( ph -> ran R C_ ( _V X. _V ) )
11		df-rel 4666	. . 3 |- ( Rel ran R <-> ran R C_ ( _V X. _V ) )
12	10, 11	sylibr 134	. 2 |- ( ph -> Rel ran R )
13		frecuzrdgfunlem.g	. . . . . . . . . 10 |- G = frec ( ( x e. ZZ |-> ( x + 1 ) ) , C )
14	1, 13	frec2uzf1od 10477	. . . . . . . . 9 |- ( ph -> G : om -1-1-onto-> ( ZZ>= ` C ) )
15		f1ocnvdm 5824	. . . . . . . . 9 |- ( ( G : om -1-1-onto-> ( ZZ>= ` C ) /\ v e. ( ZZ>= ` C ) ) -> ( `' G ` v ) e. om )
16	14, 15	sylan 283	. . . . . . . 8 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> ( `' G ` v ) e. om )
17	6	ffvelcdmda 5693	. . . . . . . 8 |- ( ( ph /\ ( `' G ` v ) e. om ) -> ( R ` ( `' G ` v ) ) e. ( ( ZZ>= ` C ) X. S ) )
18	16, 17	syldan 282	. . . . . . 7 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> ( R ` ( `' G ` v ) ) e. ( ( ZZ>= ` C ) X. S ) )
19		xp2nd 6219	. . . . . . 7 |- ( ( R ` ( `' G ` v ) ) e. ( ( ZZ>= ` C ) X. S ) -> ( 2nd ` ( R ` ( `' G ` v ) ) ) e. S )
20	18, 19	syl 14	. . . . . 6 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> ( 2nd ` ( R ` ( `' G ` v ) ) ) e. S )
21		ffn 5403	. . . . . . . . . 10 |- ( R : om --> ( ( ZZ>= ` C ) X. S ) -> R Fn om )
22		fvelrnb 5604	. . . . . . . . . 10 |- ( R Fn om -> ( <. v , z >. e. ran R <-> E. w e. om ( R ` w ) = <. v , z >. ) )
23	6, 21, 22	3syl 17	. . . . . . . . 9 |- ( ph -> ( <. v , z >. e. ran R <-> E. w e. om ( R ` w ) = <. v , z >. ) )
24	6	ffvelcdmda 5693	. . . . . . . . . . . . . . . . . . 19 |- ( ( ph /\ w e. om ) -> ( R ` w ) e. ( ( ZZ>= ` C ) X. S ) )
25		1st2nd2 6228	. . . . . . . . . . . . . . . . . . 19 |- ( ( R ` w ) e. ( ( ZZ>= ` C ) X. S ) -> ( R ` w ) = <. ( 1st ` ( R ` w ) ) , ( 2nd ` ( R ` w ) ) >. )
26	24, 25	syl 14	. . . . . . . . . . . . . . . . . 18 |- ( ( ph /\ w e. om ) -> ( R ` w ) = <. ( 1st ` ( R ` w ) ) , ( 2nd ` ( R ` w ) ) >. )
27	1	adantr 276	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ph /\ w e. om ) -> C e. ZZ )
28	2	adantr 276	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ph /\ w e. om ) -> A e. S )
29	3	adantr 276	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ph /\ w e. om ) -> S C_ T )
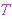30	4	adantlr 477	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ( ph /\ w e. om ) /\ ( x e. ( ZZ>= ` C ) /\ y e. S ) ) -> ( x F y ) e. S )
31		simpr 110	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ph /\ w e. om ) -> w e. om )
32	27, 28, 29, 30, 5, 31, 13	frecuzrdgg 10487	. . . . . . . . . . . . . . . . . . 19 |- ( ( ph /\ w e. om ) -> ( 1st ` ( R ` w ) ) = ( G ` w ) )
33	32	opeq1d 3810	. . . . . . . . . . . . . . . . . 18 |- ( ( ph /\ w e. om ) -> <. ( 1st ` ( R ` w ) ) , ( 2nd ` ( R ` w ) ) >. = <. ( G ` w ) , ( 2nd ` ( R ` w ) ) >. )
34	26, 33	eqtrd 2226	. . . . . . . . . . . . . . . . 17 |- ( ( ph /\ w e. om ) -> ( R ` w ) = <. ( G ` w ) , ( 2nd ` ( R ` w ) ) >. )
35	34	eqeq1d 2202	. . . . . . . . . . . . . . . 16 |- ( ( ph /\ w e. om ) -> ( ( R ` w ) = <. v , z >. <-> <. ( G ` w ) , ( 2nd ` ( R ` w ) ) >. = <. v , z >. ) )
36		vex 2763	. . . . . . . . . . . . . . . . . 18 |- v e. _V
37		vex 2763	. . . . . . . . . . . . . . . . . 18 |- z e. _V
38	36, 37	opth2 4269	. . . . . . . . . . . . . . . . 17 |- ( <. ( G ` w ) , ( 2nd ` ( R ` w ) ) >. = <. v , z >. <-> ( ( G ` w ) = v /\ ( 2nd ` ( R ` w ) ) = z ) )
39	38	simplbi 274	. . . . . . . . . . . . . . . 16 |- ( <. ( G ` w ) , ( 2nd ` ( R ` w ) ) >. = <. v , z >. -> ( G ` w ) = v )
40	35, 39	biimtrdi 163	. . . . . . . . . . . . . . 15 |- ( ( ph /\ w e. om ) -> ( ( R ` w ) = <. v , z >. -> ( G ` w ) = v ) )
41		f1ocnvfv 5822	. . . . . . . . . . . . . . . 16 |- ( ( G : om -1-1-onto-> ( ZZ>= ` C ) /\ w e. om ) -> ( ( G ` w ) = v -> ( `' G ` v ) = w ) )
42	14, 41	sylan 283	. . . . . . . . . . . . . . 15 |- ( ( ph /\ w e. om ) -> ( ( G ` w ) = v -> ( `' G ` v ) = w ) )
43	40, 42	syld 45	. . . . . . . . . . . . . 14 |- ( ( ph /\ w e. om ) -> ( ( R ` w ) = <. v , z >. -> ( `' G ` v ) = w ) )
44		fveq2 5554	. . . . . . . . . . . . . . 15 |- ( ( `' G ` v ) = w -> ( R ` ( `' G ` v ) ) = ( R ` w ) )
45	44	fveq2d 5558	. . . . . . . . . . . . . 14 |- ( ( `' G ` v ) = w -> ( 2nd ` ( R ` ( `' G ` v ) ) ) = ( 2nd ` ( R ` w ) ) )
46	43, 45	syl6 33	. . . . . . . . . . . . 13 |- ( ( ph /\ w e. om ) -> ( ( R ` w ) = <. v , z >. -> ( 2nd ` ( R ` ( `' G ` v ) ) ) = ( 2nd ` ( R ` w ) ) ) )
47	46	imp 124	. . . . . . . . . . . 12 |- ( ( ( ph /\ w e. om ) /\ ( R ` w ) = <. v , z >. ) -> ( 2nd ` ( R ` ( `' G ` v ) ) ) = ( 2nd ` ( R ` w ) ) )
48	36, 37	op2ndd 6202	. . . . . . . . . . . . 13 |- ( ( R ` w ) = <. v , z >. -> ( 2nd ` ( R ` w ) ) = z )
49	48	adantl 277	. . . . . . . . . . . 12 |- ( ( ( ph /\ w e. om ) /\ ( R ` w ) = <. v , z >. ) -> ( 2nd ` ( R ` w ) ) = z )
50	47, 49	eqtr2d 2227	. . . . . . . . . . 11 |- ( ( ( ph /\ w e. om ) /\ ( R ` w ) = <. v , z >. ) -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) )
51	50	ex 115	. . . . . . . . . 10 |- ( ( ph /\ w e. om ) -> ( ( R ` w ) = <. v , z >. -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
52	51	rexlimdva 2611	. . . . . . . . 9 |- ( ph -> ( E. w e. om ( R ` w ) = <. v , z >. -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
53	23, 52	sylbid 150	. . . . . . . 8 |- ( ph -> ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
54	53	alrimiv 1885	. . . . . . 7 |- ( ph -> A. z ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
55	54	adantr 276	. . . . . 6 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> A. z ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
56		eqeq2 2203	. . . . . . . . 9 |- ( w = ( 2nd ` ( R ` ( `' G ` v ) ) ) -> ( z = w <-> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) )
57	56	imbi2d 230	. . . . . . . 8 |- ( w = ( 2nd ` ( R ` ( `' G ` v ) ) ) -> ( ( <. v , z >. e. ran R -> z = w ) <-> ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) ) )
58	57	albidv 1835	. . . . . . 7 |- ( w = ( 2nd ` ( R ` ( `' G ` v ) ) ) -> ( A. z ( <. v , z >. e. ran R -> z = w ) <-> A. z ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) ) )
59	58	spcegv 2848	. . . . . 6 |- ( ( 2nd ` ( R ` ( `' G ` v ) ) ) e. S -> ( A. z ( <. v , z >. e. ran R -> z = ( 2nd ` ( R ` ( `' G ` v ) ) ) ) -> E. w A. z ( <. v , z >. e. ran R -> z = w ) ) )
60	20, 55, 59	sylc 62	. . . . 5 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> E. w A. z ( <. v , z >. e. ran R -> z = w ) )
61		nfv 1539	. . . . . 6 |- F/ w <. v , z >. e. ran R
62	61	mo2r 2094	. . . . 5 |- ( E. w A. z ( <. v , z >. e. ran R -> z = w ) -> E* z <. v , z >. e. ran R )
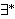63	60, 62	syl 14	. . . 4 |- ( ( ph /\ v e. ( ZZ>= ` C ) ) -> E* z <. v , z >. e. ran R )
64	1, 2, 3, 4, 5	frecuzrdgdom 10489	. . . . . 6 |- ( ph -> dom ran R = ( ZZ>= ` C ) )
65	64	eleq2d 2263	. . . . 5 |- ( ph -> ( v e. dom ran R <-> v e. ( ZZ>= ` C ) ) )
66	65	pm5.32i 454	. . . 4 |- ( ( ph /\ v e. dom ran R ) <-> ( ph /\ v e. ( ZZ>= ` C ) ) )
67		df-br 4030	. . . . 5 |- ( v ran R z <-> <. v , z >. e. ran R )
68	67	mobii 2079	. . . 4 |- ( E* z v ran R z <-> E* z <. v , z >. e. ran R )
69	63, 66, 68	3imtr4i 201	. . 3 |- ( ( ph /\ v e. dom ran R ) -> E* z v ran R z )
70	69	ralrimiva 2567	. 2 |- ( ph -> A. v e. dom ran R E* z v ran R z )
71		dffun7 5281	. 2 |- ( Fun ran R <-> ( Rel ran R /\ A. v e. dom ran R E* z v ran R z ) )
72	12, 70, 71	sylanbrc 417	1 |- ( ph -> Fun ran R )

Syntax hints:   -> wi 4   /\ wa 104   <-> wb 105   A.wal 1362   = wceq 1364   E.wex 1503   E*wmo 2043   e. wcel 2164   A.wral 2472   E.wrex 2473   _Vcvv 2760   C_ wss 3153   <.cop 3621   class class class wbr 4029   |-> cmpt 4090   omcom 4622   X. cxp 4657   `'ccnv 4658   dom cdm 4659   ran crn 4660   Rel wrel 4664   Fun wfun 5248   Fn wfn 5249   -->wf 5250   -1-1-onto->wf1o 5253   ` cfv 5254  (class class class)co 5918   e. cmpo 5920   1stc1st 6191   2ndc2nd 6192  freccfrec 6443   1c1 7873   + caddc 7875   ZZcz 9317   ZZ>=cuz 9592

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-coll 4144  ax-sep 4147  ax-nul 4155  ax-pow 4203  ax-pr 4238  ax-un 4464  ax-setind 4569  ax-iinf 4620  ax-cnex 7963  ax-resscn 7964  ax-1cn 7965  ax-1re 7966  ax-icn 7967  ax-addcl 7968  ax-addrcl 7969  ax-mulcl 7970  ax-addcom 7972  ax-addass 7974  ax-distr 7976  ax-i2m1 7977  ax-0lt1 7978  ax-0id 7980  ax-rnegex 7981  ax-cnre 7983  ax-pre-ltirr 7984  ax-pre-ltwlin 7985  ax-pre-lttrn 7986  ax-pre-ltadd 7988

This theorem depends on definitions:  df-bi 117  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-nel 2460  df-ral 2477  df-rex 2478  df-reu 2479  df-rab 2481  df-v 2762  df-sbc 2986  df-csb 3081  df-dif 3155  df-un 3157  df-in 3159  df-ss 3166  df-nul 3447  df-pw 3603  df-sn 3624  df-pr 3625  df-op 3627  df-uni 3836  df-int 3871  df-iun 3914  df-br 4030  df-opab 4091  df-mpt 4092  df-tr 4128  df-id 4324  df-iord 4397  df-on 4399  df-ilim 4400  df-suc 4402  df-iom 4623  df-xp 4665  df-rel 4666  df-cnv 4667  df-co 4668  df-dm 4669  df-rn 4670  df-res 4671  df-ima 4672  df-iota 5215  df-fun 5256  df-fn 5257  df-f 5258  df-f1 5259  df-fo 5260  df-f1o 5261  df-fv 5262  df-riota 5873  df-ov 5921  df-oprab 5922  df-mpo 5923  df-1st 6193  df-2nd 6194  df-recs 6358  df-frec 6444  df-pnf 8056  df-mnf 8057  df-xr 8058  df-ltxr 8059  df-le 8060  df-sub 8192  df-neg 8193  df-inn 8983  df-n0 9241  df-z 9318  df-uz 9593

This theorem is referenced by:  frecuzrdgfun  10491