| Step | Hyp | Ref
| Expression |
|---|
| 1 | | subrgugrp.1 |
. . . 4
     ↾s
↾s   |
| 2 | | subrgugrp.2 |
. . . 4
    Unit Unit   |
| 3 | | subrgugrp.3 |
. . . 4
    Unit Unit   |
| 4 | 1, 2, 3 | subrguss 13735 |
. . 3
     SubRing SubRing   
    |
| 5 | | subrgrcl 13725 |
. . . 4
     SubRing SubRing   
    |
| 6 | 2 | a1i 9 |
. . . . 5
    
    Unit Unit    |
| 7 | | subrgugrp.4 |
. . . . . 6
     mulGrp mulGrp   ↾s ↾s   |
| 8 | 7 | a1i 9 |
. . . . 5
    
     mulGrp mulGrp   ↾s ↾s    |
| 9 | | ringsrg 13546 |
. . . . 5
    
   SRing SRing |
| 10 | 6, 8, 9 | unitgrpbasd 13614 |
. . . 4
    
         |
| 11 | 5, 10 | syl 14 |
. . 3
     SubRing SubRing   
        |
| 12 | 4, 11 | sseqtrd 3218 |
. 2
     SubRing SubRing   
        |
| 13 | 1 | subrgring 13723 |
. . 3
     SubRing SubRing   
    |
| 14 | | eqid 2193 |
. . . 4
            |
| 15 | 3, 14 | 1unit 13606 |
. . 3
    
         |
| 16 | | elex2 2776 |
. . 3
             
  |
| 17 | 13, 15, 16 | 3syl 17 |
. 2
     SubRing SubRing   
   
  |
| 18 | | eqid 2193 |
. . . . . . . . . . . 12
            |
| 19 | 1, 18 | ressmulrg 12765 |
. . . . . . . . . . 11
      SubRing SubRing   
    
            |
| 20 | 5, 19 | mpdan 421 |
. . . . . . . . . 10
     SubRing SubRing   
            |
| 21 | 20 | 3ad2ant1 1020 |
. . . . . . . . 9
      SubRing SubRing   
        
            |
| 22 | 21 | oveqd 5936 |
. . . . . . . 8
      SubRing SubRing   
        
                    |
| 23 | | eqid 2193 |
. . . . . . . . . 10
            |
| 24 | 3, 23 | unitmulcl 13612 |
. . . . . . . . 9
         
                  |
| 25 | 13, 24 | syl3an1 1282 |
. . . . . . . 8
      SubRing SubRing   
        
            |
| 26 | 22, 25 | eqeltrd 2270 |
. . . . . . 7
      SubRing SubRing   
        
            |
| 27 | 26 | 3expa 1205 |
. . . . . 6
       SubRing SubRing                          |
| 28 | 27 | ralrimiva 2567 |
. . . . 5
      SubRing SubRing   
        
            |
| 29 | | eqid 2193 |
. . . . . . 7
            |
| 30 | | eqid 2193 |
. . . . . . 7
            |
| 31 | 1, 29, 3, 30 | subrginv 13736 |
. . . . . 6
      SubRing SubRing   
                         |
| 32 | 3, 30 | unitinvcl 13622 |
. . . . . . 7
                        |
| 33 | 13, 32 | sylan 283 |
. . . . . 6
      SubRing SubRing   
                 |
| 34 | 31, 33 | eqeltrd 2270 |
. . . . 5
      SubRing SubRing   
                 |
| 35 | 28, 34 | jca 306 |
. . . 4
      SubRing SubRing   
       
                           |
| 36 | 35 | ralrimiva 2567 |
. . 3
     SubRing SubRing   
   
   
                          |
| 37 | | eqid 2193 |
. . . . . . . . . . 11
  mulGrp mulGrp     mulGrp mulGrp   |
| 38 | 37, 18 | mgpplusgg 13423 |
. . . . . . . . . 10
    
           mulGrp mulGrp     |
| 39 | | basfn 12679 |
. . . . . . . . . . . 12
    |
| 40 | | elex 2771 |
. . . . . . . . . . . 12
    
     |
| 41 | | funfvex 5572 |
. . . . . . . . . . . . 13
   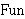 
               |
| 42 | 41 | funfni 5355 |
. . . . . . . . . . . 12
    
               |
| 43 | 39, 40, 42 | sylancr 414 |
. . . . . . . . . . 11
    
         |
| 44 | | eqidd 2194 |
. . . . . . . . . . . 12
    
             |
| 45 | 44, 6, 9 | unitssd 13608 |
. . . . . . . . . . 11
    
         |
| 46 | 43, 45 | ssexd 4170 |
. . . . . . . . . 10
    
     |
| 47 | 37 | ringmgp 13501 |
. . . . . . . . . 10
    
  mulGrp mulGrp    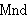  |
| 48 | 8, 38, 46, 47 | ressplusgd 12749 |
. . . . . . . . 9
    
             |
| 49 | 5, 48 | syl 14 |
. . . . . . . 8
     SubRing SubRing   
            |
| 50 | 49 | oveqd 5936 |
. . . . . . 7
     SubRing SubRing   
                    |
| 51 | 50 | eleq1d 2262 |
. . . . . 6
     SubRing SubRing   
            
   
         |
| 52 | 51 | ralbidv 2494 |
. . . . 5
     SubRing SubRing   
   
            
   
   
         |
| 53 | 2 | a1i 9 |
. . . . . . . 8
     SubRing SubRing   
   Unit Unit    |
| 54 | 7 | a1i 9 |
. . . . . . . 8
     SubRing SubRing   
    mulGrp mulGrp   ↾s ↾s    |
| 55 | | eqidd 2194 |
. . . . . . . 8
     SubRing SubRing   
            |
| 56 | 53, 54, 55, 5 | invrfvald 13621 |
. . . . . . 7
     SubRing SubRing   
       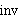      |
| 57 | 56 | fveq1d 5557 |
. . . . . 6
     SubRing SubRing   
            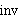         |
| 58 | 57 | eleq1d 2262 |
. . . . 5
     SubRing SubRing   
               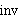            |
| 59 | 52, 58 | anbi12d 473 |
. . . 4
     SubRing SubRing   
   
                          
   
               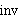             |
| 60 | 59 | ralbidv 2494 |
. . 3
     SubRing SubRing   
   
   
                          
   
   
               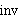             |
| 61 | 36, 60 | mpbid 147 |
. 2
     SubRing SubRing   
   
   
               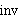            |
| 62 | 2, 7 | unitgrp 13615 |
. . 3
    
   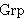  |
| 63 | | eqid 2193 |
. . . 4
            |
| 64 | | eqid 2193 |
. . . 4
            |
| 65 | | eqid 2193 |
. . . 4
  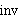       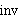     |
| 66 | 63, 64, 65 | issubg2m 13262 |
. . 3
    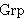    
 SubGrp SubGrp                      
   
               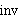              |
| 67 | 5, 62, 66 | 3syl 17 |
. 2
     SubRing SubRing   
    SubGrp SubGrp      
        
                          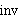              |
| 68 | 12, 17, 61, 67 | mpbir3and 1182 |
1
     SubRing SubRing   
   SubGrp SubGrp    |