| Step | Hyp | Ref
| Expression |
|---|
| 1 | | xpomen 12555 |
. . . . 5
        |
| 2 | 1 | ensymi 6838 |
. . . 4
        |
| 3 | | bren 6803 |
. . . 4
        
             |
| 4 | 2, 3 | mpbi 145 |
. . 3
            |
| 5 | 4 | a1i 9 |
. 2
                |
| 6 | | ctiunct.a |
. . . . . . . 8
          ⊔ ⊔    |
| 7 | | eqid 2193 |
. . . . . . . 8
             inl inl                 inl inl    |
| 8 | | eqid 2193 |
. . . . . . . 8
   inl inl       inl inl    |
| 9 | 6, 7, 8 | ctssdccl 7172 |
. . . . . . 7
                 inl inl    
    inl inl                 inl inl           DECID DECID               inl inl      |
| 10 | 9 | simp1d 1011 |
. . . . . 6
                inl inl       |
| 11 | 10 | adantr 276 |
. . . . 5
    
          
            inl inl    
  |
| 12 | 9 | simp3d 1013 |
. . . . . 6
        DECID DECID               inl inl     |
| 13 | 12 | adantr 276 |
. . . . 5
    
          
    DECID DECID               inl inl     |
| 14 | 9 | simp2d 1012 |
. . . . . 6
      inl inl                 inl inl       |
| 15 | 14 | adantr 276 |
. . . . 5
    
          
  inl inl                 inl inl       |
| 16 | | ctiunct.b |
. . . . . . . 8
    
           ⊔ ⊔    |
| 17 | | eqid 2193 |
. . . . . . . 8
             inl inl                 inl inl    |
| 18 | | eqid 2193 |
. . . . . . . 8
   inl inl       inl inl    |
| 19 | 16, 17, 18 | ctssdccl 7172 |
. . . . . . 7
    
       
          inl inl         inl inl                 inl inl           DECID DECID               inl inl      |
| 20 | 19 | simp1d 1011 |
. . . . . 6
    
                 inl inl    
  |
| 21 | 20 | adantlr 477 |
. . . . 5
                                  inl inl    
  |
| 22 | 19 | simp3d 1013 |
. . . . . 6
    
         DECID DECID               inl inl     |
| 23 | 22 | adantlr 477 |
. . . . 5
                          DECID DECID               inl inl     |
| 24 | 19 | simp2d 1012 |
. . . . . 6
    
       inl inl                 inl inl       |
| 25 | 24 | adantlr 477 |
. . . . 5
                        inl inl                 inl inl       |
| 26 | | simpr 110 |
. . . . 5
    
          
          |
| 27 | | eqid 2193 |
. . . . 5
                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl                                   inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      |
| 28 | 11, 13, 15, 21, 23, 25, 26, 27 | ctiunctlemuom 12596 |
. . . 4
    
          
                            inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
  |
| 29 | | eqid 2193 |
. . . . . 6
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                |
| 30 | | nfv 1539 |
. . . . . . . . 9
                         inl inl    |
| 31 | | nfcsb1v 3114 |
. . . . . . . . . 10
 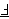      inl inl                 ![]_ ]_](_urbrack.gif)             inl inl    |
| 32 | 31 | nfel2 2349 |
. . . . . . . . 9
                 inl inl
                ![]_ ]_](_urbrack.gif)             inl inl    |
| 33 | 30, 32 | nfan 1576 |
. . . . . . . 8
                          inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl     |
| 34 | | nfcv 2336 |
. . . . . . . 8
 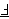   |
| 35 | 33, 34 | nfrabw 2675 |
. . . . . . 7
 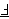                              inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl      |
| 36 | | nfcsb1v 3114 |
. . . . . . . 8
 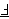      inl inl                 ![]_ ]_](_urbrack.gif)   inl inl    |
| 37 | | nfcv 2336 |
. . . . . . . 8
 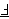           |
| 38 | 36, 37 | nffv 5565 |
. . . . . . 7
 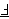       inl inl                 ![]_ ]_](_urbrack.gif)   inl inl               |
| 39 | 35, 38 | nfmpt 4122 |
. . . . . 6
 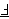                                 inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                |
| 40 | 11, 13, 15, 21, 23, 25, 26, 27, 29, 39, 35 | ctiunctlemfo 12599 |
. . . . 5
    
          
                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl             |
| 41 | | omex 4626 |
. . . . . . . 8
    |
| 42 | 41 | rabex 4174 |
. . . . . . 7
                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl        |
| 43 | 42 | mptex 5785 |
. . . . . 6
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                  |
| 44 | | foeq1 5473 |
. . . . . 6
                                   inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                
                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl                                            inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl              |
| 45 | 43, 44 | spcev 2856 |
. . . . 5
                                 inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl            inl inl
                ![]_ ]_](_urbrack.gif)   inl inl                                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl                                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl             |
| 46 | 40, 45 | syl 14 |
. . . 4
    
          
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl             |
| 47 | 11, 13, 15, 21, 23, 25, 26, 27 | ctiunctlemudc 12597 |
. . . 4
    
          
    DECID DECID                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl       |
| 48 | | sseq1 3203 |
. . . . . 6
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
                                 inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl      
   |
| 49 | | foeq2 5474 |
. . . . . . 7
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
        
 
                              inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl              |
| 50 | 49 | exbidv 1836 |
. . . . . 6
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
                                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl              |
| 51 | | eleq2 2257 |
. . . . . . . 8
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
   
 
                             inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl        |
| 52 | 51 | dcbid 839 |
. . . . . . 7
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
 DECID DECID
    DECID DECID                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl        |
| 53 | 52 | ralbidv 2494 |
. . . . . 6
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
   
 DECID
DECID 
       DECID DECID
                              inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl        |
| 54 | 48, 50, 53 | 3anbi123d 1323 |
. . . . 5
                                inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
   
                  DECID DECID                                   inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl      
                                  inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl                 DECID DECID                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl         |
| 55 | 42, 54 | spcev 2856 |
. . . 4
                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)  
          inl inl      
                                  inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl                 DECID DECID                               inl inl                   inl inl                 ![]_ ]_](_urbrack.gif)             inl inl                               DECID DECID      |
| 56 | 28, 46, 47, 55 | syl3anc 1249 |
. . 3
    
          
                       DECID DECID      |
| 57 | | ctssdc 7174 |
. . . 4
                         DECID DECID       
        
 ⊔ ⊔    |
| 58 | | foeq1 5473 |
. . . . 5
             
   ⊔ ⊔   
        
 ⊔ ⊔     |
| 59 | 58 | cbvexv 1930 |
. . . 4
              ⊔ ⊔   
         
  ⊔ ⊔    |
| 60 | 57, 59 | bitri 184 |
. . 3
                         DECID DECID       
        
 ⊔ ⊔    |
| 61 | 56, 60 | sylib 122 |
. 2
    
          
         
  ⊔ ⊔    |
| 62 | 5, 61 | exlimddv 1910 |
1
              
 ⊔ ⊔    |