Users' Mathboxes Mathbox for Jim Kingdon < Previous   Next >
Nearby theorems
Mirrors  >  Home  >  ILE Home  >  Th. List  >   Mathboxes  >  ismkvnnlem Unicode version
Theorem ismkvnnlem 15696
Description: Lemma for ismkvnn 15697. The result, with a hypothesis to give a name to an expression for convenience. (Contributed by Jim Kingdon, 25-Jun-2024.)
Hypothesis
Ref Expression
ismkvnnlem.g |- G = frec ( ( x e. ZZ |-> ( x + 1 ) ) , 0 )
Assertion
Ref Expression
ismkvnnlem |- ( A e. V -> ( A e. Markov <-> A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) )
Distinct variable groups:   A, f, x   f, G, x   f, V, x
Proof of Theorem ismkvnnlem
Dummy variable g is distinct from all other variables.
StepHypRef Expression
1 ismkvmap 7220 . 2 |- ( A e. V -> ( A e. Markov <-> A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) )
2 nfv 1542 . . . . . . . . 9 |- F/ x A e. V
3 nfcv 2339 . . . . . . . . . 10 |- F/_ x ( 2o ^m A )
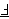4 nfra1 2528 . . . . . . . . . . . 12 |- F/ x A. x e. A ( g ` x ) = 1o
54nfn 1672 . . . . . . . . . . 11 |- F/ x -. A. x e. A ( g ` x ) = 1o
6 nfre1 2540 . . . . . . . . . . 11 |- F/ x E. x e. A ( g ` x ) = (/)
75, 6nfim 1586 . . . . . . . . . 10 |- F/ x ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) )
83, 7nfralxy 2535 . . . . . . . . 9 |- F/ x A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) )
92, 8nfan 1579 . . . . . . . 8 |- F/ x ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) )
10 nfv 1542 . . . . . . . 8 |- F/ x f e. ( { 0 , 1 } ^m A )
119, 10nfan 1579 . . . . . . 7 |- F/ x ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) )
12 ismkvnnlem.g . . . . . . . . . . . 12 |- G = frec ( ( x e. ZZ |-> ( x + 1 ) ) , 0 )
1312frechashgf1o 10520 . . . . . . . . . . 11 |- G : om -1-1-onto-> NN0
14 0nn0 9264 . . . . . . . . . . . . 13 |- 0 e. NN0
15 1nn0 9265 . . . . . . . . . . . . 13 |- 1 e. NN0
16 prssi 3780 . . . . . . . . . . . . 13 |- ( ( 0 e. NN0 /\ 1 e. NN0 ) -> { 0 , 1 } C_ NN0 )
1714, 15, 16mp2an 426 . . . . . . . . . . . 12 |- { 0 , 1 } C_ NN0
18 elmapi 6729 . . . . . . . . . . . . . 14 |- ( f e. ( { 0 , 1 } ^m A ) -> f : A --> { 0 , 1 } )
1918ad2antlr 489 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> f : A --> { 0 , 1 } )
20 simpr 110 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> x e. A )
2119, 20ffvelcdmd 5698 . . . . . . . . . . . 12 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( f ` x ) e. { 0 , 1 } )
2217, 21sselid 3181 . . . . . . . . . . 11 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( f ` x ) e. NN0 )
23 f1ocnvfv2 5825 . . . . . . . . . . 11 |- ( ( G : om -1-1-onto-> NN0 /\ ( f ` x ) e. NN0 ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( f ` x ) )
2413, 22, 23sylancr 414 . . . . . . . . . 10 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( f ` x ) )
2524adantr 276 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( f ` x ) )
26 fvco3 5632 . . . . . . . . . . . . . 14 |- ( ( f : A --> { 0 , 1 } /\ x e. A ) -> ( ( `' G o. f ) ` x ) = ( `' G ` ( f ` x ) ) )
2719, 26sylancom 420 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( ( `' G o. f ) ` x ) = ( `' G ` ( f ` x ) ) )
2827adantr 276 . . . . . . . . . . . 12 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( ( `' G o. f ) ` x ) = ( `' G ` ( f ` x ) ) )
29 simpr 110 . . . . . . . . . . . 12 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( ( `' G o. f ) ` x ) = 1o )
3028, 29eqtr3d 2231 . . . . . . . . . . 11 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( `' G ` ( f ` x ) ) = 1o )
3130fveq2d 5562 . . . . . . . . . 10 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( G ` 1o ) )
32 df-1o 6474 . . . . . . . . . . . 12 |- 1o = suc (/)
3332fveq2i 5561 . . . . . . . . . . 11 |- ( G ` 1o ) = ( G ` suc (/) )
34 0zd 9338 . . . . . . . . . . . . 13 |- ( T. -> 0 e. ZZ )
35 peano1 4630 . . . . . . . . . . . . . 14 |- (/) e. om
3635a1i 9 . . . . . . . . . . . . 13 |- ( T. -> (/) e. om )
3734, 12, 36frec2uzsucd 10493 . . . . . . . . . . . 12 |- ( T. -> ( G ` suc (/) ) = ( ( G ` (/) ) + 1 ) )
3837mptru 1373 . . . . . . . . . . 11 |- ( G ` suc (/) ) = ( ( G ` (/) ) + 1 )
3934, 12frec2uz0d 10491 . . . . . . . . . . . . . 14 |- ( T. -> ( G ` (/) ) = 0 )
4039mptru 1373 . . . . . . . . . . . . 13 |- ( G ` (/) ) = 0
4140oveq1i 5932 . . . . . . . . . . . 12 |- ( ( G ` (/) ) + 1 ) = ( 0 + 1 )
42 0p1e1 9104 . . . . . . . . . . . 12 |- ( 0 + 1 ) = 1
4341, 42eqtri 2217 . . . . . . . . . . 11 |- ( ( G ` (/) ) + 1 ) = 1
4433, 38, 433eqtri 2221 . . . . . . . . . 10 |- ( G ` 1o ) = 1
4531, 44eqtrdi 2245 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( G ` ( `' G ` ( f ` x ) ) ) = 1 )
4625, 45eqtr3d 2231 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = 1o ) -> ( f ` x ) = 1 )
4746ex 115 . . . . . . 7 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( ( ( `' G o. f ) ` x ) = 1o -> ( f ` x ) = 1 ) )
4811, 47ralimdaa 2563 . . . . . 6 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( A. x e. A ( ( `' G o. f ) ` x ) = 1o -> A. x e. A ( f ` x ) = 1 ) )
4948con3d 632 . . . . 5 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( -. A. x e. A ( f ` x ) = 1 -> -. A. x e. A ( ( `' G o. f ) ` x ) = 1o ) )
50 fveq1 5557 . . . . . . . . . 10 |- ( g = ( `' G o. f ) -> ( g ` x ) = ( ( `' G o. f ) ` x ) )
5150eqeq1d 2205 . . . . . . . . 9 |- ( g = ( `' G o. f ) -> ( ( g ` x ) = 1o <-> ( ( `' G o. f ) ` x ) = 1o ) )
5251ralbidv 2497 . . . . . . . 8 |- ( g = ( `' G o. f ) -> ( A. x e. A ( g ` x ) = 1o <-> A. x e. A ( ( `' G o. f ) ` x ) = 1o ) )
5352notbid 668 . . . . . . 7 |- ( g = ( `' G o. f ) -> ( -. A. x e. A ( g ` x ) = 1o <-> -. A. x e. A ( ( `' G o. f ) ` x ) = 1o ) )
5450eqeq1d 2205 . . . . . . . 8 |- ( g = ( `' G o. f ) -> ( ( g ` x ) = (/) <-> ( ( `' G o. f ) ` x ) = (/) ) )
5554rexbidv 2498 . . . . . . 7 |- ( g = ( `' G o. f ) -> ( E. x e. A ( g ` x ) = (/) <-> E. x e. A ( ( `' G o. f ) ` x ) = (/) ) )
5653, 55imbi12d 234 . . . . . 6 |- ( g = ( `' G o. f ) -> ( ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) <-> ( -. A. x e. A ( ( `' G o. f ) ` x ) = 1o -> E. x e. A ( ( `' G o. f ) ` x ) = (/) ) ) )
57 simplr 528 . . . . . 6 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) )
5812012of 15640 . . . . . . . 8 |- ( `' G |` { 0 , 1 } ) : { 0 , 1 } --> 2o
5918adantl 277 . . . . . . . 8 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> f : A --> { 0 , 1 } )
60 fco2 5424 . . . . . . . 8 |- ( ( ( `' G |` { 0 , 1 } ) : { 0 , 1 } --> 2o /\ f : A --> { 0 , 1 } ) -> ( `' G o. f ) : A --> 2o )
6158, 59, 60sylancr 414 . . . . . . 7 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( `' G o. f ) : A --> 2o )
62 2onn 6579 . . . . . . . . 9 |- 2o e. om
6362a1i 9 . . . . . . . 8 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> 2o e. om )
64 simpll 527 . . . . . . . 8 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> A e. V )
6563, 64elmapd 6721 . . . . . . 7 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( ( `' G o. f ) e. ( 2o ^m A ) <-> ( `' G o. f ) : A --> 2o ) )
6661, 65mpbird 167 . . . . . 6 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( `' G o. f ) e. ( 2o ^m A ) )
6756, 57, 66rspcdva 2873 . . . . 5 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( -. A. x e. A ( ( `' G o. f ) ` x ) = 1o -> E. x e. A ( ( `' G o. f ) ` x ) = (/) ) )
6824adantr 276 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = (/) ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( f ` x ) )
6927eqeq1d 2205 . . . . . . . . . . 11 |- ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) -> ( ( ( `' G o. f ) ` x ) = (/) <-> ( `' G ` ( f ` x ) ) = (/) ) )
7069biimpa 296 . . . . . . . . . 10 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = (/) ) -> ( `' G ` ( f ` x ) ) = (/) )
7170fveq2d 5562 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = (/) ) -> ( G ` ( `' G ` ( f ` x ) ) ) = ( G ` (/) ) )
7271, 40eqtrdi 2245 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = (/) ) -> ( G ` ( `' G ` ( f ` x ) ) ) = 0 )
7368, 72eqtr3d 2231 . . . . . . 7 |- ( ( ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) /\ x e. A ) /\ ( ( `' G o. f ) ` x ) = (/) ) -> ( f ` x ) = 0 )
7473exp31 364 . . . . . 6 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( x e. A -> ( ( ( `' G o. f ) ` x ) = (/) -> ( f ` x ) = 0 ) ) )
7511, 74reximdai 2595 . . . . 5 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( E. x e. A ( ( `' G o. f ) ` x ) = (/) -> E. x e. A ( f ` x ) = 0 ) )
7649, 67, 753syld 57 . . . 4 |- ( ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) /\ f e. ( { 0 , 1 } ^m A ) ) -> ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) )
7776ralrimiva 2570 . . 3 |- ( ( A e. V /\ A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) ) -> A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) )
78 nfcv 2339 . . . . . . . . . 10 |- F/_ x ( { 0 , 1 } ^m A )
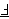79 nfra1 2528 . . . . . . . . . . . 12 |- F/ x A. x e. A ( f ` x ) = 1
8079nfn 1672 . . . . . . . . . . 11 |- F/ x -. A. x e. A ( f ` x ) = 1
81 nfre1 2540 . . . . . . . . . . 11 |- F/ x E. x e. A ( f ` x ) = 0
8280, 81nfim 1586 . . . . . . . . . 10 |- F/ x ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 )
8378, 82nfralxy 2535 . . . . . . . . 9 |- F/ x A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 )
842, 83nfan 1579 . . . . . . . 8 |- F/ x ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) )
85 nfv 1542 . . . . . . . 8 |- F/ x g e. ( 2o ^m A )
8684, 85nfan 1579 . . . . . . 7 |- F/ x ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) )
87 elmapi 6729 . . . . . . . . . . . . . 14 |- ( g e. ( 2o ^m A ) -> g : A --> 2o )
8887ad2antlr 489 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> g : A --> 2o )
89 omelon 4645 . . . . . . . . . . . . . . . 16 |- om e. On
9089onelssi 4464 . . . . . . . . . . . . . . 15 |- ( 2o e. om -> 2o C_ om )
9162, 90ax-mp 5 . . . . . . . . . . . . . 14 |- 2o C_ om
9291a1i 9 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> 2o C_ om )
9388, 92fssd 5420 . . . . . . . . . . . 12 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> g : A --> om )
94 simpr 110 . . . . . . . . . . . 12 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> x e. A )
9593, 94ffvelcdmd 5698 . . . . . . . . . . 11 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( g ` x ) e. om )
96 f1ocnvfv1 5824 . . . . . . . . . . 11 |- ( ( G : om -1-1-onto-> NN0 /\ ( g ` x ) e. om ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( g ` x ) )
9713, 95, 96sylancr 414 . . . . . . . . . 10 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( g ` x ) )
9897adantr 276 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 1 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( g ` x ) )
99 fvco3 5632 . . . . . . . . . . . . . 14 |- ( ( g : A --> 2o /\ x e. A ) -> ( ( G o. g ) ` x ) = ( G ` ( g ` x ) ) )
10088, 99sylancom 420 . . . . . . . . . . . . 13 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( ( G o. g ) ` x ) = ( G ` ( g ` x ) ) )
101100eqeq1d 2205 . . . . . . . . . . . 12 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( ( ( G o. g ) ` x ) = 1 <-> ( G ` ( g ` x ) ) = 1 ) )
102101biimpa 296 . . . . . . . . . . 11 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 1 ) -> ( G ` ( g ` x ) ) = 1 )
103102fveq2d 5562 . . . . . . . . . 10 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 1 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( `' G ` 1 ) )
104 1onn 6578 . . . . . . . . . . . 12 |- 1o e. om
105 f1ocnvfv 5826 . . . . . . . . . . . 12 |- ( ( G : om -1-1-onto-> NN0 /\ 1o e. om ) -> ( ( G ` 1o ) = 1 -> ( `' G ` 1 ) = 1o ) )
10613, 104, 105mp2an 426 . . . . . . . . . . 11 |- ( ( G ` 1o ) = 1 -> ( `' G ` 1 ) = 1o )
10744, 106ax-mp 5 . . . . . . . . . 10 |- ( `' G ` 1 ) = 1o
108103, 107eqtrdi 2245 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 1 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = 1o )
10998, 108eqtr3d 2231 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 1 ) -> ( g ` x ) = 1o )
110109ex 115 . . . . . . 7 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( ( ( G o. g ) ` x ) = 1 -> ( g ` x ) = 1o ) )
11186, 110ralimdaa 2563 . . . . . 6 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( A. x e. A ( ( G o. g ) ` x ) = 1 -> A. x e. A ( g ` x ) = 1o ) )
112111con3d 632 . . . . 5 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( -. A. x e. A ( g ` x ) = 1o -> -. A. x e. A ( ( G o. g ) ` x ) = 1 ) )
113 fveq1 5557 . . . . . . . . . 10 |- ( f = ( G o. g ) -> ( f ` x ) = ( ( G o. g ) ` x ) )
114113eqeq1d 2205 . . . . . . . . 9 |- ( f = ( G o. g ) -> ( ( f ` x ) = 1 <-> ( ( G o. g ) ` x ) = 1 ) )
115114ralbidv 2497 . . . . . . . 8 |- ( f = ( G o. g ) -> ( A. x e. A ( f ` x ) = 1 <-> A. x e. A ( ( G o. g ) ` x ) = 1 ) )
116115notbid 668 . . . . . . 7 |- ( f = ( G o. g ) -> ( -. A. x e. A ( f ` x ) = 1 <-> -. A. x e. A ( ( G o. g ) ` x ) = 1 ) )
117113eqeq1d 2205 . . . . . . . 8 |- ( f = ( G o. g ) -> ( ( f ` x ) = 0 <-> ( ( G o. g ) ` x ) = 0 ) )
118117rexbidv 2498 . . . . . . 7 |- ( f = ( G o. g ) -> ( E. x e. A ( f ` x ) = 0 <-> E. x e. A ( ( G o. g ) ` x ) = 0 ) )
119116, 118imbi12d 234 . . . . . 6 |- ( f = ( G o. g ) -> ( ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) <-> ( -. A. x e. A ( ( G o. g ) ` x ) = 1 -> E. x e. A ( ( G o. g ) ` x ) = 0 ) ) )
120 simplr 528 . . . . . 6 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) )
121122o01f 15641 . . . . . . . 8 |- ( G |` 2o ) : 2o --> { 0 , 1 }
12287adantl 277 . . . . . . . 8 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> g : A --> 2o )
123 fco2 5424 . . . . . . . 8 |- ( ( ( G |` 2o ) : 2o --> { 0 , 1 } /\ g : A --> 2o ) -> ( G o. g ) : A --> { 0 , 1 } )
124121, 122, 123sylancr 414 . . . . . . 7 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( G o. g ) : A --> { 0 , 1 } )
125 prexg 4244 . . . . . . . . . 10 |- ( ( 0 e. NN0 /\ 1 e. NN0 ) -> { 0 , 1 } e. _V )
12614, 15, 125mp2an 426 . . . . . . . . 9 |- { 0 , 1 } e. _V
127126a1i 9 . . . . . . . 8 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> { 0 , 1 } e. _V )
128 simpll 527 . . . . . . . 8 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> A e. V )
129127, 128elmapd 6721 . . . . . . 7 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( ( G o. g ) e. ( { 0 , 1 } ^m A ) <-> ( G o. g ) : A --> { 0 , 1 } ) )
130124, 129mpbird 167 . . . . . 6 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( G o. g ) e. ( { 0 , 1 } ^m A ) )
131119, 120, 130rspcdva 2873 . . . . 5 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( -. A. x e. A ( ( G o. g ) ` x ) = 1 -> E. x e. A ( ( G o. g ) ` x ) = 0 ) )
13297adantr 276 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 0 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( g ` x ) )
133100eqeq1d 2205 . . . . . . . . . . 11 |- ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) -> ( ( ( G o. g ) ` x ) = 0 <-> ( G ` ( g ` x ) ) = 0 ) )
134133biimpa 296 . . . . . . . . . 10 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 0 ) -> ( G ` ( g ` x ) ) = 0 )
135134fveq2d 5562 . . . . . . . . 9 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 0 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = ( `' G ` 0 ) )
136 f1ocnvfv 5826 . . . . . . . . . . 11 |- ( ( G : om -1-1-onto-> NN0 /\ (/) e. om ) -> ( ( G ` (/) ) = 0 -> ( `' G ` 0 ) = (/) ) )
13713, 35, 136mp2an 426 . . . . . . . . . 10 |- ( ( G ` (/) ) = 0 -> ( `' G ` 0 ) = (/) )
13840, 137ax-mp 5 . . . . . . . . 9 |- ( `' G ` 0 ) = (/)
139135, 138eqtrdi 2245 . . . . . . . 8 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 0 ) -> ( `' G ` ( G ` ( g ` x ) ) ) = (/) )
140132, 139eqtr3d 2231 . . . . . . 7 |- ( ( ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) /\ x e. A ) /\ ( ( G o. g ) ` x ) = 0 ) -> ( g ` x ) = (/) )
141140exp31 364 . . . . . 6 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( x e. A -> ( ( ( G o. g ) ` x ) = 0 -> ( g ` x ) = (/) ) ) )
14286, 141reximdai 2595 . . . . 5 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( E. x e. A ( ( G o. g ) ` x ) = 0 -> E. x e. A ( g ` x ) = (/) ) )
143112, 131, 1423syld 57 . . . 4 |- ( ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) /\ g e. ( 2o ^m A ) ) -> ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) )
144143ralrimiva 2570 . . 3 |- ( ( A e. V /\ A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) -> A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) )
14577, 144impbida 596 . 2 |- ( A e. V -> ( A. g e. ( 2o ^m A ) ( -. A. x e. A ( g ` x ) = 1o -> E. x e. A ( g ` x ) = (/) ) <-> A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) )
1461, 145bitrd 188 1 |- ( A e. V -> ( A e. Markov <-> A. f e. ( { 0 , 1 } ^m A ) ( -. A. x e. A ( f ` x ) = 1 -> E. x e. A ( f ` x ) = 0 ) ) )
Colors of variables: wff set class
Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105   = wceq 1364   T. wtru 1365   e. wcel 2167   A.wral 2475   E.wrex 2476   _Vcvv 2763   C_ wss 3157   (/)c0 3450   {cpr 3623   |-> cmpt 4094   suc csuc 4400   omcom 4626   `'ccnv 4662   |` cres 4665   o. ccom 4667   -->wf 5254   -1-1-onto->wf1o 5257   ` cfv 5258  (class class class)co 5922  freccfrec 6448   1oc1o 6467   2oc2o 6468   ^m cmap 6707  Markovcmarkov 7217   0cc0 7879   1c1 7880   + caddc 7882   NN0cn0 9249   ZZcz 9326
This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1461  ax-7 1462  ax-gen 1463  ax-ie1 1507  ax-ie2 1508  ax-8 1518  ax-10 1519  ax-11 1520  ax-i12 1521  ax-bndl 1523  ax-4 1524  ax-17 1540  ax-i9 1544  ax-ial 1548  ax-i5r 1549  ax-13 2169  ax-14 2170  ax-ext 2178  ax-coll 4148  ax-sep 4151  ax-nul 4159  ax-pow 4207  ax-pr 4242  ax-un 4468  ax-setind 4573  ax-iinf 4624  ax-cnex 7970  ax-resscn 7971  ax-1cn 7972  ax-1re 7973  ax-icn 7974  ax-addcl 7975  ax-addrcl 7976  ax-mulcl 7977  ax-addcom 7979  ax-addass 7981  ax-distr 7983  ax-i2m1 7984  ax-0lt1 7985  ax-0id 7987  ax-rnegex 7988  ax-cnre 7990  ax-pre-ltirr 7991  ax-pre-ltwlin 7992  ax-pre-lttrn 7993  ax-pre-ltadd 7995
This theorem depends on definitions:  df-bi 117  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1475  df-sb 1777  df-eu 2048  df-mo 2049  df-clab 2183  df-cleq 2189  df-clel 2192  df-nfc 2328  df-ne 2368  df-nel 2463  df-ral 2480  df-rex 2481  df-reu 2482  df-rab 2484  df-v 2765  df-sbc 2990  df-csb 3085  df-dif 3159  df-un 3161  df-in 3163  df-ss 3170  df-nul 3451  df-pw 3607  df-sn 3628  df-pr 3629  df-op 3631  df-uni 3840  df-int 3875  df-iun 3918  df-br 4034  df-opab 4095  df-mpt 4096  df-tr 4132  df-id 4328  df-iord 4401  df-on 4403  df-ilim 4404  df-suc 4406  df-iom 4627  df-xp 4669  df-rel 4670  df-cnv 4671  df-co 4672  df-dm 4673  df-rn 4674  df-res 4675  df-ima 4676  df-iota 5219  df-fun 5260  df-fn 5261  df-f 5262  df-f1 5263  df-fo 5264  df-f1o 5265  df-fv 5266  df-riota 5877  df-ov 5925  df-oprab 5926  df-mpo 5927  df-recs 6363  df-frec 6449  df-1o 6474  df-2o 6475  df-map 6709  df-markov 7218  df-pnf 8063  df-mnf 8064  df-xr 8065  df-ltxr 8066  df-le 8067  df-sub 8199  df-neg 8200  df-inn 8991  df-n0 9250  df-z 9327  df-uz 9602
This theorem is referenced by:  ismkvnn  15697
  Copyright terms: Public domain W3C validator