Theorem ctinf 12672

Description: A set is countably infinite if and only if it has decidable equality, is countable, and is infinite. (Contributed by Jim Kingdon, 7-Aug-2023.)

Assertion

Ref	Expression
ctinf	|- ( A ~~ NN <-> ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) )

Distinct variable group:   A, f, y, x

Proof of Theorem ctinf

Dummy variables a b n k u g m are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		ctinfom 12670	. . . 4 |- ( A ~~ NN <-> ( A. x e. A A. y e. A DECID x = y /\ E. f ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) ) )
2	1	simplbi 274	. . 3 |- ( A ~~ NN -> A. x e. A A. y e. A DECID x = y )
3	1	simprbi 275	. . . 4 |- ( A ~~ NN -> E. f ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) )
4		simpl 109	. . . . . 6 |- ( ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) -> f : om -onto-> A )
5	4	a1i 9	. . . . 5 |- ( A ~~ NN -> ( ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) -> f : om -onto-> A ) )
6	5	eximdv 1894	. . . 4 |- ( A ~~ NN -> ( E. f ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) -> E. f f : om -onto-> A ) )
7	3, 6	mpd 13	. . 3 |- ( A ~~ NN -> E. f f : om -onto-> A )
8		nnenom 10543	. . . . . 6 |- NN ~~ om
9		entr 6852	. . . . . 6 |- ( ( A ~~ NN /\ NN ~~ om ) -> A ~~ om )
10	8, 9	mpan2 425	. . . . 5 |- ( A ~~ NN -> A ~~ om )
11	10	ensymd 6851	. . . 4 |- ( A ~~ NN -> om ~~ A )
12		endom 6831	. . . 4 |- ( om ~~ A -> om ~<_ A )
13	11, 12	syl 14	. . 3 |- ( A ~~ NN -> om ~<_ A )
14	2, 7, 13	3jca 1179	. 2 |- ( A ~~ NN -> ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) )
15		simp1 999	. . 3 |- ( ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) -> A. x e. A A. y e. A DECID x = y )
16		3simpb 997	. . . 4 |- ( ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) -> ( A. x e. A A. y e. A DECID x = y /\ om ~<_ A ) )
17		simp2 1000	. . . 4 |- ( ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) -> E. f f : om -onto-> A )
18		simp2 1000	. . . . . . . 8 |- ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) -> f : om -onto-> A )
19		simpl1 1002	. . . . . . . . . . . 12 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> A. x e. A A. y e. A DECID x = y )
20		equequ1 1726	. . . . . . . . . . . . . . 15 |- ( x = u -> ( x = y <-> u = y ) )
21	20	dcbid 839	. . . . . . . . . . . . . 14 |- ( x = u -> (DECID x = y <-> DECID u = y ) )
22	21	ralbidv 2497	. . . . . . . . . . . . 13 |- ( x = u -> ( A. y e. A DECID x = y <-> A. y e. A DECID u = y ) )
23	22	cbvralv 2729	. . . . . . . . . . . 12 |- ( A. x e. A A. y e. A DECID x = y <-> A. u e. A A. y e. A DECID u = y )
24	19, 23	sylib 122	. . . . . . . . . . 11 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> A. u e. A A. y e. A DECID u = y )
25		simpl3 1004	. . . . . . . . . . 11 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> om ~<_ A )
26		fof 5483	. . . . . . . . . . . . . 14 |- ( f : om -onto-> A -> f : om --> A )
27		imassrn 5021	. . . . . . . . . . . . . . 15 |- ( f " n ) C_ ran f
28		frn 5419	. . . . . . . . . . . . . . 15 |- ( f : om --> A -> ran f C_ A )
29	27, 28	sstrid 3195	. . . . . . . . . . . . . 14 |- ( f : om --> A -> ( f " n ) C_ A )
30	26, 29	syl 14	. . . . . . . . . . . . 13 |- ( f : om -onto-> A -> ( f " n ) C_ A )
31	30	ad2antrr 488	. . . . . . . . . . . 12 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> ( f " n ) C_ A )
32	31	3adantl1 1155	. . . . . . . . . . 11 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> ( f " n ) C_ A )
33		simpl2 1003	. . . . . . . . . . . . 13 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> f : om -onto-> A )
34		equequ1 1726	. . . . . . . . . . . . . . . 16 |- ( x = a -> ( x = y <-> a = y ) )
35	34	dcbid 839	. . . . . . . . . . . . . . 15 |- ( x = a -> (DECID x = y <-> DECID a = y ) )
36		equequ2 1727	. . . . . . . . . . . . . . . 16 |- ( y = b -> ( a = y <-> a = b ) )
37	36	dcbid 839	. . . . . . . . . . . . . . 15 |- ( y = b -> (DECID a = y <-> DECID a = b ) )
38	35, 37	cbvral2v 2742	. . . . . . . . . . . . . 14 |- ( A. x e. A A. y e. A DECID x = y <-> A. a e. A A. b e. A DECID a = b )
39		ssralv 3248	. . . . . . . . . . . . . . . . 17 |- ( ( f " n ) C_ A -> ( A. b e. A DECID a = b -> A. b e. ( f " n )DECID a = b ) )
40	30, 39	syl 14	. . . . . . . . . . . . . . . 16 |- ( f : om -onto-> A -> ( A. b e. A DECID a = b -> A. b e. ( f " n )DECID a = b ) )
41	40	ralimdv 2565	. . . . . . . . . . . . . . 15 |- ( f : om -onto-> A -> ( A. a e. A A. b e. A DECID a = b -> A. a e. A A. b e. ( f " n )DECID a = b ) )
42		ssralv 3248	. . . . . . . . . . . . . . 15 |- ( ( f " n ) C_ A -> ( A. a e. A A. b e. ( f " n )DECID a = b -> A. a e. ( f " n ) A. b e. ( f " n )DECID a = b ) )
43	30, 41, 42	sylsyld 58	. . . . . . . . . . . . . 14 |- ( f : om -onto-> A -> ( A. a e. A A. b e. A DECID a = b -> A. a e. ( f " n ) A. b e. ( f " n )DECID a = b ) )
44	38, 43	biimtrid 152	. . . . . . . . . . . . 13 |- ( f : om -onto-> A -> ( A. x e. A A. y e. A DECID x = y -> A. a e. ( f " n ) A. b e. ( f " n )DECID a = b ) )
45	33, 19, 44	sylc 62	. . . . . . . . . . . 12 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> A. a e. ( f " n ) A. b e. ( f " n )DECID a = b )
46		simpr 110	. . . . . . . . . . . . . 14 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> n e. om )
47		fofun 5484	. . . . . . . . . . . . . . . . 17 |- ( f : om -onto-> A -> Fun f )
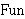48	47	ad2antrr 488	. . . . . . . . . . . . . . . 16 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> Fun f )
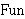
49		ordom 4644	. . . . . . . . . . . . . . . . . . 19 |- Ord om
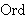50		ordtr 4414	. . . . . . . . . . . . . . . . . . 19 |- ( Ord om -> Tr om )
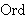
51	49, 50	ax-mp 5	. . . . . . . . . . . . . . . . . 18 |- Tr om
52		trss 4141	. . . . . . . . . . . . . . . . . 18 |- ( Tr om -> ( n e. om -> n C_ om ) )
53	51, 46, 52	mpsyl 65	. . . . . . . . . . . . . . . . 17 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> n C_ om )
54	26	fdmd 5417	. . . . . . . . . . . . . . . . . 18 |- ( f : om -onto-> A -> dom f = om )
55	54	ad2antrr 488	. . . . . . . . . . . . . . . . 17 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> dom f = om )
56	53, 55	sseqtrrd 3223	. . . . . . . . . . . . . . . 16 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> n C_ dom f )
57		fores 5493	. . . . . . . . . . . . . . . 16 |- ( ( Fun f /\ n C_ dom f ) -> ( f |` n ) : n -onto-> ( f " n ) )
58	48, 56, 57	syl2anc 411	. . . . . . . . . . . . . . 15 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> ( f |` n ) : n -onto-> ( f " n ) )
59		vex 2766	. . . . . . . . . . . . . . . . 17 |- f e. _V
60	59	resex 4988	. . . . . . . . . . . . . . . 16 |- ( f |` n ) e. _V
61		foeq1 5479	. . . . . . . . . . . . . . . 16 |- ( g = ( f |` n ) -> ( g : n -onto-> ( f " n ) <-> ( f |` n ) : n -onto-> ( f " n ) ) )
62	60, 61	spcev 2859	. . . . . . . . . . . . . . 15 |- ( ( f |` n ) : n -onto-> ( f " n ) -> E. g g : n -onto-> ( f " n ) )
63	58, 62	syl 14	. . . . . . . . . . . . . 14 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> E. g g : n -onto-> ( f " n ) )
64		foeq2 5480	. . . . . . . . . . . . . . . 16 |- ( m = n -> ( g : m -onto-> ( f " n ) <-> g : n -onto-> ( f " n ) ) )
65	64	exbidv 1839	. . . . . . . . . . . . . . 15 |- ( m = n -> ( E. g g : m -onto-> ( f " n ) <-> E. g g : n -onto-> ( f " n ) ) )
66	65	rspcev 2868	. . . . . . . . . . . . . 14 |- ( ( n e. om /\ E. g g : n -onto-> ( f " n ) ) -> E. m e. om E. g g : m -onto-> ( f " n ) )
67	46, 63, 66	syl2anc 411	. . . . . . . . . . . . 13 |- ( ( ( f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> E. m e. om E. g g : m -onto-> ( f " n ) )
68	67	3adantl1 1155	. . . . . . . . . . . 12 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> E. m e. om E. g g : m -onto-> ( f " n ) )
69		fidcenum 7031	. . . . . . . . . . . 12 |- ( ( f " n ) e. Fin <-> ( A. a e. ( f " n ) A. b e. ( f " n )DECID a = b /\ E. m e. om E. g g : m -onto-> ( f " n ) ) )
70	45, 68, 69	sylanbrc 417	. . . . . . . . . . 11 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> ( f " n ) e. Fin )
71	24, 25, 32, 70	inffinp1 12671	. . . . . . . . . 10 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> E. u e. A -. u e. ( f " n ) )
72		simprl 529	. . . . . . . . . . . 12 |- ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) -> u e. A )
73		foelrn 5802	. . . . . . . . . . . 12 |- ( ( f : om -onto-> A /\ u e. A ) -> E. k e. om u = ( f ` k ) )
74	33, 72, 73	syl2an2r 595	. . . . . . . . . . 11 |- ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) -> E. k e. om u = ( f ` k ) )
75		simpr 110	. . . . . . . . . . . . . 14 |- ( ( ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) /\ k e. om ) /\ u = ( f ` k ) ) -> u = ( f ` k ) )
76		simprr 531	. . . . . . . . . . . . . . 15 |- ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) -> -. u e. ( f " n ) )
77	76	ad2antrr 488	. . . . . . . . . . . . . 14 |- ( ( ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) /\ k e. om ) /\ u = ( f ` k ) ) -> -. u e. ( f " n ) )
78	75, 77	eqneltrrd 2293	. . . . . . . . . . . . 13 |- ( ( ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) /\ k e. om ) /\ u = ( f ` k ) ) -> -. ( f ` k ) e. ( f " n ) )
79	78	ex 115	. . . . . . . . . . . 12 |- ( ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) /\ k e. om ) -> ( u = ( f ` k ) -> -. ( f ` k ) e. ( f " n ) ) )
80	79	reximdva 2599	. . . . . . . . . . 11 |- ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) -> ( E. k e. om u = ( f ` k ) -> E. k e. om -. ( f ` k ) e. ( f " n ) ) )
81	74, 80	mpd 13	. . . . . . . . . 10 |- ( ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) /\ ( u e. A /\ -. u e. ( f " n ) ) ) -> E. k e. om -. ( f ` k ) e. ( f " n ) )
82	71, 81	rexlimddv 2619	. . . . . . . . 9 |- ( ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) /\ n e. om ) -> E. k e. om -. ( f ` k ) e. ( f " n ) )
83	82	ralrimiva 2570	. . . . . . . 8 |- ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) -> A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) )
84	18, 83	jca 306	. . . . . . 7 |- ( ( A. x e. A A. y e. A DECID x = y /\ f : om -onto-> A /\ om ~<_ A ) -> ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) )
85	84	3com23 1211	. . . . . 6 |- ( ( A. x e. A A. y e. A DECID x = y /\ om ~<_ A /\ f : om -onto-> A ) -> ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) )
86	85	3expia 1207	. . . . 5 |- ( ( A. x e. A A. y e. A DECID x = y /\ om ~<_ A ) -> ( f : om -onto-> A -> ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) ) )
87	86	eximdv 1894	. . . 4 |- ( ( A. x e. A A. y e. A DECID x = y /\ om ~<_ A ) -> ( E. f f : om -onto-> A -> E. f ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) ) )
88	16, 17, 87	sylc 62	. . 3 |- ( ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) -> E. f ( f : om -onto-> A /\ A. n e. om E. k e. om -. ( f ` k ) e. ( f " n ) ) )
89	15, 88, 1	sylanbrc 417	. 2 |- ( ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) -> A ~~ NN )
90	14, 89	impbii 126	1 |- ( A ~~ NN <-> ( A. x e. A A. y e. A DECID x = y /\ E. f f : om -onto-> A /\ om ~<_ A ) )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105  DECID wdc 835   /\ w3a 980   = wceq 1364   E.wex 1506   e. wcel 2167   A.wral 2475   E.wrex 2476   C_ wss 3157   class class class wbr 4034   Tr wtr 4132   Ord word 4398   omcom 4627   dom cdm 4664   ran crn 4665   |` cres 4666   "cima 4667   Fun wfun 5253   -->wf 5255   -onto->wfo 5257   ` cfv 5259   ~~ cen 6806   ~<_ cdom 6807   Fincfn 6808   NNcn 9007

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1461  ax-7 1462  ax-gen 1463  ax-ie1 1507  ax-ie2 1508  ax-8 1518  ax-10 1519  ax-11 1520  ax-i12 1521  ax-bndl 1523  ax-4 1524  ax-17 1540  ax-i9 1544  ax-ial 1548  ax-i5r 1549  ax-13 2169  ax-14 2170  ax-ext 2178  ax-coll 4149  ax-sep 4152  ax-nul 4160  ax-pow 4208  ax-pr 4243  ax-un 4469  ax-setind 4574  ax-iinf 4625  ax-cnex 7987  ax-resscn 7988  ax-1cn 7989  ax-1re 7990  ax-icn 7991  ax-addcl 7992  ax-addrcl 7993  ax-mulcl 7994  ax-addcom 7996  ax-addass 7998  ax-distr 8000  ax-i2m1 8001  ax-0lt1 8002  ax-0id 8004  ax-rnegex 8005  ax-cnre 8007  ax-pre-ltirr 8008  ax-pre-ltwlin 8009  ax-pre-lttrn 8010  ax-pre-ltadd 8012

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1475  df-sb 1777  df-eu 2048  df-mo 2049  df-clab 2183  df-cleq 2189  df-clel 2192  df-nfc 2328  df-ne 2368  df-nel 2463  df-ral 2480  df-rex 2481  df-reu 2482  df-rab 2484  df-v 2765  df-sbc 2990  df-csb 3085  df-dif 3159  df-un 3161  df-in 3163  df-ss 3170  df-nul 3452  df-if 3563  df-pw 3608  df-sn 3629  df-pr 3630  df-op 3632  df-uni 3841  df-int 3876  df-iun 3919  df-br 4035  df-opab 4096  df-mpt 4097  df-tr 4133  df-id 4329  df-iord 4402  df-on 4404  df-ilim 4405  df-suc 4407  df-iom 4628  df-xp 4670  df-rel 4671  df-cnv 4672  df-co 4673  df-dm 4674  df-rn 4675  df-res 4676  df-ima 4677  df-iota 5220  df-fun 5261  df-fn 5262  df-f 5263  df-f1 5264  df-fo 5265  df-f1o 5266  df-fv 5267  df-riota 5880  df-ov 5928  df-oprab 5929  df-mpo 5930  df-1st 6207  df-2nd 6208  df-recs 6372  df-frec 6458  df-1o 6483  df-er 6601  df-pm 6719  df-en 6809  df-dom 6810  df-fin 6811  df-dju 7113  df-inl 7122  df-inr 7123  df-case 7159  df-pnf 8080  df-mnf 8081  df-xr 8082  df-ltxr 8083  df-le 8084  df-sub 8216  df-neg 8217  df-inn 9008  df-n0 9267  df-z 9344  df-uz 9619  df-fz 10101  df-seqfrec 10557

This theorem is referenced by:  qnnen  12673  unbendc  12696  nnnninfen  15752