Theorem fsumcl2lem 11544

Description: - Lemma for finite sum closures. (The "-" before "Lemma" forces the math content to be displayed in the Statement List - NM 11-Feb-2008.) (Contributed by Mario Carneiro, 3-Jun-2014.)

Hypotheses

Ref	Expression
fsumcllem.1	|- ( ph -> S C_ CC )
fsumcllem.2	|- ( ( ph /\ ( x e. S /\ y e. S ) ) -> ( x + y ) e. S )
fsumcllem.3	|- ( ph -> A e. Fin )
fsumcllem.4	|- ( ( ph /\ k e. A ) -> B e. S )
fsumcl2lem.5	|- ( ph -> A =/= (/) )

Assertion

Ref	Expression
fsumcl2lem	|- ( ph -> sum_ k e. A B e. S )

Distinct variable groups:   A, k, x, y   x, B, y   S, k, x, y   ph, k, x, y

Allowed substitution hint:   B( k)

Proof of Theorem fsumcl2lem

Dummy variables a f m are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		fsumcl2lem.5	. . . 4 |- ( ph -> A =/= (/) )
2	1	neneqd 2385	. . 3 |- ( ph -> -. A = (/) )
3	2	pm2.21d 620	. 2 |- ( ph -> ( A = (/) -> sum_ k e. A B e. S ) )
4		fsumcllem.1	. . . . . . . . . . . 12 |- ( ph -> S C_ CC )
5	4	adantr 276	. . . . . . . . . . 11 |- ( ( ph /\ k e. A ) -> S C_ CC )
6		fsumcllem.4	. . . . . . . . . . 11 |- ( ( ph /\ k e. A ) -> B e. S )
7	5, 6	sseldd 3181	. . . . . . . . . 10 |- ( ( ph /\ k e. A ) -> B e. CC )
8	7	ralrimiva 2567	. . . . . . . . 9 |- ( ph -> A. k e. A B e. CC )
9		sumfct 11520	. . . . . . . . 9 |- ( A. k e. A B e. CC -> sum_ m e. A ( ( k e. A |-> B ) ` m ) = sum_ k e. A B )
10	8, 9	syl 14	. . . . . . . 8 |- ( ph -> sum_ m e. A ( ( k e. A |-> B ) ` m ) = sum_ k e. A B )
11	10	adantr 276	. . . . . . 7 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> sum_ m e. A ( ( k e. A |-> B ) ` m ) = sum_ k e. A B )
12		fveq2 5555	. . . . . . . 8 |- ( m = ( f ` a ) -> ( ( k e. A |-> B ) ` m ) = ( ( k e. A |-> B ) ` ( f ` a ) ) )
13		simprl 529	. . . . . . . 8 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> (♯ ` A ) e. NN )
14		simprr 531	. . . . . . . 8 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A )
15	4	ad2antrr 488	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ m e. A ) -> S C_ CC )
16	6	fmpttd 5714	. . . . . . . . . . 11 |- ( ph -> ( k e. A |-> B ) : A --> S )
17	16	adantr 276	. . . . . . . . . 10 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> ( k e. A |-> B ) : A --> S )
18	17	ffvelcdmda 5694	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ m e. A ) -> ( ( k e. A |-> B ) ` m ) e. S )
19	15, 18	sseldd 3181	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ m e. A ) -> ( ( k e. A |-> B ) ` m ) e. CC )
20		f1of 5501	. . . . . . . . . 10 |- ( f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A -> f : ( 1 ... (♯ ` A ) ) --> A )
21	14, 20	syl 14	. . . . . . . . 9 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> f : ( 1 ... (♯ ` A ) ) --> A )
22		fvco3 5629	. . . . . . . . 9 |- ( ( f : ( 1 ... (♯ ` A ) ) --> A /\ a e. ( 1 ... (♯ ` A ) ) ) -> ( ( ( k e. A |-> B ) o. f ) ` a ) = ( ( k e. A |-> B ) ` ( f ` a ) ) )
23	21, 22	sylan 283	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ a e. ( 1 ... (♯ ` A ) ) ) -> ( ( ( k e. A |-> B ) o. f ) ` a ) = ( ( k e. A |-> B ) ` ( f ` a ) ) )
24	12, 13, 14, 19, 23	fsum3 11533	. . . . . . 7 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> sum_ m e. A ( ( k e. A |-> B ) ` m ) = ( seq 1 ( + , ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ) ` (♯ ` A ) ) )
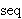25	11, 24	eqtr3d 2228	. . . . . 6 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> sum_ k e. A B = ( seq 1 ( + , ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ) ` (♯ ` A ) ) )
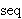26		nnuz 9631	. . . . . . . 8 |- NN = ( ZZ>= ` 1 )
27	13, 26	eleqtrdi 2286	. . . . . . 7 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> (♯ ` A ) e. ( ZZ>= ` 1 ) )
28		elnnuz 9632	. . . . . . . . . . 11 |- ( x e. NN <-> x e. ( ZZ>= ` 1 ) )
29	28	biimpri 133	. . . . . . . . . 10 |- ( x e. ( ZZ>= ` 1 ) -> x e. NN )
30	29	adantl 277	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> x e. NN )
31	4	ad3antrrr 492	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> S C_ CC )
32	17	ad2antrr 488	. . . . . . . . . . . . . 14 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( k e. A |-> B ) : A --> S )
33	21	ad2antrr 488	. . . . . . . . . . . . . 14 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> f : ( 1 ... (♯ ` A ) ) --> A )
34		fco 5420	. . . . . . . . . . . . . 14 |- ( ( ( k e. A |-> B ) : A --> S /\ f : ( 1 ... (♯ ` A ) ) --> A ) -> ( ( k e. A |-> B ) o. f ) : ( 1 ... (♯ ` A ) ) --> S )
35	32, 33, 34	syl2anc 411	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( ( k e. A |-> B ) o. f ) : ( 1 ... (♯ ` A ) ) --> S )
36		1zzd 9347	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> 1 e. ZZ )
37	13	ad2antrr 488	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> (♯ ` A ) e. NN )
38	37	nnzd 9441	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> (♯ ` A ) e. ZZ )
39		simpr 110	. . . . . . . . . . . . . . . . 17 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> x e. NN )
40	39	adantr 276	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> x e. NN )
41	40	nnzd 9441	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> x e. ZZ )
42	36, 38, 41	3jca 1179	. . . . . . . . . . . . . 14 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( 1 e. ZZ /\ (♯ ` A ) e. ZZ /\ x e. ZZ ) )
43	40	nnge1d 9027	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> 1 <_ x )
44		simpr 110	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> x <_ (♯ ` A ) )
45	43, 44	jca 306	. . . . . . . . . . . . . 14 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( 1 <_ x /\ x <_ (♯ ` A ) ) )
46		elfz2 10084	. . . . . . . . . . . . . 14 |- ( x e. ( 1 ... (♯ ` A ) ) <-> ( ( 1 e. ZZ /\ (♯ ` A ) e. ZZ /\ x e. ZZ ) /\ ( 1 <_ x /\ x <_ (♯ ` A ) ) ) )
47	42, 45, 46	sylanbrc 417	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> x e. ( 1 ... (♯ ` A ) ) )
48	35, 47	ffvelcdmd 5695	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( ( ( k e. A |-> B ) o. f ) ` x ) e. S )
49	31, 48	sseldd 3181	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ x <_ (♯ ` A ) ) -> ( ( ( k e. A |-> B ) o. f ) ` x ) e. CC )
50		0cnd 8014	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) /\ -. x <_ (♯ ` A ) ) -> 0 e. CC )
51	39	nnzd 9441	. . . . . . . . . . . 12 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> x e. ZZ )
52	13	adantr 276	. . . . . . . . . . . . 13 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> (♯ ` A ) e. NN )
53	52	nnzd 9441	. . . . . . . . . . . 12 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> (♯ ` A ) e. ZZ )
54		zdcle 9396	. . . . . . . . . . . 12 |- ( ( x e. ZZ /\ (♯ ` A ) e. ZZ ) -> DECID x <_ (♯ ` A ) )
55	51, 53, 54	syl2anc 411	. . . . . . . . . . 11 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> DECID x <_ (♯ ` A ) )
56	49, 50, 55	ifcldadc 3587	. . . . . . . . . 10 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. CC )
57	30, 56	syldan 282	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. CC )
58		breq1 4033	. . . . . . . . . . 11 |- ( a = x -> ( a <_ (♯ ` A ) <-> x <_ (♯ ` A ) ) )
59		fveq2 5555	. . . . . . . . . . 11 |- ( a = x -> ( ( ( k e. A |-> B ) o. f ) ` a ) = ( ( ( k e. A |-> B ) o. f ) ` x ) )
60	58, 59	ifbieq1d 3580	. . . . . . . . . 10 |- ( a = x -> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) = if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) )
61		eqid 2193	. . . . . . . . . 10 |- ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) = ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) )
62	60, 61	fvmptg 5634	. . . . . . . . 9 |- ( ( x e. NN /\ if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. CC ) -> ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) = if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) )
63	30, 57, 62	syl2anc 411	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) = if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) )
64	4	adantr 276	. . . . . . . . . . . . 13 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> S C_ CC )
65	17, 64	fssd 5417	. . . . . . . . . . . 12 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> ( k e. A |-> B ) : A --> CC )
66	65	ad2antrr 488	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> ( k e. A |-> B ) : A --> CC )
67	21	ad2antrr 488	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> f : ( 1 ... (♯ ` A ) ) --> A )
68		fco 5420	. . . . . . . . . . 11 |- ( ( ( k e. A |-> B ) : A --> CC /\ f : ( 1 ... (♯ ` A ) ) --> A ) -> ( ( k e. A |-> B ) o. f ) : ( 1 ... (♯ ` A ) ) --> CC )
69	66, 67, 68	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> ( ( k e. A |-> B ) o. f ) : ( 1 ... (♯ ` A ) ) --> CC )
70		1zzd 9347	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> 1 e. ZZ )
71	13	ad2antrr 488	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> (♯ ` A ) e. NN )
72	71	nnzd 9441	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> (♯ ` A ) e. ZZ )
73		eluzelz 9604	. . . . . . . . . . . . 13 |- ( x e. ( ZZ>= ` 1 ) -> x e. ZZ )
74	73	ad2antlr 489	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> x e. ZZ )
75	70, 72, 74	3jca 1179	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> ( 1 e. ZZ /\ (♯ ` A ) e. ZZ /\ x e. ZZ ) )
76	29	nnge1d 9027	. . . . . . . . . . . . 13 |- ( x e. ( ZZ>= ` 1 ) -> 1 <_ x )
77	76	ad2antlr 489	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> 1 <_ x )
78		simpr 110	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> x <_ (♯ ` A ) )
79	77, 78	jca 306	. . . . . . . . . . 11 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> ( 1 <_ x /\ x <_ (♯ ` A ) ) )
80	75, 79, 46	sylanbrc 417	. . . . . . . . . 10 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> x e. ( 1 ... (♯ ` A ) ) )
81	69, 80	ffvelcdmd 5695	. . . . . . . . 9 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ x <_ (♯ ` A ) ) -> ( ( ( k e. A |-> B ) o. f ) ` x ) e. CC )
82		0cnd 8014	. . . . . . . . 9 |- ( ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) /\ -. x <_ (♯ ` A ) ) -> 0 e. CC )
83	30, 55	syldan 282	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> DECID x <_ (♯ ` A ) )
84	81, 82, 83	ifcldadc 3587	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. CC )
85	63, 84	eqeltrd 2270	. . . . . . 7 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( ZZ>= ` 1 ) ) -> ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) e. CC )
86		elfzle2 10097	. . . . . . . . . . 11 |- ( x e. ( 1 ... (♯ ` A ) ) -> x <_ (♯ ` A ) )
87	86	adantl 277	. . . . . . . . . 10 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> x <_ (♯ ` A ) )
88	87	iftrued 3565	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) = ( ( ( k e. A |-> B ) o. f ) ` x ) )
89		elfznn 10123	. . . . . . . . . . 11 |- ( x e. ( 1 ... (♯ ` A ) ) -> x e. NN )
90	89	anim2i 342	. . . . . . . . . 10 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) )
91	90, 87, 48	syl2anc 411	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> ( ( ( k e. A |-> B ) o. f ) ` x ) e. S )
92	88, 91	eqeltrd 2270	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. S )
93	39, 56, 62	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) = if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) )
94	93	eleq1d 2262	. . . . . . . . 9 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. NN ) -> ( ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) e. S <-> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. S ) )
95	90, 94	syl 14	. . . . . . . 8 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> ( ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) e. S <-> if ( x <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` x ) , 0 ) e. S ) )
96	92, 95	mpbird 167	. . . . . . 7 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ x e. ( 1 ... (♯ ` A ) ) ) -> ( ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ` x ) e. S )
97		fsumcllem.2	. . . . . . . 8 |- ( ( ph /\ ( x e. S /\ y e. S ) ) -> ( x + y ) e. S )
98	97	adantlr 477	. . . . . . 7 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ ( x e. S /\ y e. S ) ) -> ( x + y ) e. S )
99		addcl 7999	. . . . . . . 8 |- ( ( x e. CC /\ y e. CC ) -> ( x + y ) e. CC )
100	99	adantl 277	. . . . . . 7 |- ( ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) /\ ( x e. CC /\ y e. CC ) ) -> ( x + y ) e. CC )
101	27, 85, 96, 98, 64, 100	seq3clss 10545	. . . . . 6 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> ( seq 1 ( + , ( a e. NN |-> if ( a <_ (♯ ` A ) , ( ( ( k e. A |-> B ) o. f ) ` a ) , 0 ) ) ) ` (♯ ` A ) ) e. S )
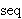102	25, 101	eqeltrd 2270	. . . . 5 |- ( ( ph /\ ( (♯ ` A ) e. NN /\ f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) -> sum_ k e. A B e. S )
103	102	expr 375	. . . 4 |- ( ( ph /\ (♯ ` A ) e. NN ) -> ( f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A -> sum_ k e. A B e. S ) )
104	103	exlimdv 1830	. . 3 |- ( ( ph /\ (♯ ` A ) e. NN ) -> ( E. f f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A -> sum_ k e. A B e. S ) )
105	104	expimpd 363	. 2 |- ( ph -> ( ( (♯ ` A ) e. NN /\ E. f f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) -> sum_ k e. A B e. S ) )
106		fsumcllem.3	. . 3 |- ( ph -> A e. Fin )
107		fz1f1o 11521	. . 3 |- ( A e. Fin -> ( A = (/) \/ ( (♯ ` A ) e. NN /\ E. f f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) )
108	106, 107	syl 14	. 2 |- ( ph -> ( A = (/) \/ ( (♯ ` A ) e. NN /\ E. f f : ( 1 ... (♯ ` A ) ) -1-1-onto-> A ) ) )
109	3, 105, 108	mpjaod 719	1 |- ( ph -> sum_ k e. A B e. S )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105   \/ wo 709  DECID wdc 835   /\ w3a 980   = wceq 1364   E.wex 1503   e. wcel 2164   =/= wne 2364   A.wral 2472   C_ wss 3154   (/)c0 3447   ifcif 3558   class class class wbr 4030   |-> cmpt 4091   o. ccom 4664   -->wf 5251   -1-1-onto->wf1o 5254   ` cfv 5255  (class class class)co 5919   Fincfn 6796   CCcc 7872   0cc0 7874   1c1 7875   + caddc 7877   <_ cle 8057   NNcn 8984   ZZcz 9320   ZZ>=cuz 9595   ...cfz 10077   seqcseq 10521  ♯chash 10849   sum_csu 11499

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-coll 4145  ax-sep 4148  ax-nul 4156  ax-pow 4204  ax-pr 4239  ax-un 4465  ax-setind 4570  ax-iinf 4621  ax-cnex 7965  ax-resscn 7966  ax-1cn 7967  ax-1re 7968  ax-icn 7969  ax-addcl 7970  ax-addrcl 7971  ax-mulcl 7972  ax-mulrcl 7973  ax-addcom 7974  ax-mulcom 7975  ax-addass 7976  ax-mulass 7977  ax-distr 7978  ax-i2m1 7979  ax-0lt1 7980  ax-1rid 7981  ax-0id 7982  ax-rnegex 7983  ax-precex 7984  ax-cnre 7985  ax-pre-ltirr 7986  ax-pre-ltwlin 7987  ax-pre-lttrn 7988  ax-pre-apti 7989  ax-pre-ltadd 7990  ax-pre-mulgt0 7991  ax-pre-mulext 7992  ax-arch 7993  ax-caucvg 7994

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-nel 2460  df-ral 2477  df-rex 2478  df-reu 2479  df-rmo 2480  df-rab 2481  df-v 2762  df-sbc 2987  df-csb 3082  df-dif 3156  df-un 3158  df-in 3160  df-ss 3167  df-nul 3448  df-if 3559  df-pw 3604  df-sn 3625  df-pr 3626  df-op 3628  df-uni 3837  df-int 3872  df-iun 3915  df-br 4031  df-opab 4092  df-mpt 4093  df-tr 4129  df-id 4325  df-po 4328  df-iso 4329  df-iord 4398  df-on 4400  df-ilim 4401  df-suc 4403  df-iom 4624  df-xp 4666  df-rel 4667  df-cnv 4668  df-co 4669  df-dm 4670  df-rn 4671  df-res 4672  df-ima 4673  df-iota 5216  df-fun 5257  df-fn 5258  df-f 5259  df-f1 5260  df-fo 5261  df-f1o 5262  df-fv 5263  df-isom 5264  df-riota 5874  df-ov 5922  df-oprab 5923  df-mpo 5924  df-1st 6195  df-2nd 6196  df-recs 6360  df-irdg 6425  df-frec 6446  df-1o 6471  df-oadd 6475  df-er 6589  df-en 6797  df-dom 6798  df-fin 6799  df-pnf 8058  df-mnf 8059  df-xr 8060  df-ltxr 8061  df-le 8062  df-sub 8194  df-neg 8195  df-reap 8596  df-ap 8603  df-div 8694  df-inn 8985  df-2 9043  df-3 9044  df-4 9045  df-n0 9244  df-z 9321  df-uz 9596  df-q 9688  df-rp 9723  df-fz 10078  df-fzo 10212  df-seqfrec 10522  df-exp 10613  df-ihash 10850  df-cj 10989  df-re 10990  df-im 10991  df-rsqrt 11145  df-abs 11146  df-clim 11425  df-sumdc 11500

This theorem is referenced by:  fsumcllem  11545  fsumrpcl  11550