Theorem nconstwlpolemgt0 15708

Description: Lemma for nconstwlpo 15710. If one of the terms of series is positive, so is the sum. (Contributed by Jim Kingdon, 26-Jul-2024.)

Hypotheses

Ref	Expression
nconstwlpolem0.g	|- ( ph -> G : NN --> { 0 , 1 } )
nconstwlpolem0.a	|- A = sum_ i e. NN ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) )
nconstwlpolemgt0.0	|- ( ph -> E. x e. NN ( G ` x ) = 1 )

Assertion

Ref	Expression
nconstwlpolemgt0	|- ( ph -> 0 < A )

Distinct variable groups:   x, A   i, G   ph, i, x

Allowed substitution hints:   A( i)   G( x)

Proof of Theorem nconstwlpolemgt0

Dummy variable n is distinct from all other variables.

Step	Hyp	Ref	Expression
1		nconstwlpolemgt0.0	. 2 |- ( ph -> E. x e. NN ( G ` x ) = 1 )
2		1zzd 9353	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 1 e. ZZ )
3		simprl 529	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> x e. NN )
4	3	peano2nnd 9005	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( x + 1 ) e. NN )
5	4	nnzd 9447	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( x + 1 ) e. ZZ )
6	5, 2	zsubcld 9453	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( x + 1 ) - 1 ) e. ZZ )
7	2, 6	fzfigd 10523	. . . . . 6 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 ... ( ( x + 1 ) - 1 ) ) e. Fin )
8		elfznn 10129	. . . . . . 7 |- ( i e. ( 1 ... ( ( x + 1 ) - 1 ) ) -> i e. NN )
9		2rp 9733	. . . . . . . . . . . 12 |- 2 e. RR+
10	9	a1i 9	. . . . . . . . . . 11 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> 2 e. RR+ )
11		simpr 110	. . . . . . . . . . . 12 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> i e. NN )
12	11	nnzd 9447	. . . . . . . . . . 11 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> i e. ZZ )
13	10, 12	rpexpcld 10789	. . . . . . . . . 10 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( 2 ^ i ) e. RR+ )
14	13	rpreccld 9782	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( 1 / ( 2 ^ i ) ) e. RR+ )
15	14	rpred 9771	. . . . . . . 8 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( 1 / ( 2 ^ i ) ) e. RR )
16		0re 8026	. . . . . . . . . 10 |- 0 e. RR
17		1re 8025	. . . . . . . . . 10 |- 1 e. RR
18		prssi 3780	. . . . . . . . . 10 |- ( ( 0 e. RR /\ 1 e. RR ) -> { 0 , 1 } C_ RR )
19	16, 17, 18	mp2an 426	. . . . . . . . 9 |- { 0 , 1 } C_ RR
20		nconstwlpolem0.g	. . . . . . . . . . 11 |- ( ph -> G : NN --> { 0 , 1 } )
21	20	ad2antrr 488	. . . . . . . . . 10 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> G : NN --> { 0 , 1 } )
22	21, 11	ffvelcdmd 5698	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( G ` i ) e. { 0 , 1 } )
23	19, 22	sselid 3181	. . . . . . . 8 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( G ` i ) e. RR )
24	15, 23	remulcld 8057	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
25	8, 24	sylan2 286	. . . . . 6 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
26	7, 25	fsumrecl 11566	. . . . 5 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
27		eqid 2196	. . . . . 6 |- ( ZZ>= ` ( x + 1 ) ) = ( ZZ>= ` ( x + 1 ) )
28		eqid 2196	. . . . . . 7 |- ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) = ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) )
29		oveq2 5930	. . . . . . . . 9 |- ( n = i -> ( 2 ^ n ) = ( 2 ^ i ) )
30	29	oveq2d 5938	. . . . . . . 8 |- ( n = i -> ( 1 / ( 2 ^ n ) ) = ( 1 / ( 2 ^ i ) ) )
31		fveq2 5558	. . . . . . . 8 |- ( n = i -> ( G ` n ) = ( G ` i ) )
32	30, 31	oveq12d 5940	. . . . . . 7 |- ( n = i -> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) = ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
33		eluznn 9674	. . . . . . . 8 |- ( ( ( x + 1 ) e. NN /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> i e. NN )
34	4, 33	sylan 283	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> i e. NN )
35	34, 24	syldan 282	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
36	28, 32, 34, 35	fvmptd3 5655	. . . . . 6 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> ( ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ` i ) = ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
37	20, 28	trilpolemclim 15680	. . . . . . . 8 |- ( ph -> seq 1 ( + , ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ) e. dom ~~> )
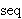38	37	adantr 276	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> seq 1 ( + , ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ) e. dom ~~> )
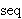39		nnuz 9637	. . . . . . . 8 |- NN = ( ZZ>= ` 1 )
40	28, 32, 11, 24	fvmptd3 5655	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ` i ) = ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
41	24	recnd 8055	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. CC )
42	40, 41	eqeltrd 2273	. . . . . . . 8 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ` i ) e. CC )
43	39, 4, 42	iserex 11504	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( seq 1 ( + , ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ) e. dom ~~> <-> seq ( x + 1 ) ( + , ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ) e. dom ~~> ) )
44	38, 43	mpbid 147	. . . . . 6 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> seq ( x + 1 ) ( + , ( n e. NN |-> ( ( 1 / ( 2 ^ n ) ) x. ( G ` n ) ) ) ) e. dom ~~> )
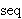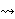45	27, 5, 36, 35, 44	isumrecl 11594	. . . . 5 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. ( ZZ>= ` ( x + 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
46	3	nnzd 9447	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> x e. ZZ )
47		fzofig 10524	. . . . . . . . . 10 |- ( ( 1 e. ZZ /\ x e. ZZ ) -> ( 1..^ x ) e. Fin )
48	2, 46, 47	syl2anc 411	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1..^ x ) e. Fin )
49		elfzo1 10266	. . . . . . . . . . 11 |- ( i e. ( 1..^ x ) <-> ( i e. NN /\ x e. NN /\ i < x ) )
50	49	simp1bi 1014	. . . . . . . . . 10 |- ( i e. ( 1..^ x ) -> i e. NN )
51	50, 24	sylan2 286	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( 1..^ x ) ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
52	48, 51	fsumrecl 11566	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. ( 1..^ x ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. RR )
53	9	a1i 9	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 2 e. RR+ )
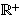54	53, 46	rpexpcld 10789	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 2 ^ x ) e. RR+ )
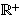55	54	rpreccld 9782	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 / ( 2 ^ x ) ) e. RR+ )
56	55	rpred 9771	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 / ( 2 ^ x ) ) e. RR )
57	20	adantr 276	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> G : NN --> { 0 , 1 } )
58	57, 3	ffvelcdmd 5698	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( G ` x ) e. { 0 , 1 } )
59	19, 58	sselid 3181	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( G ` x ) e. RR )
60	56, 59	remulcld 8057	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) e. RR )
61	14	rpge0d 9775	. . . . . . . . . . 11 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> 0 <_ ( 1 / ( 2 ^ i ) ) )
62		0le0 9079	. . . . . . . . . . . . 13 |- 0 <_ 0
63		simpr 110	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) /\ ( G ` i ) = 0 ) -> ( G ` i ) = 0 )
64	62, 63	breqtrrid 4071	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) /\ ( G ` i ) = 0 ) -> 0 <_ ( G ` i ) )
65		0le1 8508	. . . . . . . . . . . . 13 |- 0 <_ 1
66		simpr 110	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) /\ ( G ` i ) = 1 ) -> ( G ` i ) = 1 )
67	65, 66	breqtrrid 4071	. . . . . . . . . . . 12 |- ( ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) /\ ( G ` i ) = 1 ) -> 0 <_ ( G ` i ) )
68		elpri 3645	. . . . . . . . . . . . 13 |- ( ( G ` i ) e. { 0 , 1 } -> ( ( G ` i ) = 0 \/ ( G ` i ) = 1 ) )
69	22, 68	syl 14	. . . . . . . . . . . 12 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> ( ( G ` i ) = 0 \/ ( G ` i ) = 1 ) )
70	64, 67, 69	mpjaodan 799	. . . . . . . . . . 11 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> 0 <_ ( G ` i ) )
71	15, 23, 61, 70	mulge0d 8648	. . . . . . . . . 10 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. NN ) -> 0 <_ ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
72	50, 71	sylan2 286	. . . . . . . . 9 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( 1..^ x ) ) -> 0 <_ ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
73	48, 51, 72	fsumge0 11624	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 <_ sum_ i e. ( 1..^ x ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
74	55	rpgt0d 9774	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < ( 1 / ( 2 ^ x ) ) )
75		simprr 531	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( G ` x ) = 1 )
76	75	oveq2d 5938	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) = ( ( 1 / ( 2 ^ x ) ) x. 1 ) )
77	56	recnd 8055	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 / ( 2 ^ x ) ) e. CC )
78	77	mulridd 8043	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( 1 / ( 2 ^ x ) ) x. 1 ) = ( 1 / ( 2 ^ x ) ) )
79	76, 78	eqtrd 2229	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) = ( 1 / ( 2 ^ x ) ) )
80	74, 79	breqtrrd 4061	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) )
81	52, 60, 73, 80	addgegt0d 8546	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < ( sum_ i e. ( 1..^ x ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) + ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) ) )
82		nfv 1542	. . . . . . . 8 |- F/ i ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) )
83		nfcv 2339	. . . . . . . 8 |- F/_ i ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) )
84		fzonel 10236	. . . . . . . . 9 |- -. x e. ( 1..^ x )
85	84	a1i 9	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> -. x e. ( 1..^ x ) )
86	50, 41	sylan2 286	. . . . . . . 8 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( 1..^ x ) ) -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) e. CC )
87		oveq2 5930	. . . . . . . . . 10 |- ( i = x -> ( 2 ^ i ) = ( 2 ^ x ) )
88	87	oveq2d 5938	. . . . . . . . 9 |- ( i = x -> ( 1 / ( 2 ^ i ) ) = ( 1 / ( 2 ^ x ) ) )
89		fveq2 5558	. . . . . . . . 9 |- ( i = x -> ( G ` i ) = ( G ` x ) )
90	88, 89	oveq12d 5940	. . . . . . . 8 |- ( i = x -> ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) = ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) )
91	60	recnd 8055	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) e. CC )
92	82, 83, 48, 3, 85, 86, 90, 91	fsumsplitsn 11575	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. ( ( 1..^ x ) u. { x } ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) = ( sum_ i e. ( 1..^ x ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) + ( ( 1 / ( 2 ^ x ) ) x. ( G ` x ) ) ) )
93	81, 92	breqtrrd 4061	. . . . . 6 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < sum_ i e. ( ( 1..^ x ) u. { x } ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
94	3	nncnd 9004	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> x e. CC )
95		1cnd 8042	. . . . . . . . . 10 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 1 e. CC )
96	94, 95	pncand 8338	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( ( x + 1 ) - 1 ) = x )
97	96	oveq2d 5938	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 ... ( ( x + 1 ) - 1 ) ) = ( 1 ... x ) )
98	3, 39	eleqtrdi 2289	. . . . . . . . 9 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> x e. ( ZZ>= ` 1 ) )
99		fzisfzounsn 10312	. . . . . . . . 9 |- ( x e. ( ZZ>= ` 1 ) -> ( 1 ... x ) = ( ( 1..^ x ) u. { x } ) )
100	98, 99	syl 14	. . . . . . . 8 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 ... x ) = ( ( 1..^ x ) u. { x } ) )
101	97, 100	eqtrd 2229	. . . . . . 7 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> ( 1 ... ( ( x + 1 ) - 1 ) ) = ( ( 1..^ x ) u. { x } ) )
102	101	sumeq1d 11531	. . . . . 6 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) = sum_ i e. ( ( 1..^ x ) u. { x } ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
103	93, 102	breqtrrd 4061	. . . . 5 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < sum_ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
104	34, 15	syldan 282	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> ( 1 / ( 2 ^ i ) ) e. RR )
105	34, 23	syldan 282	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> ( G ` i ) e. RR )
106	34, 14	syldan 282	. . . . . . . 8 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> ( 1 / ( 2 ^ i ) ) e. RR+ )
107	106	rpge0d 9775	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> 0 <_ ( 1 / ( 2 ^ i ) ) )
108	34, 70	syldan 282	. . . . . . 7 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> 0 <_ ( G ` i ) )
109	104, 105, 107, 108	mulge0d 8648	. . . . . 6 |- ( ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) /\ i e. ( ZZ>= ` ( x + 1 ) ) ) -> 0 <_ ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
110	27, 5, 36, 35, 44, 109	isumge0 11595	. . . . 5 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 <_ sum_ i e. ( ZZ>= ` ( x + 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
111	26, 45, 103, 110	addgtge0d 8547	. . . 4 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < ( sum_ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) + sum_ i e. ( ZZ>= ` ( x + 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) ) )
112	39, 27, 4, 40, 41, 38	isumsplit 11656	. . . 4 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> sum_ i e. NN ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) = ( sum_ i e. ( 1 ... ( ( x + 1 ) - 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) + sum_ i e. ( ZZ>= ` ( x + 1 ) ) ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) ) )
113	111, 112	breqtrrd 4061	. . 3 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < sum_ i e. NN ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) ) )
114		nconstwlpolem0.a	. . 3 |- A = sum_ i e. NN ( ( 1 / ( 2 ^ i ) ) x. ( G ` i ) )
115	113, 114	breqtrrdi 4075	. 2 |- ( ( ph /\ ( x e. NN /\ ( G ` x ) = 1 ) ) -> 0 < A )
116	1, 115	rexlimddv 2619	1 |- ( ph -> 0 < A )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   \/ wo 709   = wceq 1364   e. wcel 2167   E.wrex 2476   u. cun 3155   C_ wss 3157   {csn 3622   {cpr 3623   class class class wbr 4033   |-> cmpt 4094   dom cdm 4663   -->wf 5254   ` cfv 5258  (class class class)co 5922   Fincfn 6799   CCcc 7877   RRcr 7878   0cc0 7879   1c1 7880   + caddc 7882   x. cmul 7884   < clt 8061   <_ cle 8062   - cmin 8197   / cdiv 8699   NNcn 8990   2c2 9041   ZZcz 9326   ZZ>=cuz 9601   RR+crp 9728   ...cfz 10083  ..^cfzo 10217   seqcseq 10539   ^cexp 10630   ~~> cli 11443   sum_csu 11518

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1461  ax-7 1462  ax-gen 1463  ax-ie1 1507  ax-ie2 1508  ax-8 1518  ax-10 1519  ax-11 1520  ax-i12 1521  ax-bndl 1523  ax-4 1524  ax-17 1540  ax-i9 1544  ax-ial 1548  ax-i5r 1549  ax-13 2169  ax-14 2170  ax-ext 2178  ax-coll 4148  ax-sep 4151  ax-nul 4159  ax-pow 4207  ax-pr 4242  ax-un 4468  ax-setind 4573  ax-iinf 4624  ax-cnex 7970  ax-resscn 7971  ax-1cn 7972  ax-1re 7973  ax-icn 7974  ax-addcl 7975  ax-addrcl 7976  ax-mulcl 7977  ax-mulrcl 7978  ax-addcom 7979  ax-mulcom 7980  ax-addass 7981  ax-mulass 7982  ax-distr 7983  ax-i2m1 7984  ax-0lt1 7985  ax-1rid 7986  ax-0id 7987  ax-rnegex 7988  ax-precex 7989  ax-cnre 7990  ax-pre-ltirr 7991  ax-pre-ltwlin 7992  ax-pre-lttrn 7993  ax-pre-apti 7994  ax-pre-ltadd 7995  ax-pre-mulgt0 7996  ax-pre-mulext 7997  ax-arch 7998  ax-caucvg 7999

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1475  df-sb 1777  df-eu 2048  df-mo 2049  df-clab 2183  df-cleq 2189  df-clel 2192  df-nfc 2328  df-ne 2368  df-nel 2463  df-ral 2480  df-rex 2481  df-reu 2482  df-rmo 2483  df-rab 2484  df-v 2765  df-sbc 2990  df-csb 3085  df-dif 3159  df-un 3161  df-in 3163  df-ss 3170  df-nul 3451  df-if 3562  df-pw 3607  df-sn 3628  df-pr 3629  df-op 3631  df-uni 3840  df-int 3875  df-iun 3918  df-br 4034  df-opab 4095  df-mpt 4096  df-tr 4132  df-id 4328  df-po 4331  df-iso 4332  df-iord 4401  df-on 4403  df-ilim 4404  df-suc 4406  df-iom 4627  df-xp 4669  df-rel 4670  df-cnv 4671  df-co 4672  df-dm 4673  df-rn 4674  df-res 4675  df-ima 4676  df-iota 5219  df-fun 5260  df-fn 5261  df-f 5262  df-f1 5263  df-fo 5264  df-f1o 5265  df-fv 5266  df-isom 5267  df-riota 5877  df-ov 5925  df-oprab 5926  df-mpo 5927  df-1st 6198  df-2nd 6199  df-recs 6363  df-irdg 6428  df-frec 6449  df-1o 6474  df-oadd 6478  df-er 6592  df-en 6800  df-dom 6801  df-fin 6802  df-pnf 8063  df-mnf 8064  df-xr 8065  df-ltxr 8066  df-le 8067  df-sub 8199  df-neg 8200  df-reap 8602  df-ap 8609  df-div 8700  df-inn 8991  df-2 9049  df-3 9050  df-4 9051  df-n0 9250  df-z 9327  df-uz 9602  df-q 9694  df-rp 9729  df-ico 9969  df-fz 10084  df-fzo 10218  df-seqfrec 10540  df-exp 10631  df-ihash 10868  df-cj 11007  df-re 11008  df-im 11009  df-rsqrt 11163  df-abs 11164  df-clim 11444  df-sumdc 11519

This theorem is referenced by:  nconstwlpolem  15709