ILE Home Intuitionistic Logic Explorer < Previous   Next >
Nearby theorems
Mirrors  >  Home  >  ILE Home  >  Th. List  >  prdsex Unicode version
Theorem prdsex 12883
Description: Existence of the structure product. (Contributed by Jim Kingdon, 18-Mar-2025.)
Assertion
Ref Expression
prdsex |- ( ( S e. V /\ R e. W ) -> ( S X_s R ) e. _V )
Proof of Theorem prdsex
Dummy variables a c d e f g h r s v x are mutually distinct and distinct from all other variables.
StepHypRef Expression
1 elex 2771 . . . 4 |- ( S e. V -> S e. _V )
21adantr 276 . . 3 |- ( ( S e. V /\ R e. W ) -> S e. _V )
3 elex 2771 . . . 4 |- ( R e. W -> R e. _V )
43adantl 277 . . 3 |- ( ( S e. V /\ R e. W ) -> R e. _V )
5 dmexg 4927 . . . . 5 |- ( R e. W -> dom R e. _V )
6 basfn 12679 . . . . . . 7 |- Base Fn _V
7 simpr 110 . . . . . . . 8 |- ( ( S e. V /\ R e. W ) -> R e. W )
8 vex 2763 . . . . . . . 8 |- x e. _V
9 fvexg 5574 . . . . . . . 8 |- ( ( R e. W /\ x e. _V ) -> ( R ` x ) e. _V )
107, 8, 9sylancl 413 . . . . . . 7 |- ( ( S e. V /\ R e. W ) -> ( R ` x ) e. _V )
11 funfvex 5572 . . . . . . . 8 |- ( ( Fun Base /\ ( R ` x ) e. dom Base ) -> ( Base ` ( R ` x ) ) e. _V )
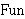
1211funfni 5355 . . . . . . 7 |- ( ( Base Fn _V /\ ( R ` x ) e. _V ) -> ( Base ` ( R ` x ) ) e. _V )
136, 10, 12sylancr 414 . . . . . 6 |- ( ( S e. V /\ R e. W ) -> ( Base ` ( R ` x ) ) e. _V )
1413ralrimivw 2568 . . . . 5 |- ( ( S e. V /\ R e. W ) -> A. x e. dom R ( Base ` ( R ` x ) ) e. _V )
15 ixpexgg 6778 . . . . 5 |- ( ( dom R e. _V /\ A. x e. dom R ( Base ` ( R ` x ) ) e. _V ) -> X_ x e. dom R ( Base ` ( R ` x ) ) e. _V )
165, 14, 15syl2an2 594 . . . 4 |- ( ( S e. V /\ R e. W ) -> X_ x e. dom R ( Base ` ( R ` x ) ) e. _V )
17 vex 2763 . . . . . . 7 |- v e. _V
1817, 17mpoex 6269 . . . . . 6 |- ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) e. _V
19 basendxnn 12677 . . . . . . . . . . 11 |- ( Base ` ndx ) e. NN
2017a1i 9 . . . . . . . . . . 11 |- ( ( S e. V /\ R e. W ) -> v e. _V )
21 opexg 4258 . . . . . . . . . . 11 |- ( ( ( Base ` ndx ) e. NN /\ v e. _V ) -> <. ( Base ` ndx ) , v >. e. _V )
2219, 20, 21sylancr 414 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( Base ` ndx ) , v >. e. _V )
23 plusgndxnn 12732 . . . . . . . . . . . . 13 |- ( +g ` ndx ) e. NN
2423elexi 2772 . . . . . . . . . . . 12 |- ( +g ` ndx ) e. _V
2517, 17mpoex 6269 . . . . . . . . . . . 12 |- ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) e. _V
2624, 25opex 4259 . . . . . . . . . . 11 |- <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V
2726a1i 9 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V )
28 mulrslid 12752 . . . . . . . . . . . . . 14 |- ( .r = Slot ( .r ` ndx ) /\ ( .r ` ndx ) e. NN )
2928simpri 113 . . . . . . . . . . . . 13 |- ( .r ` ndx ) e. NN
3029elexi 2772 . . . . . . . . . . . 12 |- ( .r ` ndx ) e. _V
3117, 17mpoex 6269 . . . . . . . . . . . 12 |- ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) e. _V
3230, 31opex 4259 . . . . . . . . . . 11 |- <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V
3332a1i 9 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V )
34 tpexg 4476 . . . . . . . . . 10 |- ( ( <. ( Base ` ndx ) , v >. e. _V /\ <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V /\ <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V ) -> { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } e. _V )
3522, 27, 33, 34syl3anc 1249 . . . . . . . . 9 |- ( ( S e. V /\ R e. W ) -> { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } e. _V )
36 scaslid 12773 . . . . . . . . . . . 12 |- (Scalar = Slot (Scalar ` ndx ) /\ (Scalar ` ndx ) e. NN )
3736simpri 113 . . . . . . . . . . 11 |- (Scalar ` ndx ) e. NN
38 simpl 109 . . . . . . . . . . 11 |- ( ( S e. V /\ R e. W ) -> S e. V )
39 opexg 4258 . . . . . . . . . . 11 |- ( ( (Scalar ` ndx ) e. NN /\ S e. V ) -> <. (Scalar ` ndx ) , S >. e. _V )
4037, 38, 39sylancr 414 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. (Scalar ` ndx ) , S >. e. _V )
41 vscaslid 12783 . . . . . . . . . . . 12 |- ( .s = Slot ( .s ` ndx ) /\ ( .s ` ndx ) e. NN )
4241simpri 113 . . . . . . . . . . 11 |- ( .s ` ndx ) e. NN
4338elexd 2773 . . . . . . . . . . . . 13 |- ( ( S e. V /\ R e. W ) -> S e. _V )
44 funfvex 5572 . . . . . . . . . . . . . 14 |- ( ( Fun Base /\ S e. dom Base ) -> ( Base ` S ) e. _V )
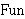
4544funfni 5355 . . . . . . . . . . . . 13 |- ( ( Base Fn _V /\ S e. _V ) -> ( Base ` S ) e. _V )
466, 43, 45sylancr 414 . . . . . . . . . . . 12 |- ( ( S e. V /\ R e. W ) -> ( Base ` S ) e. _V )
47 mpoexga 6267 . . . . . . . . . . . 12 |- ( ( ( Base ` S ) e. _V /\ v e. _V ) -> ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) e. _V )
4846, 17, 47sylancl 413 . . . . . . . . . . 11 |- ( ( S e. V /\ R e. W ) -> ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) e. _V )
49 opexg 4258 . . . . . . . . . . 11 |- ( ( ( .s ` ndx ) e. NN /\ ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) e. _V ) -> <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V )
5042, 48, 49sylancr 414 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V )
51 ipslid 12791 . . . . . . . . . . . . . 14 |- ( .i = Slot ( .i ` ndx ) /\ ( .i ` ndx ) e. NN )
5251simpri 113 . . . . . . . . . . . . 13 |- ( .i ` ndx ) e. NN
5352elexi 2772 . . . . . . . . . . . 12 |- ( .i ` ndx ) e. _V
5417, 17mpoex 6269 . . . . . . . . . . . 12 |- ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) e. _V
5553, 54opex 4259 . . . . . . . . . . 11 |- <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. e. _V
5655a1i 9 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. e. _V )
57 tpexg 4476 . . . . . . . . . 10 |- ( ( <. (Scalar ` ndx ) , S >. e. _V /\ <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. e. _V /\ <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. e. _V ) -> { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } e. _V )
5840, 50, 56, 57syl3anc 1249 . . . . . . . . 9 |- ( ( S e. V /\ R e. W ) -> { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } e. _V )
59 unexg 4475 . . . . . . . . 9 |- ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } e. _V /\ { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } e. _V ) -> ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) e. _V )
6035, 58, 59syl2anc 411 . . . . . . . 8 |- ( ( S e. V /\ R e. W ) -> ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) e. _V )
61 tsetndxnn 12809 . . . . . . . . . . 11 |- (TopSet ` ndx ) e. NN
62 topnfn 12858 . . . . . . . . . . . . . 14 |- TopOpen Fn _V
63 fnfun 5352 . . . . . . . . . . . . . 14 |- ( TopOpen Fn _V -> Fun TopOpen )
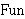
6462, 63ax-mp 5 . . . . . . . . . . . . 13 |- Fun TopOpen
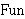65 cofunexg 6163 . . . . . . . . . . . . 13 |- ( ( Fun TopOpen /\ R e. W ) -> ( TopOpen o. R ) e. _V )
6664, 7, 65sylancr 414 . . . . . . . . . . . 12 |- ( ( S e. V /\ R e. W ) -> ( TopOpen o. R ) e. _V )
67 ptex 12878 . . . . . . . . . . . 12 |- ( ( TopOpen o. R ) e. _V -> ( Xt_ ` ( TopOpen o. R ) ) e. _V )
6866, 67syl 14 . . . . . . . . . . 11 |- ( ( S e. V /\ R e. W ) -> ( Xt_ ` ( TopOpen o. R ) ) e. _V )
69 opexg 4258 . . . . . . . . . . 11 |- ( ( (TopSet ` ndx ) e. NN /\ ( Xt_ ` ( TopOpen o. R ) ) e. _V ) -> <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. e. _V )
7061, 68, 69sylancr 414 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. e. _V )
71 plendxnn 12823 . . . . . . . . . . 11 |- ( le ` ndx ) e. NN
72 vex 2763 . . . . . . . . . . . . . . . 16 |- f e. _V
73 vex 2763 . . . . . . . . . . . . . . . 16 |- g e. _V
7472, 73prss 3775 . . . . . . . . . . . . . . 15 |- ( ( f e. v /\ g e. v ) <-> { f , g } C_ v )
7574anbi1i 458 . . . . . . . . . . . . . 14 |- ( ( ( f e. v /\ g e. v ) /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) <-> ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) )
7675opabbii 4097 . . . . . . . . . . . . 13 |- { <. f , g >. | ( ( f e. v /\ g e. v ) /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } = { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) }
7717, 17xpex 4775 . . . . . . . . . . . . . 14 |- ( v X. v ) e. _V
78 opabssxp 4734 . . . . . . . . . . . . . 14 |- { <. f , g >. | ( ( f e. v /\ g e. v ) /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } C_ ( v X. v )
7977, 78ssexi 4168 . . . . . . . . . . . . 13 |- { <. f , g >. | ( ( f e. v /\ g e. v ) /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } e. _V
8076, 79eqeltrri 2267 . . . . . . . . . . . 12 |- { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } e. _V
8180a1i 9 . . . . . . . . . . 11 |- ( ( S e. V /\ R e. W ) -> { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } e. _V )
82 opexg 4258 . . . . . . . . . . 11 |- ( ( ( le ` ndx ) e. NN /\ { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } e. _V ) -> <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. e. _V )
8371, 81, 82sylancr 414 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. e. _V )
84 dsndxnn 12834 . . . . . . . . . . . 12 |- ( dist ` ndx ) e. NN
8517, 17mpoex 6269 . . . . . . . . . . . 12 |- ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) e. _V
86 opexg 4258 . . . . . . . . . . . 12 |- ( ( ( dist ` ndx ) e. NN /\ ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) e. _V ) -> <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. e. _V )
8784, 85, 86mp2an 426 . . . . . . . . . . 11 |- <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. e. _V
8887a1i 9 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. e. _V )
89 tpexg 4476 . . . . . . . . . 10 |- ( ( <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. e. _V /\ <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. e. _V /\ <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. e. _V ) -> { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } e. _V )
9070, 83, 88, 89syl3anc 1249 . . . . . . . . 9 |- ( ( S e. V /\ R e. W ) -> { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } e. _V )
91 homslid 12850 . . . . . . . . . . . 12 |- ( Hom = Slot ( Hom ` ndx ) /\ ( Hom ` ndx ) e. NN )
9291simpri 113 . . . . . . . . . . 11 |- ( Hom ` ndx ) e. NN
93 vex 2763 . . . . . . . . . . 11 |- h e. _V
94 opexg 4258 . . . . . . . . . . 11 |- ( ( ( Hom ` ndx ) e. NN /\ h e. _V ) -> <. ( Hom ` ndx ) , h >. e. _V )
9592, 93, 94mp2an 426 . . . . . . . . . 10 |- <. ( Hom ` ndx ) , h >. e. _V
96 ccoslid 12852 . . . . . . . . . . . . 13 |- (comp = Slot (comp ` ndx ) /\ (comp ` ndx ) e. NN )
9796simpri 113 . . . . . . . . . . . 12 |- (comp ` ndx ) e. NN
9877, 17mpoex 6269 . . . . . . . . . . . 12 |- ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) e. _V
99 opexg 4258 . . . . . . . . . . . 12 |- ( ( (comp ` ndx ) e. NN /\ ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) e. _V ) -> <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. e. _V )
10097, 98, 99mp2an 426 . . . . . . . . . . 11 |- <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. e. _V
101100a1i 9 . . . . . . . . . 10 |- ( ( S e. V /\ R e. W ) -> <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. e. _V )
102 prexg 4241 . . . . . . . . . 10 |- ( ( <. ( Hom ` ndx ) , h >. e. _V /\ <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. e. _V ) -> { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } e. _V )
10395, 101, 102sylancr 414 . . . . . . . . 9 |- ( ( S e. V /\ R e. W ) -> { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } e. _V )
104 unexg 4475 . . . . . . . . 9 |- ( ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } e. _V /\ { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } e. _V ) -> ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) e. _V )
10590, 103, 104syl2anc 411 . . . . . . . 8 |- ( ( S e. V /\ R e. W ) -> ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) e. _V )
106 unexg 4475 . . . . . . . 8 |- ( ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) e. _V /\ ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) e. _V ) -> ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
10760, 105, 106syl2anc 411 . . . . . . 7 |- ( ( S e. V /\ R e. W ) -> ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
108107alrimiv 1885 . . . . . 6 |- ( ( S e. V /\ R e. W ) -> A. h ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
109 csbexga 4158 . . . . . 6 |- ( ( ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) e. _V /\ A. h ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V ) -> [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
11018, 108, 109sylancr 414 . . . . 5 |- ( ( S e. V /\ R e. W ) -> [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
111110alrimiv 1885 . . . 4 |- ( ( S e. V /\ R e. W ) -> A. v [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
112 csbexga 4158 . . . 4 |- ( ( X_ x e. dom R ( Base ` ( R ` x ) ) e. _V /\ A. v [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V ) -> [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
11316, 111, 112syl2anc 411 . . 3 |- ( ( S e. V /\ R e. W ) -> [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V )
114 dmeq 4863 . . . . . . . . 9 |- ( r = R -> dom r = dom R )
115114ixpeq1d 6766 . . . . . . . 8 |- ( r = R -> X_ x e. dom r ( Base ` ( r ` x ) ) = X_ x e. dom R ( Base ` ( r ` x ) ) )
116 fveq1 5554 . . . . . . . . . 10 |- ( r = R -> ( r ` x ) = ( R ` x ) )
117116fveq2d 5559 . . . . . . . . 9 |- ( r = R -> ( Base ` ( r ` x ) ) = ( Base ` ( R ` x ) ) )
118117ixpeq2dv 6770 . . . . . . . 8 |- ( r = R -> X_ x e. dom R ( Base ` ( r ` x ) ) = X_ x e. dom R ( Base ` ( R ` x ) ) )
119115, 118eqtrd 2226 . . . . . . 7 |- ( r = R -> X_ x e. dom r ( Base ` ( r ` x ) ) = X_ x e. dom R ( Base ` ( R ` x ) ) )
120119adantl 277 . . . . . 6 |- ( ( s = S /\ r = R ) -> X_ x e. dom r ( Base ` ( r ` x ) ) = X_ x e. dom R ( Base ` ( R ` x ) ) )
121120csbeq1d 3088 . . . . 5 |- ( ( s = S /\ r = R ) -> [_ X_ x e. dom r ( Base ` ( r ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
122114adantl 277 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> dom r = dom R )
123122ixpeq1d 6766 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) = X_ x e. dom R ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) )
124 simpr 110 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> r = R )
125124fveq1d 5557 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( r ` x ) = ( R ` x ) )
126125fveq2d 5559 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( Hom ` ( r ` x ) ) = ( Hom ` ( R ` x ) ) )
127126oveqd 5936 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) = ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) )
128127ixpeq2dv 6770 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> X_ x e. dom R ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) = X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) )
129123, 128eqtrd 2226 . . . . . . . . 9 |- ( ( s = S /\ r = R ) -> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) = X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) )
130129mpoeq3dv 5985 . . . . . . . 8 |- ( ( s = S /\ r = R ) -> ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) = ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) )
131130csbeq1d 3088 . . . . . . 7 |- ( ( s = S /\ r = R ) -> [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
132 eqidd 2194 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( Base ` ndx ) , v >. = <. ( Base ` ndx ) , v >. )
133125fveq2d 5559 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( +g ` ( r ` x ) ) = ( +g ` ( R ` x ) ) )
134133oveqd 5936 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) = ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) )
135122, 134mpteq12dv 4112 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) = ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) )
136135mpoeq3dv 5985 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) = ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) )
137136opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. = <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. )
138125fveq2d 5559 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( .r ` ( r ` x ) ) = ( .r ` ( R ` x ) ) )
139138oveqd 5936 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) = ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) )
140122, 139mpteq12dv 4112 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) = ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) )
141140mpoeq3dv 5985 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) = ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) )
142141opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. = <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. )
143132, 137, 142tpeq123d 3711 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } = { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } )
144 simpl 109 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> s = S )
145144opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. (Scalar ` ndx ) , s >. = <. (Scalar ` ndx ) , S >. )
146144fveq2d 5559 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( Base ` s ) = ( Base ` S ) )
147 eqidd 2194 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> v = v )
148125fveq2d 5559 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( .s ` ( r ` x ) ) = ( .s ` ( R ` x ) ) )
149148oveqd 5936 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) = ( f ( .s ` ( R ` x ) ) ( g ` x ) ) )
150122, 149mpteq12dv 4112 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) = ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) )
151146, 147, 150mpoeq123dv 5981 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) = ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) )
152151opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. = <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. )
153125fveq2d 5559 . . . . . . . . . . . . . . . 16 |- ( ( s = S /\ r = R ) -> ( .i ` ( r ` x ) ) = ( .i ` ( R ` x ) ) )
154153oveqd 5936 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) = ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) )
155122, 154mpteq12dv 4112 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) = ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) )
156144, 155oveq12d 5937 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) = ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) )
157156mpoeq3dv 5985 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) = ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) )
158157opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. = <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. )
159145, 152, 158tpeq123d 3711 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } = { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } )
160143, 159uneq12d 3315 . . . . . . . . 9 |- ( ( s = S /\ r = R ) -> ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) = ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) )
161124coeq2d 4825 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( TopOpen o. r ) = ( TopOpen o. R ) )
162161fveq2d 5559 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( Xt_ ` ( TopOpen o. r ) ) = ( Xt_ ` ( TopOpen o. R ) ) )
163162opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. = <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. )
164125fveq2d 5559 . . . . . . . . . . . . . . . 16 |- ( ( s = S /\ r = R ) -> ( le ` ( r ` x ) ) = ( le ` ( R ` x ) ) )
165164breqd 4041 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) <-> ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) )
166122, 165raleqbidv 2706 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) <-> A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) )
167166anbi2d 464 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) <-> ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) ) )
168167opabbidv 4096 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } = { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } )
169168opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. = <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. )
170125fveq2d 5559 . . . . . . . . . . . . . . . . . 18 |- ( ( s = S /\ r = R ) -> ( dist ` ( r ` x ) ) = ( dist ` ( R ` x ) ) )
171170oveqd 5936 . . . . . . . . . . . . . . . . 17 |- ( ( s = S /\ r = R ) -> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) = ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) )
172122, 171mpteq12dv 4112 . . . . . . . . . . . . . . . 16 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) = ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) )
173172rneqd 4892 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) = ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) )
174173uneq1d 3313 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) = ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) )
175174supeq1d 7048 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) = sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) )
176175mpoeq3dv 5985 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) = ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) )
177176opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. = <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. )
178163, 169, 177tpeq123d 3711 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } = { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } )
179125fveq2d 5559 . . . . . . . . . . . . . . . . 17 |- ( ( s = S /\ r = R ) -> (comp ` ( r ` x ) ) = (comp ` ( R ` x ) ) )
180179oveqd 5936 . . . . . . . . . . . . . . . 16 |- ( ( s = S /\ r = R ) -> ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) = ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) )
181180oveqd 5936 . . . . . . . . . . . . . . 15 |- ( ( s = S /\ r = R ) -> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) = ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) )
182122, 181mpteq12dv 4112 . . . . . . . . . . . . . 14 |- ( ( s = S /\ r = R ) -> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) = ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) )
183182mpoeq3dv 5985 . . . . . . . . . . . . 13 |- ( ( s = S /\ r = R ) -> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) = ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) )
184183mpoeq3dv 5985 . . . . . . . . . . . 12 |- ( ( s = S /\ r = R ) -> ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) = ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) )
185184opeq2d 3812 . . . . . . . . . . 11 |- ( ( s = S /\ r = R ) -> <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. = <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. )
186185preq2d 3703 . . . . . . . . . 10 |- ( ( s = S /\ r = R ) -> { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } = { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } )
187178, 186uneq12d 3315 . . . . . . . . 9 |- ( ( s = S /\ r = R ) -> ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) = ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) )
188160, 187uneq12d 3315 . . . . . . . 8 |- ( ( s = S /\ r = R ) -> ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
189188csbeq2dv 3107 . . . . . . 7 |- ( ( s = S /\ r = R ) -> [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
190131, 189eqtrd 2226 . . . . . 6 |- ( ( s = S /\ r = R ) -> [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
191190csbeq2dv 3107 . . . . 5 |- ( ( s = S /\ r = R ) -> [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
192121, 191eqtrd 2226 . . . 4 |- ( ( s = S /\ r = R ) -> [_ X_ x e. dom r ( Base ` ( r ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) = [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
193 df-prds 12881 . . . 4 |- X_s = ( s e. _V , r e. _V |-> [_ X_ x e. dom r ( Base ` ( r ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom r ( ( f ` x ) ( Hom ` ( r ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( +g ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom r |-> ( ( f ` x ) ( .r ` ( r ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , s >. , <. ( .s ` ndx ) , ( f e. ( Base ` s ) , g e. v |-> ( x e. dom r |-> ( f ( .s ` ( r ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( s gsumg ( x e. dom r |-> ( ( f ` x ) ( .i ` ( r ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. r ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom r ( f ` x ) ( le ` ( r ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom r |-> ( ( f ` x ) ( dist ` ( r ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom r |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( r ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
194192, 193ovmpoga 6049 . . 3 |- ( ( S e. _V /\ R e. _V /\ [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) e. _V ) -> ( S X_s R ) = [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
1952, 4, 113, 194syl3anc 1249 . 2 |- ( ( S e. V /\ R e. W ) -> ( S X_s R ) = [_ X_ x e. dom R ( Base ` ( R ` x ) ) / v ]_ [_ ( f e. v , g e. v |-> X_ x e. dom R ( ( f ` x ) ( Hom ` ( R ` x ) ) ( g ` x ) ) ) / h ]_ ( ( { <. ( Base ` ndx ) , v >. , <. ( +g ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( +g ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .r ` ndx ) , ( f e. v , g e. v |-> ( x e. dom R |-> ( ( f ` x ) ( .r ` ( R ` x ) ) ( g ` x ) ) ) ) >. } u. { <. (Scalar ` ndx ) , S >. , <. ( .s ` ndx ) , ( f e. ( Base ` S ) , g e. v |-> ( x e. dom R |-> ( f ( .s ` ( R ` x ) ) ( g ` x ) ) ) ) >. , <. ( .i ` ndx ) , ( f e. v , g e. v |-> ( S gsumg ( x e. dom R |-> ( ( f ` x ) ( .i ` ( R ` x ) ) ( g ` x ) ) ) ) ) >. } ) u. ( { <. (TopSet ` ndx ) , ( Xt_ ` ( TopOpen o. R ) ) >. , <. ( le ` ndx ) , { <. f , g >. | ( { f , g } C_ v /\ A. x e. dom R ( f ` x ) ( le ` ( R ` x ) ) ( g ` x ) ) } >. , <. ( dist ` ndx ) , ( f e. v , g e. v |-> sup ( ( ran ( x e. dom R |-> ( ( f ` x ) ( dist ` ( R ` x ) ) ( g ` x ) ) ) u. { 0 } ) , RR* , < ) ) >. } u. { <. ( Hom ` ndx ) , h >. , <. (comp ` ndx ) , ( a e. ( v X. v ) , c e. v |-> ( d e. ( c h ( 2nd ` a ) ) , e e. ( h ` a ) |-> ( x e. dom R |-> ( ( d ` x ) ( <. ( ( 1st ` a ) ` x ) , ( ( 2nd ` a ) ` x ) >. (comp ` ( R ` x ) ) ( c ` x ) ) ( e ` x ) ) ) ) ) >. } ) ) )
196195, 113eqeltrd 2270 1 |- ( ( S e. V /\ R e. W ) -> ( S X_s R ) e. _V )
Colors of variables: wff set class
Syntax hints:   -> wi 4   /\ wa 104   A.wal 1362   = wceq 1364   e. wcel 2164   A.wral 2472   _Vcvv 2760   [_csb 3081   u. cun 3152   C_ wss 3154   {csn 3619   {cpr 3620   {ctp 3621   <.cop 3622   class class class wbr 4030   {copab 4090   |-> cmpt 4091   X. cxp 4658   dom cdm 4660   ran crn 4661   o. ccom 4664   Fun wfun 5249   Fn wfn 5250   ` cfv 5255  (class class class)co 5919   e. cmpo 5921   1stc1st 6193   2ndc2nd 6194   X_cixp 6754   supcsup 7043   0cc0 7874   RR*cxr 8055   < clt 8056   NNcn 8984   ndxcnx 12618  Slot cslot 12620   Basecbs 12621   +g cplusg 12698   .rcmulr 12699  Scalarcsca 12701   .scvsca 12702   .icip 12703  TopSetcts 12704   lecple 12705   distcds 12707   Hom chom 12709  compcco 12710   TopOpenctopn 12854   Xt_cpt 12869   gsumg cgsu 12871   X_scprds 12879
This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1458  ax-7 1459  ax-gen 1460  ax-ie1 1504  ax-ie2 1505  ax-8 1515  ax-10 1516  ax-11 1517  ax-i12 1518  ax-bndl 1520  ax-4 1521  ax-17 1537  ax-i9 1541  ax-ial 1545  ax-i5r 1546  ax-13 2166  ax-14 2167  ax-ext 2175  ax-coll 4145  ax-sep 4148  ax-pow 4204  ax-pr 4239  ax-un 4465  ax-setind 4570  ax-cnex 7965  ax-resscn 7966  ax-1cn 7967  ax-1re 7968  ax-icn 7969  ax-addcl 7970  ax-addrcl 7971  ax-mulcl 7972  ax-addcom 7974  ax-mulcom 7975  ax-addass 7976  ax-mulass 7977  ax-distr 7978  ax-i2m1 7979  ax-1rid 7981  ax-0id 7982  ax-rnegex 7983  ax-cnre 7985
This theorem depends on definitions:  df-bi 117  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1472  df-sb 1774  df-eu 2045  df-mo 2046  df-clab 2180  df-cleq 2186  df-clel 2189  df-nfc 2325  df-ne 2365  df-ral 2477  df-rex 2478  df-reu 2479  df-rab 2481  df-v 2762  df-sbc 2987  df-csb 3082  df-dif 3156  df-un 3158  df-in 3160  df-ss 3167  df-pw 3604  df-sn 3625  df-pr 3626  df-tp 3627  df-op 3628  df-uni 3837  df-int 3872  df-iun 3915  df-br 4031  df-opab 4092  df-mpt 4093  df-id 4325  df-xp 4666  df-rel 4667  df-cnv 4668  df-co 4669  df-dm 4670  df-rn 4671  df-res 4672  df-ima 4673  df-iota 5216  df-fun 5257  df-fn 5258  df-f 5259  df-f1 5260  df-fo 5261  df-f1o 5262  df-fv 5263  df-riota 5874  df-ov 5922  df-oprab 5923  df-mpo 5924  df-1st 6195  df-2nd 6196  df-map 6706  df-ixp 6755  df-sup 7045  df-sub 8194  df-inn 8985  df-2 9043  df-3 9044  df-4 9045  df-5 9046  df-6 9047  df-7 9048  df-8 9049  df-9 9050  df-n0 9244  df-dec 9452  df-ndx 12624  df-slot 12625  df-base 12627  df-plusg 12711  df-mulr 12712  df-sca 12714  df-vsca 12715  df-ip 12716  df-tset 12717  df-ple 12718  df-ds 12720  df-hom 12722  df-cco 12723  df-rest 12855  df-topn 12856  df-topgen 12874  df-pt 12875  df-prds 12881
This theorem is referenced by:  xpsval  12938
  Copyright terms: Public domain W3C validator