Theorem ltsonq 7482

Description: 'Less than' is a strict ordering on positive fractions. (Contributed by NM, 19-Feb-1996.) (Revised by Mario Carneiro, 4-May-2013.)

Assertion

Ref	Expression
ltsonq	|- <Q Or Q.

Proof of Theorem ltsonq

Dummy variables a b c d e f x y z w are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		df-nqqs 7432	. . . . . 6 |- Q. = ( ( N. X. N. ) /. ~Q )
2		id 19	. . . . . . . 8 |- ( [ <. z , w >. ] ~Q = x -> [ <. z , w >. ] ~Q = x )
3	2, 2	breq12d 4047	. . . . . . 7 |- ( [ <. z , w >. ] ~Q = x -> ( [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q <-> x <Q x ) )
4	3	notbid 668	. . . . . 6 |- ( [ <. z , w >. ] ~Q = x -> ( -. [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q <-> -. x <Q x ) )
5		ltsopi 7404	. . . . . . . 8 |- <N Or N.
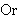6		ltrelpi 7408	. . . . . . . 8 |- <N C_ ( N. X. N. )
7	5, 6	soirri 5065	. . . . . . 7 |- -. ( w .N z ) <N ( w .N z )
8		ordpipqqs 7458	. . . . . . . . 9 |- ( ( ( z e. N. /\ w e. N. ) /\ ( z e. N. /\ w e. N. ) ) -> ( [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q <-> ( z .N w ) <N ( w .N z ) ) )
9	8	anidms 397	. . . . . . . 8 |- ( ( z e. N. /\ w e. N. ) -> ( [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q <-> ( z .N w ) <N ( w .N z ) ) )
10		mulcompig 7415	. . . . . . . . 9 |- ( ( z e. N. /\ w e. N. ) -> ( z .N w ) = ( w .N z ) )
11	10	breq1d 4044	. . . . . . . 8 |- ( ( z e. N. /\ w e. N. ) -> ( ( z .N w ) <N ( w .N z ) <-> ( w .N z ) <N ( w .N z ) ) )
12	9, 11	bitrd 188	. . . . . . 7 |- ( ( z e. N. /\ w e. N. ) -> ( [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q <-> ( w .N z ) <N ( w .N z ) ) )
13	7, 12	mtbiri 676	. . . . . 6 |- ( ( z e. N. /\ w e. N. ) -> -. [ <. z , w >. ] ~Q <Q [ <. z , w >. ] ~Q )
14	1, 4, 13	ecoptocl 6690	. . . . 5 |- ( x e. Q. -> -. x <Q x )
15	14	adantl 277	. . . 4 |- ( ( T. /\ x e. Q. ) -> -. x <Q x )
16		breq1 4037	. . . . . . . 8 |- ( [ <. a , b >. ] ~Q = x -> ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q <-> x <Q [ <. c , d >. ] ~Q ) )
17	16	anbi1d 465	. . . . . . 7 |- ( [ <. a , b >. ] ~Q = x -> ( ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) <-> ( x <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) )
18		breq1 4037	. . . . . . 7 |- ( [ <. a , b >. ] ~Q = x -> ( [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q <-> x <Q [ <. e , f >. ] ~Q ) )
19	17, 18	imbi12d 234	. . . . . 6 |- ( [ <. a , b >. ] ~Q = x -> ( ( ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) -> [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q ) <-> ( ( x <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) -> x <Q [ <. e , f >. ] ~Q ) ) )
20		breq2 4038	. . . . . . . 8 |- ( [ <. c , d >. ] ~Q = y -> ( x <Q [ <. c , d >. ] ~Q <-> x <Q y ) )
21		breq1 4037	. . . . . . . 8 |- ( [ <. c , d >. ] ~Q = y -> ( [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q <-> y <Q [ <. e , f >. ] ~Q ) )
22	20, 21	anbi12d 473	. . . . . . 7 |- ( [ <. c , d >. ] ~Q = y -> ( ( x <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) <-> ( x <Q y /\ y <Q [ <. e , f >. ] ~Q ) ) )
23	22	imbi1d 231	. . . . . 6 |- ( [ <. c , d >. ] ~Q = y -> ( ( ( x <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) -> x <Q [ <. e , f >. ] ~Q ) <-> ( ( x <Q y /\ y <Q [ <. e , f >. ] ~Q ) -> x <Q [ <. e , f >. ] ~Q ) ) )
24		breq2 4038	. . . . . . . 8 |- ( [ <. e , f >. ] ~Q = z -> ( y <Q [ <. e , f >. ] ~Q <-> y <Q z ) )
25	24	anbi2d 464	. . . . . . 7 |- ( [ <. e , f >. ] ~Q = z -> ( ( x <Q y /\ y <Q [ <. e , f >. ] ~Q ) <-> ( x <Q y /\ y <Q z ) ) )
26		breq2 4038	. . . . . . 7 |- ( [ <. e , f >. ] ~Q = z -> ( x <Q [ <. e , f >. ] ~Q <-> x <Q z ) )
27	25, 26	imbi12d 234	. . . . . 6 |- ( [ <. e , f >. ] ~Q = z -> ( ( ( x <Q y /\ y <Q [ <. e , f >. ] ~Q ) -> x <Q [ <. e , f >. ] ~Q ) <-> ( ( x <Q y /\ y <Q z ) -> x <Q z ) ) )
28		ordpipqqs 7458	. . . . . . . . . . . . . . . 16 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q <-> ( a .N d ) <N ( b .N c ) ) )
29	28	3adant3 1019	. . . . . . . . . . . . . . 15 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q <-> ( a .N d ) <N ( b .N c ) ) )
30		simp1l 1023	. . . . . . . . . . . . . . . . 17 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> a e. N. )
31		simp2r 1026	. . . . . . . . . . . . . . . . 17 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> d e. N. )
32		mulclpi 7412	. . . . . . . . . . . . . . . . 17 |- ( ( a e. N. /\ d e. N. ) -> ( a .N d ) e. N. )
33	30, 31, 32	syl2anc 411	. . . . . . . . . . . . . . . 16 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( a .N d ) e. N. )
34		simp1r 1024	. . . . . . . . . . . . . . . . 17 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> b e. N. )
35		simp2l 1025	. . . . . . . . . . . . . . . . 17 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> c e. N. )
36		mulclpi 7412	. . . . . . . . . . . . . . . . 17 |- ( ( b e. N. /\ c e. N. ) -> ( b .N c ) e. N. )
37	34, 35, 36	syl2anc 411	. . . . . . . . . . . . . . . 16 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( b .N c ) e. N. )
38		simp3r 1028	. . . . . . . . . . . . . . . . 17 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> f e. N. )
39		mulclpi 7412	. . . . . . . . . . . . . . . . 17 |- ( ( c e. N. /\ f e. N. ) -> ( c .N f ) e. N. )
40	35, 38, 39	syl2anc 411	. . . . . . . . . . . . . . . 16 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( c .N f ) e. N. )
41		ltmpig 7423	. . . . . . . . . . . . . . . 16 |- ( ( ( a .N d ) e. N. /\ ( b .N c ) e. N. /\ ( c .N f ) e. N. ) -> ( ( a .N d ) <N ( b .N c ) <-> ( ( c .N f ) .N ( a .N d ) ) <N ( ( c .N f ) .N ( b .N c ) ) ) )
42	33, 37, 40, 41	syl3anc 1249	. . . . . . . . . . . . . . 15 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( a .N d ) <N ( b .N c ) <-> ( ( c .N f ) .N ( a .N d ) ) <N ( ( c .N f ) .N ( b .N c ) ) ) )
43	29, 42	bitrd 188	. . . . . . . . . . . . . 14 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q <-> ( ( c .N f ) .N ( a .N d ) ) <N ( ( c .N f ) .N ( b .N c ) ) ) )
44	43	biimpa 296	. . . . . . . . . . . . 13 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q ) -> ( ( c .N f ) .N ( a .N d ) ) <N ( ( c .N f ) .N ( b .N c ) ) )
45	44	adantrr 479	. . . . . . . . . . . 12 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( c .N f ) .N ( a .N d ) ) <N ( ( c .N f ) .N ( b .N c ) ) )
46		mulcompig 7415	. . . . . . . . . . . . . 14 |- ( ( ( c .N f ) e. N. /\ ( b .N c ) e. N. ) -> ( ( c .N f ) .N ( b .N c ) ) = ( ( b .N c ) .N ( c .N f ) ) )
47	40, 37, 46	syl2anc 411	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N f ) .N ( b .N c ) ) = ( ( b .N c ) .N ( c .N f ) ) )
48	47	adantr 276	. . . . . . . . . . . 12 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( c .N f ) .N ( b .N c ) ) = ( ( b .N c ) .N ( c .N f ) ) )
49	45, 48	breqtrd 4060	. . . . . . . . . . 11 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( c .N f ) ) )
50		ordpipqqs 7458	. . . . . . . . . . . . . . 15 |- ( ( ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( c .N f ) <N ( d .N e ) ) )
51	50	3adant1 1017	. . . . . . . . . . . . . 14 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( c .N f ) <N ( d .N e ) ) )
52		simp3l 1027	. . . . . . . . . . . . . . . 16 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> e e. N. )
53		mulclpi 7412	. . . . . . . . . . . . . . . 16 |- ( ( d e. N. /\ e e. N. ) -> ( d .N e ) e. N. )
54	31, 52, 53	syl2anc 411	. . . . . . . . . . . . . . 15 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( d .N e ) e. N. )
55		ltmpig 7423	. . . . . . . . . . . . . . 15 |- ( ( ( c .N f ) e. N. /\ ( d .N e ) e. N. /\ ( b .N c ) e. N. ) -> ( ( c .N f ) <N ( d .N e ) <-> ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) )
56	40, 54, 37, 55	syl3anc 1249	. . . . . . . . . . . . . 14 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N f ) <N ( d .N e ) <-> ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) )
57	51, 56	bitrd 188	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) )
58	57	biimpa 296	. . . . . . . . . . . 12 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) -> ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) )
59	58	adantrl 478	. . . . . . . . . . 11 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) )
60	5, 6	sotri 5066	. . . . . . . . . . 11 |- ( ( ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( c .N f ) ) /\ ( ( b .N c ) .N ( c .N f ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) -> ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( d .N e ) ) )
61	49, 59, 60	syl2anc 411	. . . . . . . . . 10 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( d .N e ) ) )
62		mulcompig 7415	. . . . . . . . . . . . . . 15 |- ( ( x e. N. /\ y e. N. ) -> ( x .N y ) = ( y .N x ) )
63	62	adantl 277	. . . . . . . . . . . . . 14 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( x e. N. /\ y e. N. ) ) -> ( x .N y ) = ( y .N x ) )
64		mulasspig 7416	. . . . . . . . . . . . . . 15 |- ( ( x e. N. /\ y e. N. /\ z e. N. ) -> ( ( x .N y ) .N z ) = ( x .N ( y .N z ) ) )
65	64	adantl 277	. . . . . . . . . . . . . 14 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( x e. N. /\ y e. N. /\ z e. N. ) ) -> ( ( x .N y ) .N z ) = ( x .N ( y .N z ) ) )
66		mulclpi 7412	. . . . . . . . . . . . . . 15 |- ( ( x e. N. /\ y e. N. ) -> ( x .N y ) e. N. )
67	66	adantl 277	. . . . . . . . . . . . . 14 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( x e. N. /\ y e. N. ) ) -> ( x .N y ) e. N. )
68	35, 31, 30, 63, 65, 38, 67	caov411d 6113	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N d ) .N ( a .N f ) ) = ( ( a .N d ) .N ( c .N f ) ) )
69	63, 33, 40	caovcomd 6084	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( a .N d ) .N ( c .N f ) ) = ( ( c .N f ) .N ( a .N d ) ) )
70	68, 69	eqtrd 2229	. . . . . . . . . . . 12 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N d ) .N ( a .N f ) ) = ( ( c .N f ) .N ( a .N d ) ) )
71	35, 31, 34, 63, 65, 52, 67	caov4d 6112	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N d ) .N ( b .N e ) ) = ( ( c .N b ) .N ( d .N e ) ) )
72	63, 35, 34	caovcomd 6084	. . . . . . . . . . . . . 14 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( c .N b ) = ( b .N c ) )
73	72	oveq1d 5940	. . . . . . . . . . . . 13 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N b ) .N ( d .N e ) ) = ( ( b .N c ) .N ( d .N e ) ) )
74	71, 73	eqtrd 2229	. . . . . . . . . . . 12 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( c .N d ) .N ( b .N e ) ) = ( ( b .N c ) .N ( d .N e ) ) )
75	70, 74	breq12d 4047	. . . . . . . . . . 11 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) <-> ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) )
76	75	adantr 276	. . . . . . . . . 10 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) <-> ( ( c .N f ) .N ( a .N d ) ) <N ( ( b .N c ) .N ( d .N e ) ) ) )
77	61, 76	mpbird 167	. . . . . . . . 9 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) )
78		mulclpi 7412	. . . . . . . . . . . 12 |- ( ( a e. N. /\ f e. N. ) -> ( a .N f ) e. N. )
79	30, 38, 78	syl2anc 411	. . . . . . . . . . 11 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( a .N f ) e. N. )
80		mulclpi 7412	. . . . . . . . . . . 12 |- ( ( b e. N. /\ e e. N. ) -> ( b .N e ) e. N. )
81	34, 52, 80	syl2anc 411	. . . . . . . . . . 11 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( b .N e ) e. N. )
82		mulclpi 7412	. . . . . . . . . . . 12 |- ( ( c e. N. /\ d e. N. ) -> ( c .N d ) e. N. )
83	35, 31, 82	syl2anc 411	. . . . . . . . . . 11 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( c .N d ) e. N. )
84		ltmpig 7423	. . . . . . . . . . 11 |- ( ( ( a .N f ) e. N. /\ ( b .N e ) e. N. /\ ( c .N d ) e. N. ) -> ( ( a .N f ) <N ( b .N e ) <-> ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) ) )
85	79, 81, 83, 84	syl3anc 1249	. . . . . . . . . 10 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( a .N f ) <N ( b .N e ) <-> ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) ) )
86	85	adantr 276	. . . . . . . . 9 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( ( a .N f ) <N ( b .N e ) <-> ( ( c .N d ) .N ( a .N f ) ) <N ( ( c .N d ) .N ( b .N e ) ) ) )
87	77, 86	mpbird 167	. . . . . . . 8 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( a .N f ) <N ( b .N e ) )
88		ordpipqqs 7458	. . . . . . . . . 10 |- ( ( ( a e. N. /\ b e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( a .N f ) <N ( b .N e ) ) )
89	88	3adant2 1018	. . . . . . . . 9 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( a .N f ) <N ( b .N e ) ) )
90	89	adantr 276	. . . . . . . 8 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> ( [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q <-> ( a .N f ) <N ( b .N e ) ) )
91	87, 90	mpbird 167	. . . . . . 7 |- ( ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) /\ ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) ) -> [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q )
92	91	ex 115	. . . . . 6 |- ( ( ( a e. N. /\ b e. N. ) /\ ( c e. N. /\ d e. N. ) /\ ( e e. N. /\ f e. N. ) ) -> ( ( [ <. a , b >. ] ~Q <Q [ <. c , d >. ] ~Q /\ [ <. c , d >. ] ~Q <Q [ <. e , f >. ] ~Q ) -> [ <. a , b >. ] ~Q <Q [ <. e , f >. ] ~Q ) )
93	1, 19, 23, 27, 92	3ecoptocl 6692	. . . . 5 |- ( ( x e. Q. /\ y e. Q. /\ z e. Q. ) -> ( ( x <Q y /\ y <Q z ) -> x <Q z ) )
94	93	adantl 277	. . . 4 |- ( ( T. /\ ( x e. Q. /\ y e. Q. /\ z e. Q. ) ) -> ( ( x <Q y /\ y <Q z ) -> x <Q z ) )
95	15, 94	ispod 4340	. . 3 |- ( T. -> <Q Po Q. )
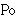96		nqtri3or 7480	. . . 4 |- ( ( x e. Q. /\ y e. Q. ) -> ( x <Q y \/ x = y \/ y <Q x ) )
97	96	adantl 277	. . 3 |- ( ( T. /\ ( x e. Q. /\ y e. Q. ) ) -> ( x <Q y \/ x = y \/ y <Q x ) )
98	95, 97	issod 4355	. 2 |- ( T. -> <Q Or Q. )
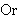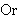99	98	mptru 1373	1 |- <Q Or Q.

Syntax hints:   -. wn 3   -> wi 4   /\ wa 104   <-> wb 105   \/ w3o 979   /\ w3a 980   = wceq 1364   T. wtru 1365   e. wcel 2167   <.cop 3626   class class class wbr 4034   Or wor 4331  (class class class)co 5925   [cec 6599   N.cnpi 7356   .N cmi 7358   <N clti 7359   ~Q ceq 7363   Q.cnq 7364   <Q cltq 7369

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-ia1 106  ax-ia2 107  ax-ia3 108  ax-in1 615  ax-in2 616  ax-io 710  ax-5 1461  ax-7 1462  ax-gen 1463  ax-ie1 1507  ax-ie2 1508  ax-8 1518  ax-10 1519  ax-11 1520  ax-i12 1521  ax-bndl 1523  ax-4 1524  ax-17 1540  ax-i9 1544  ax-ial 1548  ax-i5r 1549  ax-13 2169  ax-14 2170  ax-ext 2178  ax-coll 4149  ax-sep 4152  ax-nul 4160  ax-pow 4208  ax-pr 4243  ax-un 4469  ax-setind 4574  ax-iinf 4625

This theorem depends on definitions:  df-bi 117  df-dc 836  df-3or 981  df-3an 982  df-tru 1367  df-fal 1370  df-nf 1475  df-sb 1777  df-eu 2048  df-mo 2049  df-clab 2183  df-cleq 2189  df-clel 2192  df-nfc 2328  df-ne 2368  df-ral 2480  df-rex 2481  df-reu 2482  df-rab 2484  df-v 2765  df-sbc 2990  df-csb 3085  df-dif 3159  df-un 3161  df-in 3163  df-ss 3170  df-nul 3452  df-pw 3608  df-sn 3629  df-pr 3630  df-op 3632  df-uni 3841  df-int 3876  df-iun 3919  df-br 4035  df-opab 4096  df-mpt 4097  df-tr 4133  df-eprel 4325  df-id 4329  df-po 4332  df-iso 4333  df-iord 4402  df-on 4404  df-suc 4407  df-iom 4628  df-xp 4670  df-rel 4671  df-cnv 4672  df-co 4673  df-dm 4674  df-rn 4675  df-res 4676  df-ima 4677  df-iota 5220  df-fun 5261  df-fn 5262  df-f 5263  df-f1 5264  df-fo 5265  df-f1o 5266  df-fv 5267  df-ov 5928  df-oprab 5929  df-mpo 5930  df-1st 6207  df-2nd 6208  df-recs 6372  df-irdg 6437  df-oadd 6487  df-omul 6488  df-er 6601  df-ec 6603  df-qs 6607  df-ni 7388  df-mi 7390  df-lti 7391  df-enq 7431  df-nqqs 7432  df-ltnqqs 7437

This theorem is referenced by:  nqtric  7483  lt2addnq  7488  lt2mulnq  7489  ltbtwnnqq  7499  prarloclemarch2  7503  genplt2i  7594  genpdisj  7607  addlocprlemgt  7618  nqprdisj  7628  nqprloc  7629  addnqprlemfl  7643  addnqprlemfu  7644  prmuloclemcalc  7649  mulnqprlemfl  7659  mulnqprlemfu  7660  distrlem4prl  7668  distrlem4pru  7669  ltsopr  7680  ltexprlemopl  7685  ltexprlemopu  7687  ltexprlemdisj  7690  ltexprlemru  7696  recexprlemlol  7710  recexprlemupu  7712  recexprlemdisj  7714  recexprlemss1l  7719  recexprlemss1u  7720  cauappcvgprlemopl  7730  cauappcvgprlemlol  7731  cauappcvgprlemupu  7733  cauappcvgprlemdisj  7735  cauappcvgprlemloc  7736  cauappcvgprlemladdfu  7738  cauappcvgprlemladdru  7740  cauappcvgprlemladdrl  7741  caucvgprlemk  7749  caucvgprlemnkj  7750  caucvgprlemnbj  7751  caucvgprlemm  7752  caucvgprlemopl  7753  caucvgprlemlol  7754  caucvgprlemupu  7756  caucvgprlemloc  7759  caucvgprlemladdfu  7761  caucvgprprlemloccalc  7768  caucvgprprlemml  7778  caucvgprprlemopl  7781  suplocexprlemru  7803